how to reference a research article apa

APA Style 7th Edition: Citing Your Sources

• Basics of APA Formatting
• In Text Quick View
• Block Quotes
• Books & eBooks

Standard Format

Formatting rules, various examples.

• Thesis/Dissertation
• Audiovisual
• Conference Presentations
• Social Media
• Legal References
• Reports and Gray Literature
• Academic Integrity and Plagiarism
• Additional Resources
• Reference Page

Adapted from American Psychological Association. (2020). Publication manual of the American Psychological Association (7th ed).  https://doi.org/10.1037/0000165-000

The following formats apply to all journals, periodicals, magazines, newspapers, etc. whether you found them in an online database, search engine, or in print.

• Volume, issue and page numbers in periodicals:  Continuous pagination throughout a volume: only cite the volume number (in italics), followed by a comma and then the page numbers: 20 , 344-367.
•   Do Not copy and paste URLS from library databases or other platforms that restrict public access.

See Ch. 10 pp. 313-352 of APA Manual for more examples and formatting rules

• << Previous: Books & eBooks
• Next: Thesis/Dissertation >>
• Last Updated: Nov 1, 2023 3:17 PM
• URL: https://libguides.usc.edu/APA7th

• Franklin University |
• Help & Support |
• Locations & Maps |

• | Research Guides

To access Safari eBooks,

• Select not listed in the Select Your Institution drop down menu.
• Enter your Franklin email address and click Go
• click "Already a user? Click here" link
• Enter your Franklin email and the password you used to create your Safari account.

Continue Close

APA Citation Style 7th Edition

• APA Style Overview
• Sample Documents & Guides
• Multiple Sources With the Same Author and Year

Basic In-Text Citation Styles (7th Edition)

Journal articles (7th edition), magazine articles (7th editions), newspaper articles (7th edition), dois and urls (7th edition).

• Websites & Web Documents
• Course Materials (Slides, Lecture Notes, Specialty Software)
• Citing Business Databases
• Film, Videos, & Podcasts
• Art, Photos, Tables & Figures
• Legal Materials & Tax Codes
• Dissertations
• Pamphlet or Brochure
• Interviews, E-mail, Intranet, Religious Works, & Secondary Sources (7th edition)
• Footnotes This link opens in a new window
• Formatting an Appendix
• Evaluating Sources This link opens in a new window
• Understanding Plagiarism
• RefWorks This link opens in a new window

Basic In-Text Citation Styles

• Citation Template & Tips
• Two Authors
• Three or More Authors
• Unknown Author
• If a journal article has a DOI, include the DOI in the reference.
• If the journal article does not have a DOI and is from an academic research database, end the reference after the page range (for an explanation of why, see the  database information  page). The reference in this case is the same as for a print journal article.
• Do not include database information in the reference unless the journal article comes from a database that publishes original, proprietary content, such as UpToDate (see an example on the official APA Style  database information  page).
• If the journal article does not have a DOI but does have a URL that will resolve for readers (e.g., it is from an online journal that is not part of a database), include the URL of the article at the end of the reference.

Reference List

• Claverie, E. (2019). Folklore, fakelore, scholars, and shills: Superheroes as “Myth”.  The Journal of Popular Culture,  52 (5), 976-998. https://doi.org/10.1111/JPCU.12840

Print Version or Without a DOI from Research Database:

• Claverie, E. (2019). Folklore, fakelore, scholars, and shills: Superheroes as “Myth”.  The Journal of Popular Culture,  52 (5), 976-998.

In-Text Citation

Parenthetical citation:

• Paraphrase: (Claverie, 2019)
• Quotation: (Claverie, 2019, p. 977)

Narrative citation: Claverie (2019)

Reference List:

With DOI: 

• Zeilani, R., & Seymour, J. E. (2012). Muslim women's narrative  about bodily change and care during critical illness: A  qualitative study.  Journal Of Nursing Scholarship ,  44 (1),  99-107. https://doi.org/ 10.1111/j.1547-5069.2011.01427.x

Print Version or Without a DOI from a Research Database:

• Zeilani, R., & Seymour, J. E. (2012). Muslim women's narratives about  bodily change and care during critical illness: A qualitative study.  Journal Of Nursing Scholarship ,  44 (1), 99-107.

Parenthetical citation:  

• Paraphrase: (Zeilani & Seymour, 2012)
• Quotation:  ( Zeilani  & Seymour,  2012 , p. 105)

Narrative citation:  Zeilani & Seymour, (2012)

• Westhues, A., Lafrance, J., & Schmidt, G. (2001). A SWOT analysis of social work  education in Canada.  Social Work Education, 20 (1), 35-56. https://doi.org/ 10.1080/02615470020028364
• Westhues, A., Lafrance, J., & Schmidt, G. (2001). A SWOT analysis of social work  education in   Canada.  Social Work Education, 20 (1), 35-56.

Tips for Multiple Authors

Provide surnames and initials for up to and including 20 authors. When there are two to 20 authors, use an ampersand before the final author's name.

• Example: Author, A. A., Author, B. B., & Author, C. C.

When there are 21 or more authors, include the first 19 authors' names, insert an ellipsis (but not ampersand), and then add the final author's name.

• Example:  Author, A. A., Author, B. B., Author, C. C., Author, D. D., Author, E. E., Author, F. F., Author, G. G., Author, H. H., Author, I. I., Author, J. J., Author, K. K., Author, L. L., Author, M. M., Author, N. N., Author, O. O., Author, P. P., Author, Q. Q., Author, R. R., Author, S. S., . . .  Author, Z. Z.

NOTE:  When a work has three or more authors, include the name of only the first author plus "et al." in every citation,  including the first citation, unless doing so would create ambiguity. For more information, see Sections 8.17 & 8.18 of the APA Style Manual (7th Edition).

• Paraphrase: (Westhues et al., 2001)
• Quotation:   ​(Westhues et al., 2001, p. 40)

Narrative citation:  Westhues et al. (2001)

A work is treated as having no author when its author is unknown or cannot reasonably be determined. In this case, move the title of the work to the author position (followed by a period), before the date of publication.

Generalized anxiety disorder. (2019).

In-Text Citations

• For works with an unknown author, include the title and year of publication in the in-text citation.
• Italicize the title in the in-text citation if the title of the work is italicized in the reference list.
• If the title of the work is not italicized in the reference list, use double quotation marks around the title.
• Capitalize these titles in the text using title case, even though sentence case is used in the reference list entry.
• If the title is long, shorten for in-text citation.

Book with no author: ( Interpersonal Skills , 2019)

Magazine article with no author: ("Understanding Sensory Memory," 2018)

Note : In the rare case the "Anonymous" is used for the author, treat it as the author's name (Anonymous, 2001). In the reference list, use the name Anonymous as the author.

Magazine Article with DOI:

Schaefer, N. K., & Shapiro, B. (2019, September 6). New middle chapter in the story of human evolution.  Science ,  365 (6457), 981–982.  https://doi.org/10.1126/science.aay3550

From an Online Magazine:

Schulman, M. (2019, September 9). Superfans: A love story.  The New Yorker .  https://www.newyorker.com/magazine/2019/09/16/superfans-a-love-story

• Paraphrase: (Schaefer & Shapiro, 2019; Schulman, 2019)
• Quotation: ( Schaefer and Shapiro , 2019, p. 981)
• Narrative citations:  Schaefer and Shapiro (2019) and Schulman (2019)

Print Newspaper Article

Wallace, K. (2007, December 4). Passport applicant finds massive privacy breach.

          The  Globe and Mail , pp. A1, A8.

Online Newspaper Article

Severson, K., & Martin, A. (2009, March 3). It's organic, but does that mean it's safer?  The New York Times .

   Retrieved from https://www.nytimes.com/2009/03/04/dining/04cert.html

Print Newspaper Article

Parenthetical citations:

• Paraphrase:  (Wallace, 2007) ​​
• Quotation:  (Wallace, 2007, p. A8)

Narrative citations:  Wallace (2007)

• Paraphrase:  (Severson & Martin, 2009)
• Quotation:  (Severson & Martin, 2009)

Narrative citations: Severson (2009)

The DOI or URL is the final component of a reference list entry. Because so much scholarship is available and/or retrieved online, most reference list entries end with either a DOI or a URL.

• A  DOI  is a unique alphanumeric string that identifies content and provides a persistent link to its location on the internet. DOIs can be found in database records and the reference lists of published works.
• A  URL  specifies the location of digital information on the internet and can be found in the address bar of your internet browser. URLs in references should link directly to the cited work when possible.

When to Include DOIs and URLs

Follow these guidelines for including DOIs and URLs in references:

• Include a DOI for all works that have a DOI, regardless of whether you used the online version or the print version.
• If a print work does not have a DOI, do not include any DOI or URL in the reference.
• If an online work has both a DOI and a URL, include only the DOI.
• For works without DOIs from websites (not including academic research databases), provide a URL in the reference (as long as the URL will work for readers).
• For works without DOIs from most  academic research databases , do not include a URL or database information in the reference because these works are widely available. The reference should be the same as the reference for a print version of the work.
• Follow the current recommendations of the International DOI Foundation to format DOIs in the reference list, which as of the current  APA Style Manual  is as follows:

https://doi.org/xxxxx

• The string “https://doi.org/” is a way of presenting a DOI as a link, and “xxxxx” refers to the DOI number.
• The preferred format of the DOI has changed over time. Although older works use previous formats (e.g., “http:/dx.doi.org/” or “doi:” or “DOI:” before the DOI number), in your reference list, standardize DOIs into the current preferred format for all entries. For example, use  https://doi.org/10.1037/a0040251  in your reference even though that article, published in 2016, presented the number in an older format.
• Copy and paste the DOI or URL from your web browser directly into your reference list to avoid transcription errors. Do not change the capitalization or punctuation of the DOI or URL. Do not add line breaks manually to the hyperlink; it is acceptable if your word-processing program automatically adds a break or moves the hyperlink to its own line.
• Do not add a period after the DOI or URL because this may interfere with link functionality.
• Free DOI Lookup CrossRef currently provides a number of ways for you to locate a DOI.
• Resolve a DOI Type or paste a DOI name into the text box. Click Go. Your browser will take you to a Web page (URL) associated with that DOI name.
• << Previous: Multiple Sources With the Same Author and Year
• Next: Books >>
• Last Updated: Oct 25, 2023 2:14 PM
• URL: https://guides.franklin.edu/APA

• Research Guides
• Schlesinger Library on the History of Women in America
• Archival Materials
• Additional Resources
• Research Tips
• Ask a Schlesinger Librarian

Fundamentals of APA

American Psychological Association (APA) style includes  parenthetical in-text citations  and a  reference list . 

APA uses  parenthetical citations  as its form of in-text citation. Provide a parenthetical citation  before the period  directly following the information you are citing. These citations should correspond to a more detailed citation in the reference list but only need to specify a page number if directly quoting or borrowing from the source material. The essential elements for this in-text citation are the author's last name and the date for the specific publications. The last name may be omitted if the sentence states or makes clear the source material.

APA uses a  reference list ,  an alphabetized list of sources following the end of the book or paper,  for its complete list of sources referenced. This list should be titled "References" in bold and alphabetized by the first item in the citation, which, in most cases, is the author's last name. Each reference from this list must be cited in your paper and vice versa.

Basic Format

Author Last Name, Author First Initial. (Year of publication). Title . Publisher Name.

Print Articles

Author Last Name, Author First Initial, & Author Last Name, Author First Initial. (Year). Article Title. Periodical Title , volume number(issue number), pages.

Electronic Articles

Author Last Name, Author First Initial. (Year). Article Title. Periodical Title , volume number(issue number), pages. doi or static url.

Physical Images/Artwork

Artist Last Name, Artist First Initial. (Year). Artwork Title [medium]. Host Institution Name, City, State, Country. URL of institution.

Electronic Images/Artwork

Artist Last Name, Artist First Initial. (Year). Image Title [medium]. Source Title. URL of image.

In-text Citation Examples

Standard case :

"Lorem   ipsum  dolor sit  amet ,  consectetur   adipiscing   elit" (Last name, 2000, p.10).

If the author is not available , the title of the source may be used:

Lorem   ipsum  dolor sit  amet ,  consectetur   adipiscing   elit ("Source title", 2000, pp.10-11).

If multiple authors cited have the same last name , use the author's first initial along with their last name:

"Lorem   ipsum  dolor sit  amet ,  consectetur   adipiscing   elit" (E. Bronte, 1847, p.10).

Lorem   ipsum  dolor sit  amet ,  consectetur   adipiscing   elit (C. Bronte, 1847, p.10).

Full Citation Examples for the Reference List

Archival material :

Child, J. (1974).  Journal, 1974 . [Unpublished journal].  Papers of Julia Child, 1925-1993(MC 644, item 4).  Schlesinger Library on the History of Women in America, Radcliffe Institute,  https://id.lib.harvard.edu/ead/c/ sch00222c00006 /catalog .

Child, P. (1967). Julia Child at the White House [Photograph]. Schlesinger Library on the History of Women in America, Radcliffe Institute, https://id.lib.harvard.edu/images/olvwork539731/urn-3:RAD.SCHL:4510469/catalog.

Beck, S., Bertholle, L., & Child, J. (1961).  Mastering the art of French cooking.  Knopf.

Child, J. & Child, P. (1968).  The French chef cookbook . Alfred A. Knopf .

Journal article :

Muneal, M. (2011).  Studies in Popular Culture , 34(1), 152–154. www.jstor.org/stable/23416357.

Nussbaum, D. (2005). "In Julia Child's Kitchen, October 5 1998".  Gastronomica , 5(3), 29-38. doi: 10.1525/gfc.2005.5.3.29.

• APA Style website The online version of the APA Style Manual, this website offers to help with the technical elements of formatting in APA as well as writing and citing your work.
• Purdue OWL APA Guide The Purdue Online Writing Lab (OWL) is one of the most complete citation guides available online. The APA Guide explains how to format a paper in APA and breaks down citations by type with numerous examples.
• << Previous: MLA
• Next: Chicago >>
• Harvard Library
• Last Updated: Jan 19, 2024 9:31 AM
• URL: https://guides.library.harvard.edu/schlesinger/citation_guide

Harvard University Digital Accessibility Policy

Thursday, February 23: The Clark Library is closed today.

APA Style (7th Edition) Citation Guide: Journal Articles

• Introduction
• Journal Articles
• Magazine/Newspaper Articles
• Books & Ebooks
• Government & Legal Documents
• Biblical Sources
• Secondary Sources
• Films/Videos/TV Shows
• How to Cite: Other
• Additional Help

Table of Contents

Journal article from library database with doi - one author, journal article from library database with doi - multiple authors, journal article from a website - one author.

Journal Article- No DOI

Note: All citations should be double spaced and have a hanging indent in a Reference List.

A "hanging indent" means that each subsequent line after the first line of your citation should be indented by 0.5 inches.

This Microsoft support page contains instructions about how to format a hanging indent in a paper.

• APA 7th. ed. Journal Article Reference Checklist

If an item has no author, start the citation with the article title.

When an article has one to twenty authors, all authors' names are cited in the References List entry. When an article has twenty-one or more authors list the first nineteen authors followed by three spaced ellipse points (. . .) , and then the last author's name. Rules are different for in-text citations; please see the examples provided.

Cite author names in the order in which they appear on the source, not in alphabetical order (the first author is usually the person who contributed the most work to the publication).

Italicize titles of journals, magazines and newspapers. Do not italicize or use quotation marks for the titles of articles.

Capitalize only the first letter of the first word of the article title. If there is a colon in the article title, also capitalize the first letter of the first word after the colon.

If an item has no date, use the short form n.d. where you would normally put the date.

Volume and Issue Numbers

Italicize volume numbers but not issue numbers.

Retrieval Dates

Most articles will not need these in the citation. Only use them for online articles from places where content may change often, like a free website or a wiki.

Page Numbers

If an article doesn't appear on continuous pages, list all the page numbers the article is on, separated by commas. For example (4, 6, 12-14)

Library Database

Do not include the name of a database for works obtained from most academic research databases (e.g. APA PsycInfo, CINAHL) because works in these resources are widely available. Exceptions are Cochrane Database of Systematic Reviews, ERIC, ProQuest Dissertations, and UpToDate.

Include the DOI (formatted as a URL: https://doi.org/...) if it is available. If you do not have a DOI, include a URL if the full text of the article is available online (not as part of a library database). If the full text is from a library database, do not include a DOI, URL, or database name.

In the Body of a Paper

Books, Journals, Reports, Webpages, etc.: When you refer to titles of a “stand-alone work,” as the APA calls them on their APA Style website, such as books, journals, reports, and webpages, you should italicize them. Capitalize words as you would for an article title in a reference, e.g., In the book Crying in H Mart: A memoir , author Michelle Zauner (2021) describes her biracial origin and its impact on her identity.

Article or Chapter: When you refer to the title of a part of a work, such as an article or a chapter, put quotation marks around the title and capitalize it as you would for a journal title in a reference, e.g., In the chapter “Where’s the Wine,” Zauner (2021) describes how she decided to become a musician.

The APA Sample Paper below has more information about formatting your paper.

• APA 7th ed. Sample Paper

Author's Last Name, First Initial. Second Initial if Given. (Year of Publication). Title of article: Subtitle if any. Name of Journal, Volume Number (Issue Number), first page number-last page number. https://doi.org/doi number

Smith, K. F. (2022). The public and private dialogue about the American family on television: A second look. Journal of Media Communication, 50 (4), 79-110. https://doi.org/10.1152/j.1460-2466.2000.tb02864.x

Note: The DOI number is formatted as a URL: https://doi.org/10.1152/j.1460-2466.2000.tb02864.xIf. 

In-Text Paraphrase:

(Author's Last Name, Year)

Example: (Smith, 2000)

In-Text Quote:

(Author's Last Name, Year, p. Page Number)

Example: (Smith, 2000, p. 80)

Author's Last Name, First Initial. Second Initial if Given., & Last Name of Second Author, First Initial. Second Initial if Given. (Year of Publication). Title of article: Subtitle if any. Name of Journal, Volume Number (Issue Number), first page number-last page number. https://doi.org/doi number

Note: Separate the authors' names by putting a comma between them. For the final author listed add an ampersand (&) after the comma and before the final author's last name.

Note: In the reference list invert all authors' names; give last names and initials for only up to and including 20 authors. When a source has 21 or more authors, include the first 19 authors’ names, then three ellipses (…), and add the last author’s name. Don't include an ampersand (&) between the ellipsis and final author.

Note : For works with three or more authors, the first in-text citation is shortened to include the first author's surname followed by "et al."

Reference List Examples

Two to 20 Authors

Case, T. A., Daristotle, Y. A., Hayek, S. L., Smith, R. R., & Raash, L. I. (2011). College students' social networking experiences on Facebook. Journal of Applied Developmental Psychology, 3 (2), 227-238. https://doi.org/10.1016/j.appdev.2008.12.010

21 or more authors

Kalnay, E., Kanamitsu, M., Kistler, R., Collins, W., Deaven, D., Gandin, L., Iredell, M., Saha, J., Mo, K. C., Ropelewski, C., Wang, J., Leetma, A., . . . Joseph, D. (1996). The NCEP/NCAR 40-year reanalysis project. Bulletin of the American Meteorological Society , 77 (3), 437-471. https://doi.org/10.1175/1520-0477(1996)077<0437:TNYRP>2.0.CO;2

In-Text Citations

Two Authors/Editors

(Case & Daristotle, 2011)

Direct Quote: (Case & Daristotle, 2011, p. 57)

Three or more Authors/Editors

(Case et al., 2011)

Direct Quote: (Case et al., 2011, p. 57)

Author's Last Name, First Initial. Second Initial if Given. (Year of Publication). Title of article: Subtitle if any.  Name of Journal, Volume Number (Issue Number if given). URL

Flachs, A. (2010). Food for thought: The social impact of community gardens in the Greater Cleveland Area.  Electronic Green Journal, 1 (30). http://escholarship.org/uc/item/6bh7j4z4

Example: (Flachs, 2010)

Example: (Flachs, 2010, Conclusion section, para. 3)

Note: In this example there were no visible page numbers or paragraph numbers, in this case you can cite the section heading and the number of the paragraph in that section to identify where your quote came from. If there are no page or paragraph numbers and no marked section, leave this information out.

Journal Article - No DOI

Author's Last Name, First Initial. Second Initial if Given. (Year of Publication). Title of article: Subtitle if any.  Name of Journal, Volume Number (Issue Number), first page number-last page number. URL [if article is available online, not as part of a library database]

Full-Text Available Online (Not as Part of a Library Database):

Steinberg, M. P., & Lacoe, J. (2017). What do we know about school discipline reform? Assessing the alternatives to suspensions and expulsions.  Education Next, 17 (1), 44–52.  https://www.educationnext.org/what-do-we-know-about-school-discipline-reform-suspensions-expulsions/

Example: (Steinberg & Lacoe, 2017)

(Author's Last Name, Year, p. Page number)

Example: (Steinberg & Lacoe, 2017, p. 47)

Full-Text Available in Library Database:

Jungers, W. L. (2010). Biomechanics: Barefoot running strikes back.  Nature, 463 (2), 433-434.

Example: (Jungers, 2010)

Example: (Jungers, 2010, p. 433)

• << Previous: How to Cite: Common Sources
• Next: Magazine/Newspaper Articles >>
• Last Updated: Mar 11, 2024 3:40 PM
• URL: https://libguides.up.edu/apa

APA Citation Style 7th Edition: Welcome

• Advertisements
• Books & eBooks
• Book Reviews
• Class Handouts, Presentations, and Readings
• Encyclopedias & Dictionaries
• Government Documents
• Images, Charts, Graphs, Maps & Tables
• Journal Articles
• Magazine Articles
• Newspaper Articles
• Personal Communication (Interviews, Emails)
• Social Media
• Videos & DVDs
• Paraphrasing
• No Author, No Date etc.
• Sample Papers
• Annotated Bibliography

What is APA?

APA style was created by the American Psychological Association. It is a set of rules for publications, including research papers.

In APA, you must "cite" sources that you have paraphrased, quoted or otherwise used to write your research paper. Cite your sources in two places:

• In the body of your paper where you add a brief in-text citation.
• In the Reference list at the end of your paper where you give more complete information for the source.

Acknowledgement

What's new in the 7th edition of apa.

Below is a summary of the major changes in the 7th edition of the APA Publication Manual.

Essay Format:

• Font - While you still can use Times New Roman 12, you are free to use other fonts. Calibri 11, Arial 11, Lucida Sans 10, and Georgia 11 are all acceptable.
• Headers - No running headers are required for student papers.
• Tables and Figures - There is a standardized format for both tables and figures.

Style, Grammar, Usage:

• Singular "they" required in two situations: when used by a known person as their personal pronoun or when the gender of a singular person is not known.
• Use only one space after a sentence-ending period.

Citation Style:

• Developed the 'Four Elements of a Reference" (Author, Date, Title, Source) to help writers to create references for source types not explicitly examined in the APA Manual.
• Three or more authors can be abbreviated to First author, et al. on the first citation.
• Up to 20 authors are spelled out in the References List.
• Publisher location is not required for books
• Ebook platform, format, or device is not required for eBooks.  
• Library database names are generally not required
• No "doi:" prefix, simply include the doi.
• All hyperlinks retain the https://
• Links can be "live" in blue with underline or black without underlining

Commonly Used Terms

Citing : The process of acknowledging the sources of your information and ideas.

DOI (doi) : Some electronic content, such as online journal articles, is assigned a unique number called a Digital Object Identifier (DOI or doi). Items can be tracked down online using their doi.

In-Text Citation : A brief note at the point where information is used from a source to indicate where the information came from. An in-text citation should always match more detailed information that is available in the Reference List.

Paraphrasing : Taking information that you have read and putting it into your own words.

Plagiarism : Taking, using, and passing off as your own, the ideas or words of another.

Quoting : The copying of words of text originally published elsewhere. Direct quotations generally appear in quotation marks and end with a citation.

Reference : Details about one cited source.

Reference List : Contains details on ALL the sources cited in a text or essay, and supports your research and/or premise.

Retrieval Date : Used for websites where content is likely to change over time (e.g. Wikis), the retrieval date refers to the date you last visited the website.

• Next: How Do I Cite? >>
• Last Updated: Mar 13, 2024 2:21 PM
• URL: https://libguides.msubillings.edu/apa7

• PRO Courses Guides New Tech Help Pro Expert Videos About wikiHow Pro Upgrade Sign In
• EDIT Edit this Article
• EXPLORE Tech Help Pro About Us Random Article Quizzes Request a New Article Community Dashboard This Or That Game Popular Categories Arts and Entertainment Artwork Books Movies Computers and Electronics Computers Phone Skills Technology Hacks Health Men's Health Mental Health Women's Health Relationships Dating Love Relationship Issues Hobbies and Crafts Crafts Drawing Games Education & Communication Communication Skills Personal Development Studying Personal Care and Style Fashion Hair Care Personal Hygiene Youth Personal Care School Stuff Dating All Categories Arts and Entertainment Finance and Business Home and Garden Relationship Quizzes Cars & Other Vehicles Food and Entertaining Personal Care and Style Sports and Fitness Computers and Electronics Health Pets and Animals Travel Education & Communication Hobbies and Crafts Philosophy and Religion Work World Family Life Holidays and Traditions Relationships Youth
• Browse Articles
• Learn Something New
• Quizzes Hot
• This Or That Game New
• Train Your Brain
• Explore More
• Support wikiHow
• About wikiHow
• Log in / Sign up
• Education and Communications
• College University and Postgraduate
• Academic Writing
• Research Papers

How to Cite a Research Paper in APA

Last Updated: October 19, 2022 Fact Checked

This article was co-authored by wikiHow Staff . Our trained team of editors and researchers validate articles for accuracy and comprehensiveness. wikiHow's Content Management Team carefully monitors the work from our editorial staff to ensure that each article is backed by trusted research and meets our high quality standards. There are 12 references cited in this article, which can be found at the bottom of the page. This article has been fact-checked, ensuring the accuracy of any cited facts and confirming the authority of its sources. This article has been viewed 158,118 times. Learn more...

If you’re citing a research article or paper in APA style, you’ll need to use a specific citation format that varies depending on the source. Assess whether your source is an article or report published in an academic journal or book, or whether it is an unpublished research paper, such as a print-only thesis or dissertation. Either way, your in-text citations will need to include information about the author (if available) and the date when your source was published or written.

Sample Citations

Writing an In-Text Citation

• For example, you may write, “Gardener (2008) notes, ‘There are several factors to consider about lobsters’ (p. 199).”

• For example, you may write, “‘There are several factors to consider about lobsters’ (Gardner, 2008, p. 199).” Or, “The paper claims, ‘The fallen angel trope is common in religious and non-religious texts’ (Meek & Hill, 2015, p.13-14).”
• For articles with 3-5 authors, write out the names of all the authors the first time you cite the source. For example: (Hammett, Wooster, Smith, & Charles, 1928). In subsequent citations, write only the first author’s name, followed by et al.: (Hammett et al., 1928).
• If there are 6 or more authors for the paper, include the last name of the first author listed and then write "et al." to indicate that there are more than 5 authors.
• For example, you may write, "'This is a quote' (Minaj et al., 1997, p. 45)."

• For example, you may write, “‘The risk of cervical cancer in women is rising’ (American Cancer Society, 2012, p. 2).”

• For example, you may write, “‘Shakespeare may have been a woman’ (“Radical English Literature,” 2004, p. 45).” Or, “The paper notes, ‘There is a boom in Virgin Mary imagery’ (“Art History in Italy,” 2011, p. 32).”

• For example, you may write, “‘There are several factors to consider about lobsters’ (Gardner, 2008, p. 199).” Or, “The paper claims, ‘The fallen angel trope is common in religious and non-religious texts’ (“Iconography in Italian Frescos,” 2015, p.13-14).”

• For example, you may write, “‘There are several factors to consider about lobsters’ (Gardner, 2008, p. 199).” Or, “The paper claims, ‘The fallen angel trope is common in religious and non-religious texts’ (“Iconography in Italian Frescos,” 2015, p.145-146).”

• For example, you may write, “‘The effects of food deprivation are long-term’ (Mett, 2005, para. 18).”

Creating a Reference List Citation for a Published Source

• Material on websites is also considered “published,” even if it’s not peer-reviewed or associated with a formal publishing company.
• While academic dissertations or theses that are print-only are considered unpublished, these types of documents are considered published if they’re included in an online database (such as ProQuest) or incorporated into an institutional repository.

• For example, you may write, “Gardner, L. M.” Or, “Meek, P. Q., Kendrick, L. H., & Hill, R. W.”
• If there is no author, you can list the name of the organization that published the research paper. For example, you may write, “American Cancer Society” or “The Reading Room.”
• Formally published documents that don’t list an author or that have a corporate author are typically reports or white papers .

• For example, you may write, “Gardner, L. M. (2008).” Or, “American Cancer Society. (2015).”

• For example, you may write, “Gardner, L. M. (2008). Crustaceans: Research and data.” Or, “American Cancer Society. (2015). Cervical cancer rates in women ages 20-45.”

• For example, for a journal article, you may write, “Gardner, L. M. (2008). Crustaceans: Research and data. Modern Journal of Malacostracan Research, 25, 150-305.”
• For a book chapter, you could write: “Wooster, B. W. (1937). A comparative study of modern Dutch cow creamers. In T. E. Travers (Ed.), A Detailed History of Tea Serviceware (pp. 127-155). London: Wimble Press."

• For example, you may write, “Kotb, M. A., Kamal, A. M., Aldossary, N. M., & Bedewi, M. A. (2019). Effect of vitamin D replacement on depression in multiple sclerosis patients. Multiple Sclerosis and Related Disorders, 29, 111-117. Retrieved from PubMed, https://www.ncbi.nlm.nih.gov/pubmed/30708308.
• If you’re citing a paper or article that was published online but did not come from an academic journal or database, provide information about the author (if known), the date of publication (if available), and the website where you found the article. For example: “Hill, M. (n.d.). Egypt in the Ptolemaic Period. Retrieved from https://www.metmuseum.org/toah/hd/ptol/hd_ptol.htm”

Citing Unpublished Sources in Your Reference List

• Print-only dissertations or theses.
• Articles or book chapters that are in press or have been recently prepared or submitted for publication.
• Papers that have been rejected for publication or were never intended for publication (such as student research papers or unpublished conference papers).

• If the paper is currently being prepared for publication, include the author’s name, the year when the current draft was completed, and the title of the article in italics, followed by “Manuscript in preparation.” For example: Wooster, B. W. (1932). What the well-dressed man is wearing. Manuscript in preparation.
• If the paper has been submitted for publication, format the citation the same way as if it were in preparation, but instead follow the title with “Manuscript submitted for publication.” For example: Wooster, B. W. (1932). What the well-dressed man is wearing. Manuscript submitted for publication.
• If the paper has been accepted for publication but is not yet published, replace the date with “in press.” Do not italicize the paper title, but do include the title of the periodical or book in which it will be published and italicize that. For example: Wooster, B. W. (in press). What the well-dressed man is wearing. Milady’s Boudoir.

• If the paper was written for a conference but never published, your citation should look like this: Riker, W. T. (2019, March). Traditional methods for the preparation of spiny lobe-fish. Paper presented at the 325th Annual Intergalactic Culinary Conference, San Francisco, CA.
• For an unpublished paper written by a student for a class, include details about the institution where the paper was written. For example: Crusher, B. H. (2019). A typology of Cardassian skin diseases. Unpublished manuscript, Department of External Medicine, Starfleet Academy, San Francisco, CA.

• For example, you may write, “Pendlebottom, R. H. (2011). Iconography in Italian Frescos (Unpublished doctoral dissertation). New York University, New York, United States.”

Community Q&A

• If you want certain information to stand out in the research paper, then you can consider using a block quote. Thanks Helpful 0 Not Helpful 0

You Might Also Like

• ↑ https://libraryguides.vu.edu.au/apa-referencing/7JournalArticles
• ↑ https://owl.purdue.edu/owl/research_and_citation/apa_style/apa_formatting_and_style_guide/in_text_citations_author_authors.html
• ↑ https://bowvalleycollege.libguides.com/c.php?g=714519&p=5093747
• ↑ https://guides.libraries.psu.edu/apaquickguide/intext
• ↑ https://owl.purdue.edu/owl/research_and_citation/apa_style/apa_formatting_and_style_guide/in_text_citations_the_basics.html
• ↑ https://libguides.southernct.edu/c.php?g=7125&p=34582#1951239
• ↑ https://owl.purdue.edu/owl/research_and_citation/apa_style/apa_formatting_and_style_guide/reference_list_electronic_sources.html
• ↑ https://owl.purdue.edu/owl/research_and_citation/apa_style/apa_formatting_and_style_guide/reference_list_articles_in_periodicals.html
• ↑ https://owl.purdue.edu/owl/research_and_citation/apa_style/apa_formatting_and_style_guide/reference_list_books.html
• ↑ https://morlingcollege.libguides.com/apareferencing/unpublished-or-informally-published-work
• ↑ https://owl.purdue.edu/owl/research_and_citation/apa_style/apa_formatting_and_style_guide/general_apa_faqs.html
• ↑ https://owl.purdue.edu/owl/research_and_citation/apa_style/apa_formatting_and_style_guide/reference_list_other_print_sources.html

About This Article

To cite a research paper in-text in APA, name the author in the text to introduce the quote and put the publication date for the text in parentheses. At the end of your quote, put the page number in parentheses. If you don’t mention the author in your prose, include them in the citation. Start the citation, which should come at the end of the quote, by listing the author’s last name, the year of publication, and the page number. Make sure to put all of this information in parentheses. If there’s no author, use the name of the organization that published the paper or the first few words from the title. To learn how to cite published and unpublished sources in your reference list, keep reading! Did this summary help you? Yes No

• Send fan mail to authors

Did this article help you?

Featured Articles

Trending Articles

Watch Articles

• Terms of Use
• Privacy Policy
• Do Not Sell or Share My Info
• Not Selling Info

wikiHow Tech Help Pro:

Level up your tech skills and stay ahead of the curve

• Library Guides

APA 7th Referencing

• Journal Articles

APA 7th Referencing: Journal Articles

• In-text referencing
• Compiling a Reference list
• Citing tables and figures
• DOIs and Live hyperlinks
• Secondary sources
• Reports & Grey Literature
• Conference Materials
• Datasets, Software & Tests
• Social Media
• Images, tables & figures
• Sound & video
• Legislation & Cases
• Personal Communications
• Standards & Patents
• Course Notes or Course Presentations
• Generative AI
• Sample Reference List

On this page

Basic format to reference journal articles.

• Referencing journal articles: Examples

APA Referencing: journal articles from Victoria University Library on Vimeo .

Select the 'cc' on the video to turn on/off the captions.

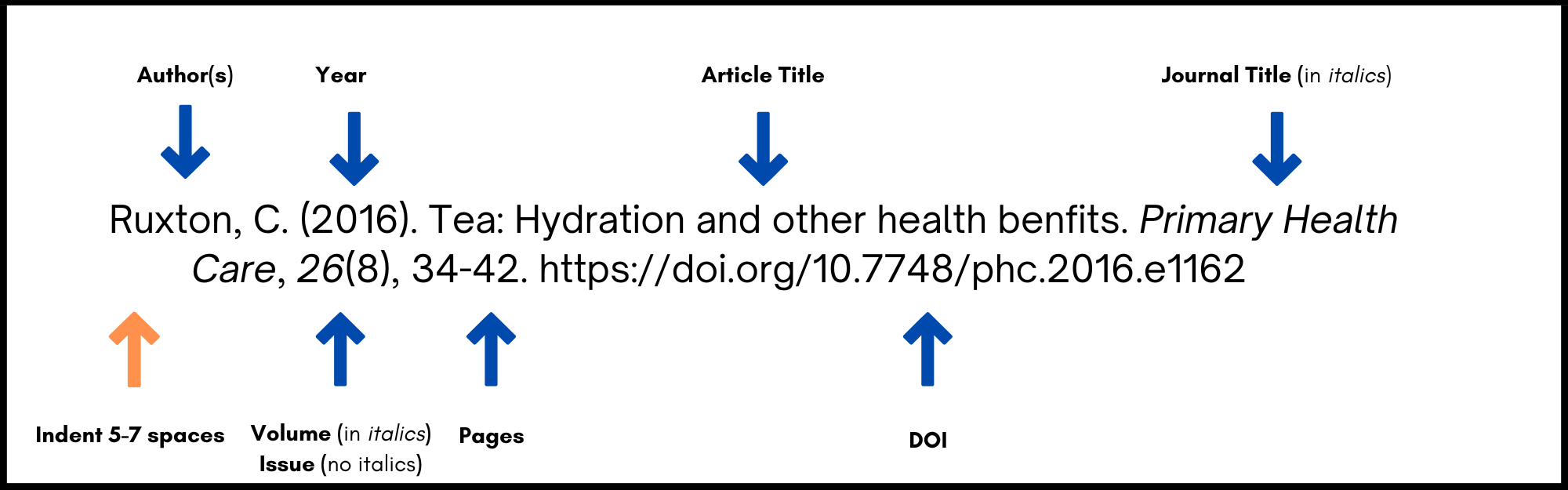
A basic reference list entry for a journal article in APA must include:

• Author or authors.  The surname is followed by first initials.
• Year of publication of the article (in round brackets).
• Article title.
• Journal title (in italics ).
• Volume of journal (in italics ).
• Issue number of journal in round brackets (no italics).
• Page range of article.
• DOI  or URL
• The first line of each citation is left adjusted. Every subsequent line is indented 5-7 spaces.

Example:  

Ruxton, C. (2016). Tea: Hydration and other health benefits. Primary Health Care , 26 (8), 34-42. https://doi.org/10.7748/phc.2016.e1162

• << Previous: APA 7th Reference Formats and Examples
• Next: Books >>
• Last Updated: Jan 18, 2024 2:09 PM
• URL: https://libraryguides.vu.edu.au/apa-referencing

• Bipolar Disorder
• Therapy Center
• When To See a Therapist
• Types of Therapy
• Best Online Therapy
• Best Couples Therapy
• Best Family Therapy
• Managing Stress
• Sleep and Dreaming
• Understanding Emotions
• Self-Improvement
• Healthy Relationships
• Student Resources
• Personality Types
• Verywell Mind Insights
• 2023 Verywell Mind 25
• Mental Health in the Classroom
• Editorial Process
• Meet Our Review Board
• Crisis Support

How to Reference Articles in APA Format

Kendra Cherry, MS, is a psychosocial rehabilitation specialist, psychology educator, and author of the "Everything Psychology Book."

Emily is a board-certified science editor who has worked with top digital publishing brands like Voices for Biodiversity, Study.com, GoodTherapy, Vox, and Verywell.

If you write a psychology paper, you will need to reference several sources in APA format. Journal articles are often used and cited to summarize the results of studies and experiments conducted by researchers. In most cases, you will need to create references for at least five or more journal articles for every APA format paper you write.

APA format details a set of strict rules for referencing articles that appear in academic journals and other periodicals. These vary somewhat based on where the article appears and who the authors are. While most articles you will use in your paper appear in academic and professional journals, you may also find articles in magazines, newspapers, and online publications.

Keep reading for a breakdown of the rules for creating references in APA format.

Basic Structure for Journal Article References

Begin the reference with the author's last name and first initials, followed by the date of publication in parentheses. Provide the title of the article, but only capitalize the first letter of the title. Next, include the journal or periodical and volume number in italics, followed by the issue number in parentheses. Finally, provide the page numbers where the article can be found.

Author, I. N. (Year). Title of the article. Title of the Journal or Periodical, volume number (issue number), page numbers.

Smith, L. V. (2000). Referencing articles in APA format. APA Format Weekly, 34 (1), 4-10.

If possible, include the DOI (digital object identifier) number at the end of your reference. If a DOI number is not available and you accessed the article online, give the URL of the journal's home page.

Formatting Rules

• The title , subtitle and all proper nouns should be capitalized.
• Your reference page should be double-spaced.
• The first line of each reference should be flush left and remaining lines should be indented

Be sure to check your references using the official Publication Manual of the American Psychological Association. See an example of different types of references and learn more about APA format .

Magazine Articles

The structure of a reference for an article appearing in a magazine is similar to that of a journal article. However, be sure to add the month and day of publication to the publication date.

James, S. A. (2001, June 7). Magazine articles in APA format. Newsweek, 20, 48-52.

Newspaper Articles

References for newspaper articles follow the basic structure as magazines, but you should list each individual page the article appears on rather than a page range.

Tensky, J. A. (2004, January 5). How to cite newspaper articles. The New York Times, 4D, 5D.

Articles With Two Authors

If an article has two authors, follow the basic format for a journal reference. Place a comma after the first initial of the first author followed by an ampersand (&). Then, include the last name and first initial of the second author.

Mischel, W., & Baker, N. (1975). Cognitive transformations of reward objects through instructions.  Journal of Personality and Social Psychology, 31 , 254-261.

Articles With Three to Twenty Authors

 For journal articles with three to 20 authors, you will follow a similar format as with two authors but each author and their initials will be separated with a comma. The final author should be preceded by an ampersand. Follow this same format for each additional author up to 20 authors.

Hart, D., Keller, M., Edelstein, W., & Hofmann, V. (1998). Childhood personality influences on social-cognitive development: A longitudinal study. Journal of Personality and Social Psychology, 74, 1288-1289. doi:10.1037/0022-3514.74.5.1278

Keller, J. L., Smithfield, K. B., Ellis, M., Michelina, R., & Bels, S. (1987). The limitations of anchoring bias. J ournal of Market Research, 17 , 115-119.

Articles With More Than Twenty Authors

The rules for referencing both single and multiple authors apply to all sources, whether the material came from books, magazine articles, newspaper articles, journal articles, or online sources. Include the last name and first initials of each author, with individuals' names separated by a comma. The last author should be preceded with an ampersand.

If the article includes 20 or fewer authors, list each author separately. If there are more than 20, include the first 19 and then include an ellipse (. . . ) in place of the authors' names before listing the final author.

Arlo, A., Black, B., Clark, C., Davidson, D., Emerson, E., Fischer, F., Grahmann, G., Habib, H., Ianelli, I., Juarez, J., Kobayashi, K., Lee, L., Martin, M., Naim, N., Odelsson, O., Pierce, P., Qiang, Q., Reed, R., Scofield, S., . . . Thatcher, T. (2011). Even more references. APA Format Today, 11 (4), 30-38.

Articles With No Author

If an article does not cite any authors, start the reference with the title of the article. Follow this with the publication date, source, and URL if the article was accessed electronically.

Scientists seek source of creativity. (2012, March, 6). Dayton County News. http://www.daytoncountynews.com/news/39756_39275.html

The reference section is one of the easiest places to lose points due to incorrect APA format, so be sure to check your references before you hand in your psychology papers . While it may be tedioous, learning to reference articles in proper APA style will help you throughout your study of psychology.

American Psychological Association. Publication Manual of the American Psychological Association  (7th ed.). The American Psychological Association, 2019.

By Kendra Cherry, MSEd Kendra Cherry, MS, is a psychosocial rehabilitation specialist, psychology educator, and author of the "Everything Psychology Book."

*Education Research Guide: APA Style

• Search for Information
• Research Tips
• Teaching Resources
• Psychological Testing

APA Style Guide

• Reference Examples: APA Style Website

Citing Personal Communications--Emails, Interviews, Lectures, etc. in APA Format

Emails, letters, memos, telephone conversations, lectures, course materials handed out in class or provided via Blackboard, and personal interviews are considered personal communications in APA. This type of communication can be difficult to provide recoverable data; therefore, these types of communication are not included in the Reference list. Cite personal communications within the body of your paper only.  Information on citing communications .  

In an interview, Sally Shoefeld explained the treatment for an accident victim (personal communication, December 18, 2023).

Note: For more information about interviews, https://apastyle.apa.org/learn/faqs/cite-interview 

Citing Government Websites

APA Citation Style does not have a separate category for government publications. According to APA, government documents can be considered Books, Technical/Research Reports, or Brochures.

Helpful Tips:

• Treat a government document as a book, report, or brochure.
• If a person is named on the title page, use her or him as author.
• If no person is named, use the government agency, department, or branch as a group author.
• Give the name of the group author exactly as it appears on the title page. If the branch or agency is not well known, include its higher department first.
• If the group author is also the publisher, just use the word Author after the location.
• If there is a series or report number, include it after the title.
• The manual refers to the GPO (U.S. Gov. Printing Office). 

General Format

In-Text Citation (Paraphrase):

(Author Surname OR Name of Government Organization, Year)

In-Text Citation (Quotation):

(Author Surname OR Name of Government Organization, Year, page number)

References:

Author Surname, First Initial. Second Initial. OR Government Name. Name of Government Agency. (Year).  Title: Subtitle  (Report No. xxx [if available]). Publisher.

(Gilmore et al., 1999)

(Gilmore et al., 1999, p. 5)

Gilmore, J., Woollam, P., Campbell, T., McLean, B., Roch, J., & Stephens, T. (1999).  Statistical report on the health of Canadians: Prepared by the Federal, Provincial and Territorial Advisory Committee on Population Health . Health Canada, Statistics Canada, Canadian Institute for Health Information.

(Edwards, et al., 1997)

(Edwards, et al., 1997, p. 2)

Edwards, N., Sims-Jones, N., Hotz, S., & Cushman, R. (1997).  Development and testing components of a multifaceted intervention program to reduce the incidence of smoking relapse during pregnancy and post-partum of both women and their partners . Report prepared for Health Canada at the Community Health Research Unit, University of Ottawa, Canada.

(Ontario Ministry of Health, 1994)

(Ontario Ministry of Health, 1994, p. 7)

Ontario Ministry of Health. (1994).  Selected findings from the mental health supplement of the Ontario Health Survey . Queen's Printer for Ontario.

(U. S. Food and Drug Administration, 2004)

(U. S. Food and Drug Administration, 2004, p. 8)

U. S. Food and Drug Administration/Center for Drug Evaluation and Research. (2004).  Worsening depression and suicidality in patients being treated with antidepressant medications: FDA public health advisory . Author.

text adapted from https://guides.himmelfarb.gwu.edu/APA/book-government-publication#:~:text=Name%20of%20Government%20Agency.,xxx%20%5Bif%20available%5D).

Citing ERIC documents

The ERIC databases adds Education document numbers to the records.  Make sure to include these and when in doubt always give your reader the trail to find the document you are referencing.

https://apastyle.apa.org/style-grammar-guidelines/references/examples/eric-database-references

Instructional Pedagogy and Curriculum Materials Librarian

• << Previous: Psychological Testing
• Last Updated: Mar 6, 2024 3:36 PM
• URL: https://guides.lib.campbell.edu/education

Purdue Online Writing Lab Purdue OWL® College of Liberal Arts

Reference List: Basic Rules

Welcome to the Purdue OWL

This page is brought to you by the OWL at Purdue University. When printing this page, you must include the entire legal notice.

Copyright ©1995-2018 by The Writing Lab & The OWL at Purdue and Purdue University. All rights reserved. This material may not be published, reproduced, broadcast, rewritten, or redistributed without permission. Use of this site constitutes acceptance of our terms and conditions of fair use.

This resourse, revised according to the 7 th  edition APA Publication Manual, offers basic guidelines for formatting the reference list at the end of a standard APA research paper. Most sources follow fairly straightforward rules. However, because sources obtained from academic journals  carry special weight in research writing, these sources are subject to special rules . Thus, this page presents basic guidelines for citing academic journals separate from its "ordinary" basic guidelines. This distinction is made clear below.

Note:  Because the information on this page pertains to virtually all citations, we've highlighted one important difference between APA 6 and APA 7 with an underlined note written in red.  For more information, please consult the   Publication Manual of the American Psychological Association , (7 th  ed.).

Formatting a Reference List

Your reference list should appear at the end of your paper. It provides the information necessary for a reader to locate and retrieve any source you cite in the body of the paper. Each source you cite in the paper must appear in your reference list; likewise, each entry in the reference list must be cited in your text.

Your references should begin on a new page separate from the text of the essay; label this page "References" in bold, centered at the top of the page (do NOT underline or use quotation marks for the title). All text should be double-spaced just like the rest of your essay.

Basic Rules for Most Sources

• All lines after the first line of each entry in your reference list should be indented one-half inch from the left margin. This is called hanging indentation.
• All authors' names should be inverted (i.e., last names should be provided first).
• For example, the reference entry for a source written by Jane Marie Smith would begin with "Smith, J. M."
• If a middle name isn't available, just initialize the author's first name: "Smith, J."
• Give the last name and first/middle initials for all authors of a particular work up to and including 20 authors ( this is a new rule, as APA 6 only required the first six authors ). Separate each author’s initials from the next author in the list with a comma. Use an ampersand (&) before the last author’s name. If there are 21 or more authors, use an ellipsis (but no ampersand) after the 19th author, and then add the final author’s name.
• Reference list entries should be alphabetized by the last name of the first author of each work.
• For multiple articles by the same author, or authors listed in the same order, list the entries in chronological order, from earliest to most recent.
• Note again that the titles of academic journals are subject to special rules. See section below.
• Italicize titles of longer works (e.g., books, edited collections, names of newspapers, and so on).
• Do not italicize, underline, or put quotes around the titles of shorter works such as chapters in books or essays in edited collections.

Basic Rules for Articles in Academic Journals

• Present journal titles in full.
• Italicize journal titles.
• For example, you should use  PhiloSOPHIA  instead of  Philosophia,  or  Past & Present   instead of  Past and Present.
• This distinction is based on the type of source being cited. Academic journal titles have all major words capitalized, while other sources' titles do not.
• Capitalize   the first word of the titles and subtitles of   journal articles , as well as the   first word after a colon or a dash in the title, and   any proper nouns .
• Do not italicize or underline the article title.
• Deep blue: The mysteries of the Marianas Trench.
• Oceanographic Study: A Peer-Reviewed Publication

Please note:  While the APA manual provides examples of how to cite common types of sources, it does not cover all conceivable sources. If you must cite a source that APA does not address, the APA suggests finding an example that is similar to your source and using that format. For more information, see page 282 of the   Publication Manual of the American Psychological Association , 7 th  ed.

• A-Z Databases
• Training Calendar
• Research Portal

Referencing: APA Style 7th Edition

• More in-text citations
• Reference list
• Books and book chapters
• DOI and URL information
• Government and other reports
• Websites and social media
• Audio, music and visual media
• Conferences
• Dictionaries/ Encyclopedias/ Guides
• Theses/ Dissertations
• University course materials
• Tables and figures
• Abbreviations

Reference list format

• Author layout guidance
• Sample reference list

General notes and tips:

• All of the references in the reference list must also be cited in the text.
• All references cited in text must also be included in the reference list. Note: unpublished items, such as personal correspondence, are an exception.
• List the references in alphabetical order by author surname according to the first listed author. (Note: The order of the authors on a document is important. Do not rearrange them).
• Where there are two articles with the same authors and date, order the references alphabetically by article title and add a letter suffix to the year of copyright, for example (Smith, 2003a, 2003b...).
• Provide organisation names in full, unless they are obviously recognisable as abbreviations (e.g., APA for American Psychological Association).
• Do not add full stops to URLs (e.g., https://lib.uwc.ac.za/)
• In an article, chapter or book title capitalise only the first word of the title and of the subtitle and any proper nouns. A proper noun is a person, place or entity. (Note: Book titles should be italicised).
• In a periodical, journal, or serial title, give the title in full, in title case. The title should be italicised (e.g., Durban Business Review).
• Formatting tip: All reference entries should be double-spaced. Quick tip: to change your text to double space: highlight the reference list then choose "CTRL 2" (PC) or "Command 2" (Mac).
• << Previous: More in-text citations
• Next: Books and book chapters >>
• Last Updated: Mar 8, 2024 3:38 PM
• URL: https://libguides.uwc.ac.za/ReferencingAPA7th

UWC LIBRARY & INFORMATION SERVICES

Have a language expert improve your writing

Run a free plagiarism check in 10 minutes, generate accurate citations for free.

• Knowledge Base
• Citing sources
• How to Cite a Journal Article | APA, MLA, & Chicago Examples

How to Cite a Journal Article | APA, MLA, & Chicago Examples

Published on March 9, 2021 by Jack Caulfield . Revised on January 17, 2024.

To cite an article from an academic journal, you need an in-text citation and a corresponding reference listing the name(s) of the author(s), the publication date, the article title and journal name, the volume and issue numbers, the page range, and the URL or DOI .

Different citation styles present this information differently. The main citation styles are APA , MLA , and Chicago style .

You can use the interactive example generator to explore the format for APA and MLA journal article citations.

Instantly correct all language mistakes in your text

Upload your document to correct all your mistakes in minutes

Table of contents

Citing an article in apa style, citing an article in mla style, citing an article in chicago style, frequently asked questions about citations.

In an APA Style journal article reference , the article title is in plain text and sentence case, while the journal name appears in italics, in title case.

The in-text citation lists up to two authors; for three or more, use “ et al. ”

When citing a journal article in print or from a database, don’t include a URL. You can still include the DOI if available.

You can also cite a journal article using our free APA Citation Generator . Search by title or DOI to automatically generate a correct citation.

Generate accurate APA citations with Scribbr

The only proofreading tool specialized in correcting academic writing - try for free.

The academic proofreading tool has been trained on 1000s of academic texts and by native English editors. Making it the most accurate and reliable proofreading tool for students.

Try for free

In an MLA Works Cited entry for a journal article , the article title appears in quotation marks, the name of the journal in italics—both in title case.

List up to two authors in both the in-text citation and the Works Cited entry. For three or more, use “et al.”

A DOI is always included when available; a URL appears if no DOI is available but the article was accessed online . If you accessed the article in print and no DOI is available, you can omit this part.

You can also use our free MLA Citation Generator to create your journal article citations.

Generate accurate MLA citations with Scribbr

In Chicago notes and bibliography style, you include a bibliography entry for each source, and cite them in the text using footnotes .

A bibliography entry for a journal article lists the title of the article in quotation marks and the journal name in italics—both in title case. List up to 10 authors in full; use “et al.” for 11 or more.

In the footnote, use “et al.” for four or more authors.

A DOI or URL (preferably a DOI) is included for articles consulted online; for articles consulted in print, omit this part.

Chicago also offers an alternative author-date style of citation. Examples of how to cite journal articles in this style can be found here .

The elements included in journal article citations across APA , MLA , and Chicago style are the name(s) of the author(s), the title of the article, the year of publication, the name of the journal, the volume and issue numbers, the page range of the article, and, when accessed online, the DOI or URL.

In MLA and Chicago style, you also include the specific month or season of publication alongside the year, when this information is available.

The DOI is usually clearly visible when you open a journal article on an academic database. It is often listed near the publication date, and includes “doi.org” or “DOI:”. If the database has a “cite this article” button, this should also produce a citation with the DOI included.

If you can’t find the DOI, you can search on Crossref using information like the author, the article title, and the journal name.

The abbreviation “ et al. ” (Latin for “and others”) is used to shorten citations of sources with multiple authors.

“Et al.” is used in APA in-text citations of sources with 3+ authors, e.g. (Smith et al., 2019). It is not used in APA reference entries .

Use “et al.” for 3+ authors in MLA in-text citations and Works Cited entries.

Use “et al.” for 4+ authors in a Chicago in-text citation , and for 10+ authors in a Chicago bibliography entry.

Check if your university or course guidelines specify which citation style to use. If the choice is left up to you, consider which style is most commonly used in your field.

• APA Style is the most popular citation style, widely used in the social and behavioral sciences.
• MLA style is the second most popular, used mainly in the humanities.
• Chicago notes and bibliography style is also popular in the humanities, especially history.
• Chicago author-date style tends to be used in the sciences.

Other more specialized styles exist for certain fields, such as Bluebook and OSCOLA for law.

The most important thing is to choose one style and use it consistently throughout your text.

Cite this Scribbr article

If you want to cite this source, you can copy and paste the citation or click the “Cite this Scribbr article” button to automatically add the citation to our free Citation Generator.

Caulfield, J. (2024, January 17). How to Cite a Journal Article | APA, MLA, & Chicago Examples. Scribbr. Retrieved March 15, 2024, from https://www.scribbr.com/citing-sources/cite-a-journal-article/

Is this article helpful?

Jack Caulfield

Other students also liked, how to cite a book | apa, mla, & chicago examples, how to cite a website | mla, apa & chicago examples, how to cite a wikipedia article | apa, mla & chicago, what is your plagiarism score.

APA Citation and Format

• APA Format, 7th Edition
• Tutorials for APA 7th ed.
• Formatting Basics
• In-Text Citations
• References List
• HU Writing Lab This link opens in a new window
• Citation Managers
• Citing and Using Images
• Avoiding Plagiarism
• Check Your Knowledge

Plagiarism Defined

• APA Plagiarism Guide
• Self-plagiarism
• AI & Plagiarism

APA considers self-plagiarism unethical, and provides the following explanation:

"Self-plagiarism is the presentation of your own previously published work as original; like plagiarism, self-plagiarism is unethical. Self-plagiarism deceives readers by making it appear that more information is available on a topic than really exists. It gives the impression that findings are more replicable than is the case or that particular conclusions are more strongly supported than is warranted by the evidence. It may lead to copyright violations if you publish the same work with multiple publishers (sometimes called duplicate publication )." -- APA Style and Grammar Guidelines

• Plagiarism - APA Style and Grammar Guidelines
• Self-Plagiarism Explained (SJSU)

Quoting or reproducing the text created by ChatGPT in your paper

If you’ve used ChatGPT or other AI tools in your research, describe how you used the tool in your Method section or in a comparable section of your paper. For literature reviews or other types of essays or response or reaction papers, you might describe how you used the tool in your introduction. In your text, provide the prompt you used and then any portion of the relevant text that was generated in response.

Unfortunately, the results of a ChatGPT “chat” are not retrievable by other readers, and although nonretrievable data or quotations in APA Style papers are usually cited as personal communications , with ChatGPT-generated text there is no person communicating. Quoting ChatGPT’s text from a chat session is therefore more like sharing an algorithm’s output; thus, credit the author of the algorithm with a reference list entry and the corresponding in-text citation.

When prompted with “Is the left brain right brain divide real or a metaphor?” the ChatGPT-generated text indicated that although the two brain hemispheres are somewhat specialized, “the notation that people can be characterized as ‘left-brained’ or ‘right-brained’ is considered to be an oversimplification and a popular myth” (OpenAI, 2023).

OpenAI. (2023). ChatGPT (Mar 14 version) [Large language model]. https://chat.openai.com/chat

You may also put the full text of long responses from ChatGPT in an appendix of your paper or in online supplemental materials, so readers have access to the exact text that was generated. It is particularly important to document the exact text created because ChatGPT will generate a unique response in each chat session, even if given the same prompt. If you create appendices or supplemental materials, remember that each should be called out at least once in the body of your APA Style paper.

When given a follow-up prompt of “What is a more accurate representation?” the ChatGPT-generated text indicated that “different brain regions work together to support various cognitive processes” and “the functional specialization of different regions can change in response to experience and environmental factors” (OpenAI, 2023; see Appendix A for the full transcript).

Creating a reference to ChatGPT or other AI models and software

The in-text citations and references above are adapted from the reference template for software in Section 10.10 of the Publication Manual (American Psychological Association, 2020, Chapter 10). Although here we focus on ChatGPT, because these guidelines are based on the software template, they can be adapted to note the use of other large language models (e.g., Bard), algorithms, and similar software.

The reference and in-text citations for ChatGPT are formatted as follows:

• Parenthetical citation: (OpenAI, 2023)
• Narrative citation: OpenAI (2023)

Let’s break that reference down and look at the four elements (author, date, title, and source):

Author: The author of the model is OpenAI.

Date: The date is the year of the version you used. Following the template in Section 10.10, you need to include only the year, not the exact date. The version number provides the specific date information a reader might need.

Title: The name of the model is “ChatGPT,” so that serves as the title and is italicized in your reference, as shown in the template. Although OpenAI labels unique iterations (i.e., ChatGPT-3, ChatGPT-4), they are using “ChatGPT” as the general name of the model, with updates identified with version numbers.

The version number is included after the title in parentheses. The format for the version number in ChatGPT references includes the date because that is how OpenAI is labeling the versions. Different large language models or software might use different version numbering; use the version number in the format the author or publisher provides, which may be a numbering system (e.g., Version 2.0) or other methods.

Bracketed text is used in references for additional descriptions when they are needed to help a reader understand what’s being cited. References for a number of common sources, such as journal articles and books, do not include bracketed descriptions, but things outside of the typical peer-reviewed system often do. In the case of a reference for ChatGPT, provide the descriptor “Large language model” in square brackets. OpenAI describes ChatGPT-4 as a “large multimodal model,” so that description may be provided instead if you are using ChatGPT-4. Later versions and software or models from other companies may need different descriptions, based on how the publishers describe the model. The goal of the bracketed text is to briefly describe the kind of model to your reader.

Source: When the publisher name and the author name are the same, do not repeat the publisher name in the source element of the reference, and move directly to the URL. This is the case for ChatGPT. The URL for ChatGPT is https://chat.openai.com/chat . For other models or products for which you may create a reference, use the URL that links as directly as possible to the source (i.e., the page where you can access the model, not the publisher’s homepage).

This information is from the APA Style Blog. For more information on this topic please refer to this page: https://apastyle.apa.org/blog/how-to-cite-chatgpt

Harrisburg University now provides access to Turnitin's Draft Coach -  a writing and grammar help tool integrated into Word Online. 

• Using Draft Coach
• Draft Coach Handout Quick guide to Turnitin Draft Coach, a grammar, writing, and citation help tool available to HU students through Word Online.

Academic Integrity and Citation Tutorial

• Academic Integrity
• Information Has Value
• Information Ethics Quiz
• Why Citations Matter
• APA Citations, 7th Ed.
• APA Citations Quiz
• Academic Integrity (Credo Plagiarism Module) Use this link to complete the Academic Integrity tutorial module. It will open in a new browser tab.

• Plagiarism Video (Credo Plagiarism Module) Use this link to complete the Plagiarism tutorial segment. It will open in a new browser tab.

• Information Has Value Tutorial (Credo Plagiarism Module)

• Information Ethics Quiz (Credo Plagiarism Module) Use this to take the Information Ethics quiz. It will open in a new browser tab.

• Why Citations Matter Video (Credo Plagiarism Module) Use this link to access the Why Citations Matter video. It will open in a new browser tab.
• Why Citations Matter Tutorial (Credo Plagiarism Module) Use this link to complete the Why Citations Matter tutorial segment. It will open in a new browser window.

• APA Citations Tutorial, 7th ed. (Credo Plagiarism Module) Use this to complete the APA 7th edition style tutorial. It will open in a new browser window.

• APA Citations Quiz (Credo Plagiarism Module) Use this link if the above content is not loading correctly or you are unable to login. It will open in a new browser window.

• << Previous: Citing and Using Images
• Next: Check Your Knowledge >>
• Last Updated: Mar 12, 2024 11:16 AM
• URL: https://library.harrisburgu.edu/apa

Login | Register

• Editorial Team

"Penang Rejects Reclamation": Pre-capitalist worldviews and post-development ideals within a Malaysian environmental movement

Author: Daniele Speziale

• View Harvard Citation Style
• View Vancouver Citation Style
• View APA Citation Style
• Download RIS
• Download BibTeX

Malaysia's indigenous and rural communities have played a vital role in the growth of the country's environmental movement. Yet, the ways in which their cultures and ancestral attachments to territory come to underpin their activism has remained understudied. This article focuses on the current dispute between state authorities in Penang Island, keen on reclaiming land for industrial development, and "Penang Tolak Tambak", a movement composed of traditional fisherfolk and environmental activists who aim at preserving the coastal ecosystem. Employing Arturo Escobar's "ecology of difference" and Erik Olin Wright's "anti-capitalist strategies" as theoretical framework, this article uncovers multiple ways in which fisherfolk's identity became a salient part of their environmental struggle. Most importantly, the spiritual principle of fardhu kifayah, by which ecosystem preservation becomes a divinely-ordained responsibility towards one another, comes to directly clash with neoliberal-capitalist development as pursued by the state. While the study illustrates how Malaysian rural communities preserve pre-capitalist human-nature relations, it also shows how fisherfolk face serious material constraints in promoting their lifestyle, their mobilization being thus limited to strategies of resistance. The conclusion encourages further research on "ecologies of difference" and how these can not only be defended but also promoted as broader, systemic alternatives.

Keywords: anti-capitalism, land reclamation, Islamic ecology, Post-Development, environmentalism

Speziale, D., (2024) “"Penang Rejects Reclamation": Pre-capitalist worldviews and post-development ideals within a Malaysian environmental movement”, Journal of Political Ecology 31(1), 158–177. doi: https://doi.org/10.2458/jpe.5476

Downloads: Download article ID 5476 new View PDF

13 Downloads

Published on 14 mar 2024, peer reviewed, creative commons attribution 4.0, harvard-style citation.

Speziale, D. (2024) '"Penang Rejects Reclamation": Pre-capitalist worldviews and post-development ideals within a Malaysian environmental movement', Journal of Political Ecology . 31(1) :158–177. doi: 10.2458/jpe.5476

Show: Vancouver Citation Style | APA Citation Style

Vancouver-Style Citation

Speziale, D. "Penang Rejects Reclamation": Pre-capitalist worldviews and post-development ideals within a Malaysian environmental movement. Journal of Political Ecology. 2024 3; 31(1) :158–177. doi: 10.2458/jpe.5476

Show: Harvard Citation Style | APA Citation Style

APA-Style Citation

Speziale, D. (2024, 3 14). "Penang Rejects Reclamation": Pre-capitalist worldviews and post-development ideals within a Malaysian environmental movement. Journal of Political Ecology 31(1) :158–177. doi: 10.2458/jpe.5476

Show: Harvard Citation Style | {% trans 'Vancouver Citation Style' %}

Non Specialist Summary

This article has no summary