hypothesis and conclusion of intermediate value theorem

• Math Article

Intermediate Value Theorem

The intermediate value theorem is a theorem about continuous functions. Intermediate value theorem has its importance in Mathematics, especially in functional analysis. This theorem explains the virtues of continuity of a function . The two important cases of this theorem are widely used in Mathematics. Let us go ahead and learn about the intermediate value theorem and its two statements in this article.

Intermediate Value Theorem Statement

Intermediate value theorem states that if “f” be a continuous function over a closed interval [a, b] with its domain having values f(a) and f(b) at the endpoints of the interval, then the function takes any value between the values f(a) and f(b) at a point inside the interval. This theorem is explained in two different ways:

Statement 1:

If k is a value between f(a) and f(b), i.e.

either f(a) < k < f(b) or f(a) > k > f(b)

then there exists at least a number c within a to b i.e. c ∈ (a, b) in such a way that f(c) = k

Statement 2:

The set of images of function in interval [a, b], containing [f(a), f(b)] or [f(b), f(a)], i.e.

Theorem Explanation:

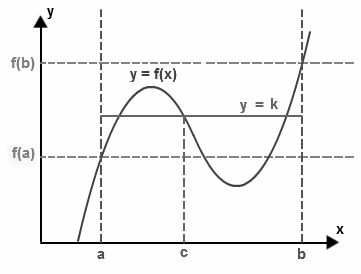
The statement of intermediate value theorem seems to be complicated. But it can be understood in simpler words. Let us consider the above diagram, there is a continuous function f with endpoints a and b, then the height of the point “a” and “b” would be “f(a)” and “f(b)”.

If we pick a height k between these heights f(a) and f(b), then according to this theorem, this line must intersect the function f at some point (say c), and this point must lie between a and b.

An intermediate value theorem, if c = 0, then it is referred to as Bolzano’s theorem .

Intermediate Theorem Proof

We are going to prove the first case of the first statement of the intermediate value theorem since the proof of the second one is similar.

We will prove this theorem by the use of completeness property of real numbers . The proof of “f(a) < k < f(b)” is given below:

Let us assume that A is the set of all the values of x in the interval [a, b], in such a way that f(x) ≤ k.

Here A is supposed to be a non-empty set as it has an element “a” and also A is bounded above by the value “b”.

Thus, by completeness property, we have that, “c” be the lowest value which is greater than or equal to each element of A. Hence, we can say that f(c) = k.

Given that f is continuous. Then let us consider a ε > 0, there exists “a δ > 0” such that

| f(x) – f(c) | < ε for every | x – c | < δ. This gives us

f(x) – ε < f(c) < f(x) + ε

For each x lying within c – δ and c + δ. So, we have values of x lying between c and c -δ, contained in A, such that :

f(c) < (f(x) + ε) ≤ (k + ε) ——– (1)

Similarly, values of x between c and c + δ that are not contained in A, such that

f(c) > (f(x) – ε) > (k − ε) ——–(2)

Combining both the inequality relations, obtain

k – ε < f(c) < k + ε

For every ε > 0

Hence, the theorem is proved.

Intermediate Theorem Applications

The intermediate value theorem has many applications. Mathematically, it is used in many areas. This theorem is utilized to prove that there exists a point below or above a given particular line. It is also used to analyze the continuity of a function that is continuous or not.

This theorem has many implications in Physics and Chemistry problems too. It is applicable whenever there is a continuously varying scalar quantity with endpoints sharing the same value for a variable. These quantities may be – pressure, temperature, elevation, carbon dioxide gas concentration, etc.

Intermediate value theorem has some significant real-life applications too. Let us take an example of a wobbly table due to the uneven ground. In order to fix this, rotate the table, provided that the ground is continuous; i.e. no ups and downs due to poorly-fitted tiles.

The wobbly table will have three of its legs touching the ground, while its fourth leg will be the problem. While rotating the table at a point, the fourth leg will be below the ground, and at some other point, it will lie above the ground. According to the intermediate value theorem, there will be a point at which the fourth leg will perfectly touch the ground, and the table is fixed.

Intermediate Theorem Example

Check whether there is a solution to the equation x 5 – 2x 3 -2 = 0 between the interval [0, 2].

Let us find the values of the given function at the x = 0 and x = 2.

f(x) = x 5 – 2x 3 -2 = 0

Substitute x = 0 in the given function

f(0) = (0) 5 – 2(0) 3 -2

Substitute x = 2 in the given function

f(2) = (2) 5 – 2(2) 3 -2

f(2) = 32 – 16 – 2

Therefore, we conclude that at x = 0, the curve is below zero; while at x = 2, it is above zero.

Since the given equation is a polynomial, its graph will be continuous.

Thus, applying the intermediate value theorem, we can say that the graph must cross at some point between (0, 2).

Hence, there exists a solution to the equation x 5 – 2x 3 -2 = 0 between the interval [0, 2].

Visit BYJU’s – The Learning App and download the app to explore all the important Maths-related videos to learn with ease.

• Share Share

Register with BYJU'S & Download Free PDFs

Register with byju's & watch live videos.

Intermediate Value Theorem

The idea behind the Intermediate Value Theorem is this:

one point below the line the other point above the line

then there is at least one place where the curve crosses the line!

Well of course we must cross the line to get from A to B!

Now that you know the idea , let's look more closely at the details.

The curve must be continuous ... no gaps or jumps in it.

Continuous is a special term with an exact definition in calculus, but here we will use this simplified definition:

More Formal

Here is the Intermediate Value Theorem stated more formally:

• The curve is the function y = f(x) ,
• which is continuous on the interval [a, b],
• and w is a number between f(a) and f(b),

... there must be at least one value c within [a, b] such that f(c) = w

In other words the function y = f(x) at some point must be w = f(c)

Notice that:

• w is between f(a) and f(b), which leads to ...
• c must be between a and b

At Least One

It also says "at least one value c", which means we could have more.

Here, for example, are 3 points where f(x)=w:

How Is This Useful?

Whenever we can show that:

• there is a point above some line
• and a point below that line, and
• that the curve is continuous,

we can then safely say "yes, there is a value somewhere in between that is on the line".

Example: is there a solution to x 5 − 2x 3 − 2 = 0 between x=0 and x=2?

0 5 − 2 × 0 3 − 2 = − 2

  At x=2:

2 5  − 2 × 2 3 − 2 = 14

Now we know:

• at x=0, the curve is below zero
• at x=2, the curve is above zero

And, being a polynomial, the curve will be continuous,

so somewhere in between the curve must cross through y=0

Yes, there is a solution to x 5 − 2x 3 − 2 = 0 in the interval [0, 2]

An Interesting Thing!

The intermediate value theorem can fix a wobbly table, why does this work.

We can always have 3 legs on the ground, it is the 4th leg that is the trouble.

Imagine we are rotating the table , and the 4th leg could somehow go into the ground (like sand):

• at some point it will be above the ground
• at another point it will be below the ground

So there must be some point where the 4th leg perfectly touches the ground and the table won't wobble.

(The famous Martin Gardner wrote about this in Scientific American. There is also a very complicated proof somewhere).

Another One

(It only works if you don't start at the highest or lowest point.)

The idea is:

• at some point you will be higher than where you started
• at another point you will be lower than where you started

So there must be a point in between where you are exactly as high as where you started.

Oh, and your path must be continuous , no disappearing and reappearing somewhere else.

The same thing happens with temperature, pressure, and so on.

And There's More!

If you follow a circular path ... somewhere on that circle there will be points that are:

• directly opposite each other
• and at the same height!

Can you think of more examples?

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons
• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

3.3: Intermediate Value Theorem, Existence of Solution

• Last updated
• Save as PDF
• Page ID 1198

While the idea of continuity may seem somewhat basic, when a function is continuous over a closed interval like x∈[1,4], you can actually draw some major conclusions. The conclusions may be obvious when you understand the statements and look at a graph, but they are powerful nonetheless.

What can you conclude using the Intermediate Value Theorem and the Extreme Value Theorem about a function that is continuous over the closed interval x∈[1,4]?

The Intermediate and Extreme Value Theorems

The Intermediate Value Theorem states that if a function is continuous on a closed interval and u is a value between f(a) and f(b) then there exists a c∈[a,b] such that f(c)=u.

CK-12 Foundation - http://commons.wikimedia.org/wiki/File:Intermediatevaluetheorem.svg - CC BY-SA

Simply stated, if a function is continuous between a low point and a high point, then it must be valued at each intermediate height in between the low and high points.

The converse of an if then statement is a new statement with the hypothesis of the original statement switched with the conclusion of the original statement. In other words, the converse is when the if part of the statement and the then part of the statement are swapped. In general, the converse of a statement is not true.

The converse of the Intermediate Value Theorem is: If there exists a value c∈[a,b] such that f(c)=u for every u between f(a) and f(b) then the function is continuous.

This statement is false. In order to show the statement is false, all you need is one counterexample where every intermediate value is hit and the function is discontinuous.A counterexample to an if then statement is when the hypothesis (the if part of the sentence) is true, but the conclusion (the then part of the statement) is not true.

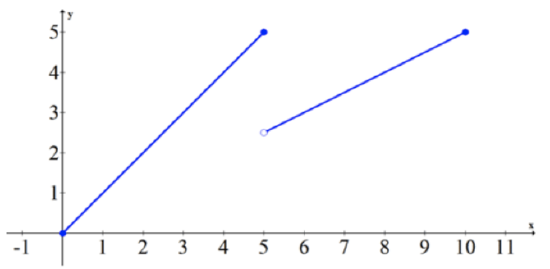
CK-12 Foundation - CC BY-SA

This function is discontinuous on the interval [0,10] but every intermediate value between the first height at (0,0) and the height of the last point (10,5) is hit.

The Extreme Value Theorem states that in every interval [a,b] where a function is continuous there is at least one maximum and one minimum. In other words, it must have at least two extreme values.

CK-12 Foundation - http://commons.wikimedia.org/wiki/File:Extreme_Value_Theorem.svg ; https://commons.wikimedia.org/wiki/File:CentralParkFromAboveCropped.jpg - CC BY-SA

The converse of the Extreme Value Theorem is : If there is at least one maximum and one minimum in the closed interval [a,b] then the function is continuous on [a,b].

This statement is false. In order to show the statement is false, all you need is one counterexample. The goal is to find a function on a closed interval [a,b] that has at least one maximum and one minimum and is also discontinuous.

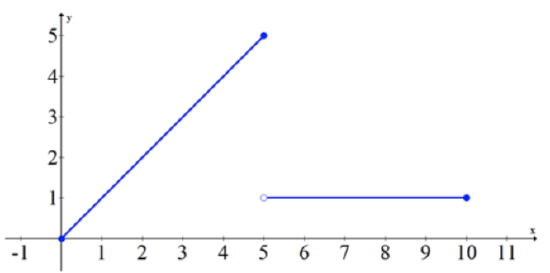
On the interval [0,10], the function attains a maximum at (5,5) and a minimum at (0,0) but is still discontinuous.

Earlier, you were asked to apply the Intermediate and Extreme Value Theorems to a function is continuous on the interval x∈[1,4]. You can conclude by the Intermediate Value Theorem that there exists a c∈[1,4] such that f(c)=u for every u between f(1) and f(4). You can also conclude that on this interval the function has both a maximum and a minimum value by the Extreme Value Theorem.

Use the Intermediate Value Theorem to show that the function f(x)=(x+1) 3 −4 has a zero on the interval [0,3].

First note that the function is a cubic and is therefore continuous everywhere.

• f(0)=(0+1) 3 −4=1 3 −4=−3
• f(3)=(3+1) 3 −4=4 3 −4=60

By the Intermediate Value Theorem, there must exist a c∈[0,3] such that f(c)=0 since 0 is between -3 and 60.

Use the Intermediate Value Theorem to show that the following equation has at least one real solution.

First rewrite the equation: x8−2x=0

Then describe it as a continuous function: f(x)=x8−2x

This function is continuous because it is the difference of two continuous functions.

• f(0)=0 8 −2 0 =0−1=−1
• f(2)=2 8 −2 2 =256−4=252

By the Intermediate Value Theorem, there must exist a c such that f(c)=0 because −1<0<252. The number c is one solution to the initial equation.

Show that there is at least one solution to the following equation.

Write the equation as a continuous function: f(x)=sinx−x−2

The function is continuous because it is the sum and difference of continuous functions.

• f(0)=sin0−0−2=−2
• f(−π)=sin(−π)+π−2=0+π−2>0

By the Intermediate Value Theorem, there must exist a c such that f(c)=0 because −2<0<π−2. The number c is one solution to the initial equation.

When are you not allowed to use the Intermediate Value Theorem?

The Intermediate Value Theorem should not be applied when the function is not continuous over the interval.

Use the Intermediate Value Theorem to show that each equation has at least one real solution.

1. cosx=−x

2. ln(x)=e −x +1

3. 2x 3 −5x 2 =10x−5

4. x 3 +1=x

5. x 2 =cosx

6. x 5 =2x 3 +2

7. 3x 2 +4x−11=0

8. 5x 4 =6x 2 +1

9. 7x 3 −18x 2 −4x+1=0

10. Show that f(x)= 2x−3 / 2x−5 has a real root on the interval [1,2].

11. Show that f(x)= 3x+1 / 2x+4 has a real root on the interval [−1,0].

12. True or false: A function has a maximum and a minimum in the closed interval [a,b]; therefore, the function is continuous.

13. True or false: A function is continuous over the interval [a,b]; therefore, the function has a maximum and a minimum in the closed interval.

14. True or false: If a function is continuous over the interval [a,b], then it is possible for the function to have more than one relative maximum in the interval [a,b].

15. What do the Intermediate Value and Extreme Value Theorems have to do with continuity?

Review (Answers)

To see the Review answers, open this PDF file and look for section 14.7.

Additional Resources

PLIX: Play, Learn, Interact, eXplore - Function Exploration

Video: Descartes' Rule of Signs - Example 1

Practice: Intermediate Value Theorem, Existence of Solutions

Real World: Ups and Downs

The Intermediate Value Theorem

We already know from the definition of continuity at a point that the graph of a function will not have a hole at any point where it is continuous. The Intermediate Value Theorem basically says that the graph of a continuous function on a closed interval will have no holes on that interval.

Statement of the Theorem

• Suppose $f(x)$ is continuous on the closed interval   $[a,b]$.   If $L$ is a real number between the values $f(a)$ and $f(b)$, but not equal to either of them, then there exists a number $c$ in the interval   $[a,b]$.   for which   $f(c)=L$.

The graph on the left below illustrates the situation described by the theorem. Since the function $f(x)$ is continuous, the endpoints of the interval are connected by the graph, and the function will pass through all values between $f(a)$ and $f(b)$. The graph on the right below shows a function that is defined everywhere on the closed interval   $[a,b]$,   but is not continuous on that interval. The lack of continuity allows for values between $f(a)$ and $f(b)$ that the function never takes on.

Using the Intermediate Value Theorem

Show that the function   $f(x)=x^{17}-3x^4+14$   is equal to 13 somewhere on the closed interval   $[0,1]$.

Proof of the Theorem

Even though the statement of the Intermediate Value Theorem seems quite obvious, its proof is actually quite involved, and we have broken it down into several pieces. First, we will discuss the Completeness Axiom, upon which the theorem is based. Then we shall prove Bolzano's Theorem, which is a similar result for a somewhat simpler situation. Then the Intermediate Value Theorem will follow almost immediately.

The Completeness Axiom

Axioms are statements in a mathematical system that are assumed to be true without proof. The Completeness Axiom is an axiom about the real numbers, and is sometimes phrased in the language of least upper bounds. A real number $x$ is called a least upper bound for a set $S$ if the following two properties are true:

• The number $x$ is an upper bound for the set $S$. (In other words, if $s$ is any number in set $S$, then   $s\le x$.)
• If   $y

Note that it is not necessary for an upper bound to be in the set $S$. Both upper bounds and least upper bounds could be numbers outside of set $S$.

The statement of the Completeness Axiom is:

• If $S$ is a nonempty set of real numbers that is bounded above, then there exists exactly one real number that is the least upper bound of $S$.

To understand this, we shall consider a few examples.

• If set $S$ is the closed interval   $[3,5]$,   then the number 5 is the least upper bound. The value 5 is certainly an upper bound, since for every $s$ in the interval,   $s\le 5$.   And for any number   $y<5$,   there is a larger number that is still in the set $S$, namely 5 itself, so $y$ is not an upper bound. The Completeness Axiom states that 5 is the only least upper bound of this interval.
• If set $S$ is the open interval   $(4,9)$,   then the number 9 is the least upper bound. The value 9 is certainly an upper bound, since for every $s$ in the interval,   $s\le 9$   (and in fact, $s$ is never equal to 9). And for any number   $y<9$,   there is a larger number that is still in the set $S$, namely $\dfrac{y+9}{2}$, so $y$ is not an upper bound. The Completeness Axiom states that 9 is the only least upper bound of this interval.
• If set $S$ is the interval   $(6,\infty)$,   then the hypothesis of the Completeness Axiom is not satisfied. In other words, set $S$ is not bounded above, because the values of $S$ continue on to infinity. Therefore, the conclusion of the Completeness Axiom is not guaranteed to follow. And in fact, in this case, the conclusion is not true, since there is no real number that is the least upper bound of this set.
• If set $S$ consists of the numbers of the sequence   $a_n=\left\{\left. 7-\dfrac{1}{n} \right| n \text{ is a positive integer}\right\}$,   then the number 7 is the least upper bound. The value 7 is certainly is an upper bound, since   $7-\dfrac{1}{n}\le 7$   for all positive integers. And for any number   $y \dfrac{1}{7-y}$.   This inequality, when solved for $y$, yields   $y<7-\dfrac{1}{n}$,   so $y$ is not an upper bound. Thus, the Completeness Axiom states that 7 is the only least upper bound for this set.

Bolzano's Theorem

The statement of Bolzano's Theorem is:

• Suppose $f(x)$ is continuous on the closed interval   $[a,b]$,   and suppose that $f(a)$ and $f(b)$ have opposite signs. Then there exists a number $c$ in the interval   $[a,b]$,   for which   $f(c)=0$.

Proof of the Intermediate Value Theorem

Having established Bolzano's Theorem, the Intermediate Value Theorem is a fairly straightforward corollary. First, we shall restate the theorem.

Intermediate Value Theorem

The intermediate value theorem (IVT) in calculus states that if a function f(x) is continuous over an interval [a, b], then the function takes on every value between f(a) and f(b). This theorem has very important applications like it is used:

• to verify whether there is a root of a given equation in a specified interval.
• to verify the existence of x-intercept of a function over a given interval, etc.

Let us learn more about the intermediate value theorem along with its proof and limitations along with examples.

What is Intermediate Value Theorem?

The intermediate value theorem (also known as IVT or IVT theorem) says that if a function f(x) is continuous on an interval [a, b], then for every y-value between f(a) and f(b), there exists some x-value in the interval (a, b). i.e., if f(x) is continuous on [a, b], then it should take every value that lies between f(a) and f(b). Recall that a continuous function is a function whose graph is a curve that can be drawn without lifting a pencil. Mathematically, this theorem is stated as follows:

Intermediate Value Theorem : "Suppose f(x) is a continuous function on [a, b] and L is a number that lies between f(a) and f(b), then there exists at least one 'c' such that c ∈ (a, b) (or a < c < b) and f(c) = L"

It is so obvious (because of continuity) how the theorem works and we can understand it from the following figure.

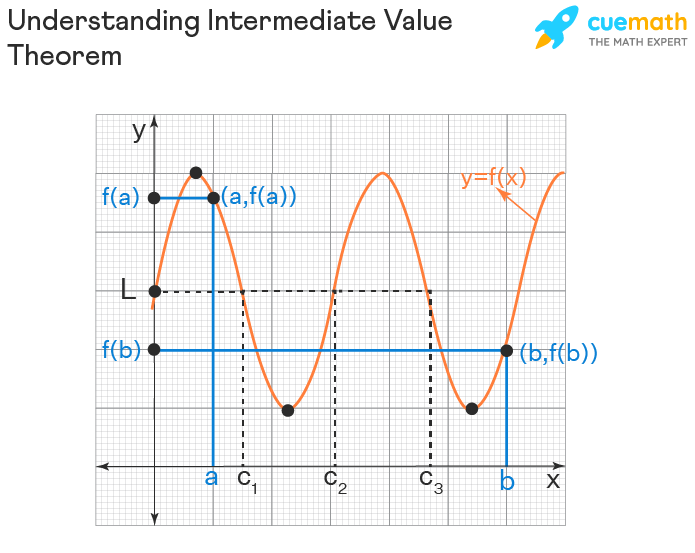
When the graph of f(x) can be drawn without lifting a pencil within the interval [a, b], it is so obvious that f(x) takes every value L that lies between f(a) and f(b). In fact, it is also possible that the equation f(c) = L can be possible for more than one value of 'c'. In the above figure, it is pretty clear that:

• f(c 1 ) = L
• f(c 2 ) = L
• f(c 3 ) = L

It means that there are three values c 1 , c 2 , and c 3 in the interval [a, b] whose function value is L.

Intermediate Value Theorem Proof

Though the proof of the intermediate value theorem is so pretty straight forward as mentioned earlier, here is the mathematical proof of the IVT theorem using the limit definition. Without loss of generality, assume that f(a) < L < f(b) and consider the following set :

A = { x ∈ [a, b]: f(x) < L}.

Notice that A is non-empty as a ∈ A (as f(a) < L) and also A is bounded above by 'b'.

Then it must have a supremum and let us assume it to be 'c'.

Proving c ∈ (a, b)

Because of the continuity of the function f(x) at 'a' and 'b', f(c) ≠ a and f(c) ≠ b.

Thus, c ∈ (a, b).

Proving f(c) = L

For this, we prove both f(c) ≤ L and f(c) ≥ L.

Proof of f(c) ≤ L:

Since 'c' is the supremum, by extreme value theorem , there is a sequence x n ∈ A with x n → c.

Since f(x) is continuous, f(x n ) → f(c).

But x n ∈ A, and by definition of A, f(x n ) < L.

⇒ f(c) = lim n → ∞ f(x n ) ≤ L.

Thus, we proved that f(c) ≤ L ... (1)

Proof of f(c) ≥ L:

Notice that c < b. Thus, for a very small 'n', t n = c + (1/n) < b.

Again, t n > c ⇒ t n > supremum of A. Thus, t n ∉ A.

Thus, f(t n ) ≥ L.

Since 'n' is very small and f(x) is continuous, f(t n ) → f(c). From this,

f(c) = lim n → ∞ f(t n ) ≥ L ... (2)

From (1) and (2), we have can conclude that f(c) = L.

Thus, the intermediate value theorem is proved.

Application of Intermediate Value Theorem

The important application of the intermediate value theorem is to verify the existence of a root of an equation in a given interval. In particular, the IVT theorem is used to see whether a given function has its zero ( x-intercept (or) f(x) = 0) within the given interval (a, b). To verify this, we follow the steps below:

• Step 1 : Find f(a) and f(b).
• Step 2 : If f(a) < 0 < f(b) (i.e., f(a) is negative and f(b) is positive) then f(x) has a zero (i.e., f(x) = 0) in the interval (a, b).

Let us examine the following example.

Example: Use the IVT to verify whether the function f(x) = x 3 - 3x - 19 has a zero in the interval [1, 5].

The interval is [1, 5]. Find f(1) and f(5).

f(1) = 1 3 - 3(1) - 19 = -21

f(5) = 5 3 - 3(5) - 19 = 91

Since f(1) is positive and f(5) is negative, by the intermediate value theorem, we can say that there must be some 'c' in [1, 5] such that f(c) = 0.

i.e., f(x) has a zero in the interval [1, 5].

Limitations of IVT Theorem

Observe the above example. Using the intermediate value theorem, we could only find whether the function f(x) has a root in the interval [1, 5]. But we couldn't find what root it is. Also, we couldn't verify how many roots f(x) has within the given interval (as we have seen in the figure of the first section , the function can take a value for more than one value of x). Further, there is one more limitation of the IVT theorem which can be understood from the example below:

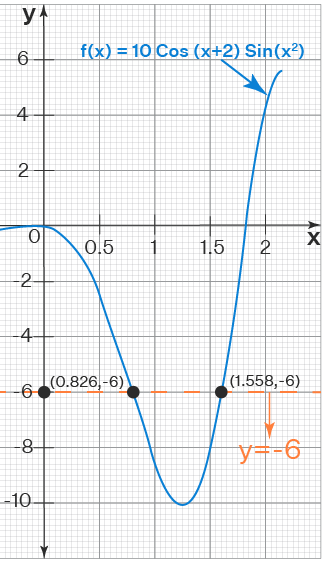
Example: Use the intermediate value theorem to verify whether the equation 10 cos (x + 2) sin (x 2 ) = -6 has a root in the interval [0.5, 2]?

Let f(x) = 10 cos (x + 2) sin (x 2 ).

The given interval is [0.5, 2]. So we will find f(0.5) and f(2).

f(0.5) = 10 cos (0.5 + 2) sin (0.5 2 ) = -1.98

f(2) = 10 cos (2 + 2) sin (2 2 ) = 4.95

We wanted to know whether f(x) = -6 has a solution in the given interval. We can see that -6 does NOT lie between -1.98 and 4.95. What do the values of f(0.5) and f(2) tell about the existence of the root of f(x) = -6 in the given interval? Unfortunately, the intermediate value theorem does not mean anything in this case. This theorem can guarantee only whether the function takes the value, but it cannot guarantee about a value that a function cannot take.

Thus, in the above example, we cannot say using the IVT theorem that f(x) = -6 does NOT have a root in the interval [0.5, 2]. In fact, it has two roots (0.826 and 1.558) in the given interval as shown in the graph below.

Important Notes on Intermediate Value Theorem:

• Using the intermediate value theorem, we can just know if the root exists; but it cannot guarantee if the root does not exist (see the above example).
• The IVT theorem cannot be applied when the function is NOT continuous.
• It does not help in finding the roots of an equation.
• We cannot say how many roots the equation has in the given interval using this theorem.

☛ Related Topics:

• Lagrange Mean Value Theorem
• Mean Value Theorem Formula
• Rolle's Theorem

Intermediate Value Theorem Examples

Example 1: Use the intermediate value theorem to verify whether a function f(x) = x + 1/x has a zero in the interval [-1, 1]?

The first condition to apply the IVT theorem is the function should be continuous in the given interval.

But the given function f(x) = x + 1/x is NOT continuous over [-1, 1] as f(0) is NOT defined here.

Thus, we cannot apply IVT here.

Answer: IVT cannot be applied here.

Example 2: Does the equation x 4 + 3x 2 - 2 = 0 has a solution in the interval [0, 1]? Explain your reasoning using IVT theorem.

Let us assume that f(x) = x 4 + 3x 2 - 2.

Since f(x) is a polynomial function , it is continuous.

We will find f(0) and f(1).

f(0) = 0 4 + 3(0) 2 - 2 = -2

f(1) = 1 4 + 3(1) 2 - 2 = 2

We want to know whether f(x) = 0 has any roots in the given interval. i.e., we have to see whether the function takes in the value 0. It is clear that -2 < 0 < 2 and hence by the intermediate value theorem, the given equation has at least one root in the given interval.

Answer: Yes, it has a root in [0, 1].

Example 3: Support your answer for Example 2 by graphing it.

In Example 2 , we have already proved by IVT that the given equation has a root in [0, 1]. Let us graph f(x) = x 4 + 3x 2 - 2 and see whether it has any roots (i.e., see whether it intersects the x-axis).

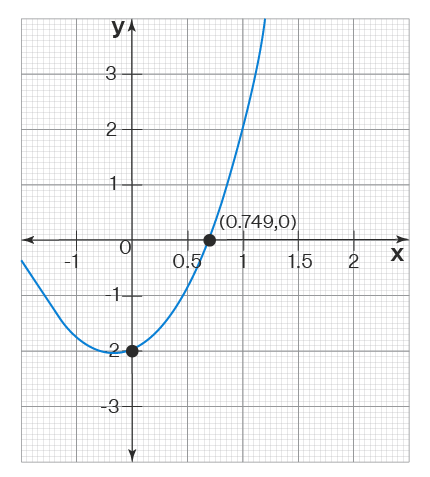
Since the graph cuts the x-axis at (0.749, 0), 0.749 is a root of the given function and it lies in the interval [0, 1].

Answer: The existence of the root is verified using the graph.

go to slide go to slide go to slide

Book a Free Trial Class

Practice Questions on Intermediate Value Theorem

go to slide go to slide

FAQs on Intermediate Value Theorem

What is ivt calculus.

IVT ( Intermediate Value Theorem ) in calculus states that a function f(x) that is continuous on a specified interval [a, b] takes every value that is between f(a) and f(b). i.e., for any value 'L' lying between f(a) and f(b), there exists at least one value c such that a < c < b and f(c) = L.

How Do You Know When to Use Intermediate Value Theorem?

If we want to know whether a function has a root in the given interval, then we can use the intermediate value theorem. Note that this theorem only when the function is continuous.

Can the IVT Theorem be Applied for Discontinuous Functions?

No, the intermediate value theorem cannot be applied for discontinuous functions . Because "continuity" is what is making a function f(x) on an interval [a, b] to take any value between f(a) and f(b).

What are the Conditions of the Intermediate Value Theorem?

The conditions of IVT to apply on a function f(x) over an interval [a, b] are:

• f(x) should be continuous on [a, b]
• f(a) ≠ f(b)

When Cannot We Use Intermediate Value Theorem?

The intermediate value theorem :

• says "yes the function takes a value" if it really takes
• but it cannot say "no the function doesn't take a value" if it doesn't take

Thus, for a function f(x) that is continuous on [a, b], if a value L does not lie between f(a) and f(b), it is not always true that there is no value such that f(c) = L. Sometimes, there can be values of 'c' satisfying f(c) = L.

How Can We Find Zeros Using IVT Theorem?

IVT theorem is used just to verify if the function has a zero in the given interval. But it is neither used in finding the number of zeros nor used in finding the number of zeros .

What is the Difference Between Mean Value Theorem and Intermediate Value Theorem?

The mean value theorem talks about the differentiable and continuous functions and the intermediate value theorem talks only about the continuous functions. For more information, click here .

1.5: the Intermediate Value Theorem

$\newcommand{\R}{\mathbb R }$ $\newcommand{\N}{\mathbb N }$ $\newcommand{\Z}{\mathbb Z }$ $\newcommand{\bfa}{\mathbf a}$ $\newcommand{\bfb}{\mathbf b}$ $\newcommand{\bff}{\mathbf f}$ $\newcommand{\bfu}{\mathbf u}$ $\newcommand{\bfx}{\mathbf x}$ $\newcommand{\bfy}{\mathbf y}$ $\newcommand{\ep}{\varepsilon}$

The Intermediate Value Theorem

Definition, examples of path-connected the Intermediate Value Theorem Problems

Definition, examples of path-connected

The familiar Intermediate Value Theorem (abbreviated IVT ) in 1d applies to a continuous function $f$ whose domain is an interval.

To state an analogue of the IVT in higher dimensions, we need to figure out what kind of domains the theorem should apply to.

A condition that is easy to state, intuitively reasonable, and good enough for all our purposes is path-connectedness . Informally, a set $S\subset \R^n$ is path-connected if any two points in $S$ can be connected by a path that stays in $S$. More precisely:

Definition. A set $S\subset \R^n$ is path-connected if, for every pair of points $\bfx$ and $\bfy$ in $S$, \begin{equation}\label{pc1} \exists \mbox{ continuous }\ \gamma:[0,1]\to S, \mbox{ such that }\gamma(0) = \bfx, \qquad \gamma(1) = \bfy. \end{equation}

(As is usual with mathematics, different sources sometimes use different terms. For example, Folland uses the terms arcwise connected or pathwise connected in place of path-connected .)

We emphasize that a key point in the definition is that $\gamma(s)$ is required to belong to $S$ for all $s\in [0,1]$

In general, our position will be that we can recognize path-connectedness when we see it. For example, consider the pictures below:

The set above is clearly path-connected set, and the set below clearly is not.

Because we can easily determine whether a set is path-connected by looking at it, we will not often go to the trouble of giving a rigorous mathematical proof of path-conectedness. If for some reason we decide to prove it, then normally the main point of a proof will be to write down in mathematical language an argument that captures our geometric intuition.

Here are a couple of examples:

Example 1 . A ball $B(r,\bfa)$ is path-connected.

Our geometric intuition: This is clear, because if $\bfx, \bfy$ both belong to $B(r,\bfa)$, then from drawing a picture, we are confident that the straight line segment that starts at $\bfx$ and ends at $\bfy$ is a continuous path joining $\bfx$ to $\bfy$.

If we decide to prove it: then we can just write down the above idea using mathematical language. We can interpret straight line segment that starts at $\bfx$ and ends at $\bfy$ as the function of the form $$ \gamma(t) = \bfb +t {\bf m} \quad\mbox{ for vectors }\bfb, {\bf m}\in \R^n, $$ where we will choose $\bfb$ and $\bf m$ so that $\gamma$ satisfies $\gamma(0)=\bfx, \gamma(1) = \bfy$, as required. This leads to \begin{equation}\label{segment} \boxed{ \gamma(t) = \bfx + t(\bfy - \bfx) = (1-t) \bfx + t\bfy } \end{equation} We have to check 3 things:

• $\gamma:[0,1]\to \R^n$ is continuous -- obvious!
• $\gamma(0)=\bfx$ and $\gamma(1) = \bfy$ --- obvious!
• $\gamma(t)\in B(r,\bfa)$ for all $t\in [0,1]$. In other words, we have to show that $|\gamma(t) - \bfa|<r$ for all $t\in [0,1]$. This is not obvious, but since the (mental) picture is very convincing, we can be confident that it's actually true. In fact this is the case, because for $t\in [0,1]$, \begin{align*} |\gamma(t) - \bfa| &= |(1-t) \bfx + t\bfy - \bfa| &\mbox{(definiton of $\gamma(t)$)}\\ &= |(1-t) (\bfx-\bfa) + t(\bfy - \bfa)| &\mbox{(rewrite)}\\ &\le |(1-t) (\bfx-\bfa)| + |t(\bfy - \bfa)| &\mbox{(triangle ineq.)}\\ %&= %|1-t|\, |\bfx-\bfa| + |t|\ |\bfy - \bfa| &\mbox{(triangle ineq.)}\\ &< (1-t) r + t r = r &\mbox{ since $\bfx, \bfy \in B(r,\bfa)$}. \end{align*} Thus $B(r,\bfa)$ satisfies condition \eqref{pc1}, and hence it is path-connected.

Remark. The formula \eqref{segment} for a path corresponding to a straight-line segment that starts at $\bfx$ and ends at $\bfy$ appears very frequently in mathematics, and should be committed to memory.

Example 2 . If $S_1$ and $S_2$ are path-connected, and $S_1\cap S_2 \ne \emptyset$, then $S := S_1\cup S_2$ is path-connected.

Our geometric intuition: Consider any $\bfx$ and $\bfy$ in $S$.

To find a path connecting these two points, let $\bf z$ be an element of $S_1\cap S_2$.

Then since $\bf z$ and $\bfx$ both belong to either $S_1$ or $S_2$, we can find a continuous path connecting $\bfx$ to $\bf z$ that stays in that set, and hence in $S$.

Similarly we can find a continuous path connecting $\bf z$ to $\bfy$ that stays in $S$.

Now we can move continuously from $\bfx$ to $\bfy$, while staying inside $S$, by concatenating the paths, i.e. first following the continuous path from $\bfx$ to $\bf z$; then following the continuous path from $\bf z$ to $\bfy$.

In this way, we can find a path conecting any two points $\bfx$ and $\bfy$.

If we decide to prove it : we will just follow the above geometric intuition.

Suppose we are given any two points $\bfx, \bfy$ in $S_1\cup S_2$.

Fix a point ${\bf z}\in S_1\cap S_2$. Here we are using the hypothesis that $S_1\cap S_2\ne \emptyset$.

Since $\bfx$ belongs to either $S_1$ or $S_2$, and $\bf z$ belongs to both $S_1$ and $S_2$, it is clear that one of these two path-connected sets --- either $S_1$ or $S_2$ --- must contain both $\bfx$ and $\bf z$. Thus these two points can be connected by a path, say $\gamma_x$, that stays in this set, and hence inside $S = S_1\cup S_2$. That is, $$ \exists \mbox{ continuous }\gamma_x:[0,1]\to S \mbox{ such that }\gamma_x(0) = \bfx, \qquad \gamma_x(1) ={\bf z}. $$

By exactly the same reasoning, $$ \exists\mbox{ continuous }\gamma_y:[0,1]\to S \mbox{ such that }\gamma_y(0) = {\bf z}, \qquad \gamma_y(1) ={\bf y}. $$

Now we have to figure out how to write down our idea of joining the two paths together to obtain a single continuous function $\gamma:[0,1]\to S$ that starts that $\bfx$ and ends at $\bfy$. As a preliminary step, define $\widetilde \gamma:[0,2]\to S$ by \begin{equation}\label{tg} \widetilde \gamma(t) := \begin{cases} \gamma_x(t) &\mbox{ if }t\in [0,1]\\ \gamma_y(t-1) &\mbox{ if }t\in [1,2] \ . \end{cases} \end{equation} This satisfies $$ \widetilde \gamma\mbox{ is continuous}, \qquad \widetilde \gamma(0) = \bfx, \qquad \widetilde \gamma(2) = \bfy. $$ (The detailed $\ep-\delta$ proof of continuity is left as a practice problem for those who are interested.) The only problem is that we want a function that ends up at $\bfy$ at $t=1$ rather than $t=2$. But this is easy to fix, by defining $$ \gamma(t) := \widetilde \gamma(2t),\qquad t\in [0,1]. $$ Then $\gamma$ satisfies \eqref{pc1}. Since $\bfx$ and $\bfy$ were arbitrary points in $S = S_1\cup S_2$, it follows that $S$ is path-connected. $\quad \Box$

Having given the definition of path-connected and seen some examples, we now state an $n$-dimensional version of the Intermediate Value Theorem, for which path-connectedness is the main geometric hypothesis.

Theorem 1: the Intermediate Value Theorem. Assume that $S$ is a path-connected subset of $\R^n$ and that $f:S\to \R$ is continuous. If $\bfa, \bfb$ are points in $S$ and $$ \mbox{ either } f(\bfa) < t <f(\bfb), \qquad \mbox{ or }\quad f(\bfb) < t <f(\bfa), $$ then there exists a point ${\bf c} \in S$ such that $f({\bf c})=t$.

Because $S$ is path-connected, there exists a continuous $\gamma:[0,1]\to S$ such that $\gamma(0)=\bfa$ and $\gamma(1) = \bfb$.

Now define $\phi: [0,1]\to \R$ by $\phi = f\circ \gamma$.

Because both $f$ and $\gamma$ are continuous, we know that $\phi$ is continuous. Also, by hypothesis $$ \mbox{ either } \ \ \phi(0) = f(\bfa) < t <f(\bfb)= \phi(1) , \qquad \mbox{ or }\quad \phi(1) = f(\bfb) < t <f(\bfa) =\phi(0). $$ So the Intermediate Value Theorem for functions of a single variable implies that there exists $s\in [0,1]$ such that $\phi(s) = t$.

But since $\phi(s) = f(\gamma(s))$, if we define ${\bf c} := \gamma(s)$, then ${\bf c} \in S$ and $f({\bf c})=t$. This proves the existence of a point $\bf c$ with the desired properties. $\quad\Box$

As with functions of a single variable, the theorem can be used to show that certain equations have solutions. The following example is a little artificial but it illustrates this point.

Example 3 . Prove that the equation $$ x^2+2y^2 = e^{(z-\frac 12)^2 cos(e^{-\sin (y/(x+2))}) } $$ has a solution in the ball $B(2, {\bf 0})\subset \R^3$.

To solve this, let's rewrite the problem as $f(x,y,z)=0$ for $f(x,y,z) = x^2+2y^2 - e^{(z-\frac 12)^2 cos(e^{-\sin (y/(x+2))})}$. Note that $f(0,0,\frac 12) = -e^0 = -1 0$. Both of these points belong to $B(2,{\bf 0})$. We also know that balls are pathwise connected, so it follows from the Intermediate Value Theorem that there must exist some point in $B(2, {\bf 0})$ where $f=0$.

Example 4 Assume that $S$ is a subset of $\R^n$. The Intermediate Value Theorem implies if there exists a continuous function $f:S\to \R$ and a number $c\in \R$ and points $\bfa,\bfb\in S$ such that $$ f(\bfa)< c, \qquad f(\bfb)>c, \qquad f(\bfx)\ne c \mbox{ for any }\bfx\in S $$ then $S$ is not path-connected.

This can be used to prove that some sets $S$ are not path connected. For example, let $$ S := \{(x,y)\in \R^2 : x^2 - y^2 \ge 1 \}. $$ Let $f(x,y) = x$, and let $\bfa = (-1,0)$ and $\bfb= (1,0)$. Clearly $f(\bfa) = -1$ and $f(\bfb)=1$. But $S$ cannot contain any points where $f=0$, since $$ f(x,y)=0 \Rightarrow x=0 \Rightarrow x^2-y^2 = -y^2 \le 0 \Rightarrow (x,y)\not \in S. $$

Basic skills

Given an example of a set that is/is not path-connected

Determine (without proof) whether a given set is path-connected. For example, let $Q_j\subset \R^2$ denote the (open) $j$th quadrant, for $j=1,\ldots, 4$, so that $$ Q_1= \{(x,y)\in \R^2 : x>0\mbox{ and }y>0\}, \qquad Q_3= \{(x,y)\in \R^2 : x< 0\mbox{ and }y< 0\}, $$ Determine which of the following sets are path-connected:

• $Q_1\cup Q_3$
• $\bar Q_1 \cup Q_3$
• $\bar Q_1 \cup \bar Q_3$.
• $\partial Q_1\cup Q_3$.

Show that the equation $f(\bfx)= 0$ has a solution on the set $S = \cdots$. See Example 3 above. Strictly speaking, we should only ask this question when $S$ is a set that we have proved is path-connected, such as a ball.

More advanced/abstract questions

Prove that the function $\widetilde \gamma$, defined in \eqref{tg}, is continuous.

Detrmine whether a set is path-connected, and explain why your answer is correct. Consider for example the same sets appearing in the Basic Skills above. (Recall that in this class, explain means: give a mathematical argument that may fall short of a full proof but that commnunicates a basic relevant mathematical idea. In this case, to explain why a set is path-connected, you might for example describe, in words, how to connect any two points in a set by a continuous path, without writing down a formula for the path.)

If $S_1$ and $S_2$ are path-connected sets in $\R^2$, must it be true that $S_1\cap S_2$ is path-connected? Explain your answer.

A set $S\subset \R^n$ is defined to be star-shaped about the origin if, $$ \mbox{ for every $\bfx \in S$ and every $\lambda\in [0,1]$ }, \qquad\mbox{ the point }\lambda \bfx \mbox{ belongs to $S$}. $$

• Is a ball $B(r, {\bf 0})$ star-shaped about the origin?
• Draw a picture of a set $S \subset\R^2$ that is star-shaped about the origin and that contains the points $(10,0)$ and $(0,10)$, but not the point $(1,1)$.
• It is a fact that every set that is star-shaped about the origin is path-connected. Give a pictorial argument to explain why this is true.
• If you wish, prove that every set that is star-shaped about the origin is path-connected (presumably by converting your pictorial argument into a mathematical proof).

Let $$ S^+ := \{ \bfx = (x_1,\ldots, x_n)\in \R^n : |\bfx|=1, \ x_n \ge 0 \}. $$ and $$ S^- := \{ \bfx = (x_1,\ldots, x_n)\in \R^n : |\bfx|=1, \ x_n \le 0 \}. $$ One might call these sets the upper (unit) hemisphere and lower (unit) hemisphere respectively.

• Draw a picture of $S^+$ when $n=3$.
• Determine (without proof) whether $S^+$ is path-connected.
• If you wish, give a proof to show that your answer to the above question is correct. For this, it may be helpful to write a point $\ \bfx = (x_1,\ldots, x_n)\in S^+$ in the form $$ \bfx = (x_1,\ldots, x_{n-1}, f(x_1,\ldots, x_{n-1})) $$ where $$ x_1^2 +\ldots + x_{n-1}^2 \le 1, \quad\mbox{ and }\quad f(x_1,\ldots, x_{n-1}) =\sqrt{1- x_1^2 - \cdots -x_{n-1}^2}. $$

Let $S$ denote the unit sphere in $\R^n$: $$ S := \{ \bfx \in \R^n : |\bfx|=1, \}. $$

• Determine (without proof) whether $S$ is path-connected.
• If you wish, give a proof to show that your answer to the above question is correct.

Let $S$ denote the unit sphere in $\R^n$, as in the previous problem, and assume that $f:S\to \R$ is continuous. Prove that there exists some $\bfx\in S$ such that $f(\bfx) = f(-\bfx)$. (This implies, for example, that at any given moment, there are two exactly antipodal points on the earth where the temperature is exactly the same.) hints :

• Consider the function $g(\bfx) := f(\bfx) - f(-\bfx)$.
• If you have done the previous problem correctly, you now know that $S$ is pathwise connected. Use this fact in your solution.

$\Leftarrow$    $\Uparrow$    $\Rightarrow$

Understanding Analysis and its Connections to Secondary Mathematics Teaching pp 93–109 Cite as

The Intermediate Value Theorem and Implicit Assumptions

• Nicholas H. Wasserman 6 ,
• Timothy Fukawa-Connelly 7 ,
• Keith Weber 8 ,
• Juan Pablo Mejia-Ramos 8 &
• Stephen Abbott 9  
• First Online: 24 September 2021

530 Accesses

Part of the book series: Springer Texts in Education ((SPTE))

This chapter engages with the content and proof of the Intermediate Value Theorem; similar to Abbott’s (Understanding analysis (2nd ed.). New York, NY: Springer (2015)) Section 4.5. Using this theorem as a case study, we explore the different ways explicit and implicit assumptions play a role in the back and forth communications between teachers and students in the classroom.

• Semantic contamination
• Concept definition
• Concept image
• Intermediate value theorem
• Cartesian product
• Intermediate Zero Theorem
• Implicit assumptions
• Intermediate value property

This is a preview of subscription content, log in via an institution .

Buying options

• Available as PDF
• Read on any device
• Instant download
• Own it forever
• Available as EPUB and PDF
• Compact, lightweight edition
• Dispatched in 3 to 5 business days
• Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Tall and Vinner [ 5 ] make a similar differentiation. They contrast a concept definition , by which they mean a formal definition, with a concept image , by which they mean all the other interesting things associated with that concept.

Brown and Walter [ 3 ] describe this as the “what-if-not” strategy for problem posing.

Ball and Bass [ 2 ] describe this notion of unpacking; teachers need to be able to “deconstruct [their] own mathematical knowledge into less polished and final form, where elemental components are accessible and visible” (p. 98).

Abbott, S. (2015). Understanding analysis (2nd ed.). New York, NY: Springer.

Book   Google Scholar  

Ball, D. L., & Bass, H. (2000). Interweaving content and pedagogy in teaching and learning to teach: Knowing and using mathematics. In J. Boaler (Ed.), Multiple perspectives on mathematics teaching and learning (pp. 83–104). Westport, CT: Ablex.

Google Scholar  

Brown, S. I., & Walter, M. I. (2005). The art of problem posing (3rd ed.). Mahwah, NJ: Lawrence Erlbaum.

Jakobsen, A., Thames, M. H., Ribeiro, C. M., & Delaney, S. (2012). Using practice to define and distinguish horizon content knowledge. In Proceedings of the 12th International Congress on Mathematical Education (pp. 4635–4644). Seoul, South Korea: International Commission on Mathematical Instruction.

Tall, D., & Vinner, S. (1981). Concept image and concept definition in mathematics with particular reference to limits and continuity. Educational Studies in Mathematics , 12 (2), 151–169.

Article   Google Scholar  

Download references

Author information

Authors and affiliations.

Teachers College, Columbia University, New York, NY, USA

Nicholas H. Wasserman

Temple University, Philadelphia, PA, USA

Timothy Fukawa-Connelly

Graduate School of Education, Rutgers University, New Brunswick, NJ, USA

Keith Weber & Juan Pablo Mejia-Ramos

Department of Mathematics, Middlebury College, Middlebury, VT, USA

Stephen Abbott

You can also search for this author in PubMed   Google Scholar

Rights and permissions

Reprints and permissions

Copyright information

© 2022 The Author(s), under exclusive license to Springer Nature Switzerland AG

About this chapter

Cite this chapter.

Wasserman, N.H., Fukawa-Connelly, T., Weber, K., Pablo Mejia-Ramos, J., Abbott, S. (2022). The Intermediate Value Theorem and Implicit Assumptions. In: Understanding Analysis and its Connections to Secondary Mathematics Teaching. Springer Texts in Education. Springer, Cham. https://doi.org/10.1007/978-3-030-89198-5_7

Download citation

DOI : https://doi.org/10.1007/978-3-030-89198-5_7

Published : 24 September 2021

Publisher Name : Springer, Cham

Print ISBN : 978-3-030-89197-8

Online ISBN : 978-3-030-89198-5

eBook Packages : Education Education (R0)

Share this chapter

Anyone you share the following link with will be able to read this content:

Sorry, a shareable link is not currently available for this article.

Provided by the Springer Nature SharedIt content-sharing initiative

• Publish with us

Policies and ethics

• Find a journal
• Track your research

You are about to erase your work on this activity. Are you sure you want to do this?

Updated Version Available

There is an updated version of this activity. If you update to the most recent version of this activity, then your current progress on this activity will be erased. Regardless, your record of completion will remain. How would you like to proceed?

Mathematical Expression Editor

Here we see a consequence of a function being continuous.

Now, let’s contrast this with a time when the conclusion of the Intermediate Value Theorem does not hold.

Building on the question above, it is not difficult to see that each of the hypothesis of the Intermediate Value Theorem are necessary.

Let’s see the Intermediate Value Theorem in action.

This example also points the way to a simple method for approximating roots.

Now we move on to a more subtle example:

• They start and finish drinking at the same times.
• Roxy starts with more water than Yuri, and leaves less water left in her bowl than Yuri.

And finally, an example when the Intermediate Value Theorem does not apply.

• They start and finish eating at the same times.
• Roxy starts with more food than Yuri, and leaves less food uneaten than Yuri.

4.4 The Mean Value Theorem

Learning objectives.

• 4.4.1 Explain the meaning of Rolle’s theorem.
• 4.4.2 Describe the significance of the Mean Value Theorem.
• 4.4.3 State three important consequences of the Mean Value Theorem.

The Mean Value Theorem is one of the most important theorems in calculus. We look at some of its implications at the end of this section. First, let’s start with a special case of the Mean Value Theorem, called Rolle’s theorem.

Rolle’s Theorem

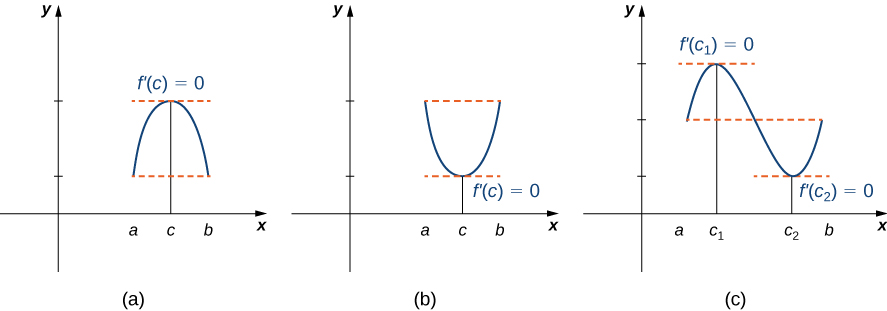
Informally, Rolle’s theorem states that if the outputs of a differentiable function f f are equal at the endpoints of an interval, then there must be an interior point c c where f ′ ( c ) = 0 . f ′ ( c ) = 0 . Figure 4.21 illustrates this theorem.

Theorem 4.4

Let f f be a continuous function over the closed interval [ a , b ] [ a , b ] and differentiable over the open interval ( a , b ) ( a , b ) such that f ( a ) = f ( b ) . f ( a ) = f ( b ) . There then exists at least one c ∈ ( a , b ) c ∈ ( a , b ) such that f ′ ( c ) = 0 . f ′ ( c ) = 0 .

Let k = f ( a ) = f ( b ) . k = f ( a ) = f ( b ) . We consider three cases:

• f ( x ) = k f ( x ) = k for all x ∈ ( a , b ) . x ∈ ( a , b ) .
• There exists x ∈ ( a , b ) x ∈ ( a , b ) such that f ( x ) > k . f ( x ) > k .
• There exists x ∈ ( a , b ) x ∈ ( a , b ) such that f ( x ) < k . f ( x ) < k .

Case 1: If f ( x ) = k f ( x ) = k for all x ∈ ( a , b ) , x ∈ ( a , b ) , then f ′ ( x ) = 0 f ′ ( x ) = 0 for all x ∈ ( a , b ) . x ∈ ( a , b ) .

Case 2: Since f f is a continuous function over the closed, bounded interval [ a , b ] , [ a , b ] , by the extreme value theorem, it has an absolute maximum. Also, since there is a point x ∈ ( a , b ) x ∈ ( a , b ) such that f ( x ) > k , f ( x ) > k , the absolute maximum is greater than k . k . Therefore, the absolute maximum does not occur at either endpoint. As a result, the absolute maximum must occur at an interior point c ∈ ( a , b ) . c ∈ ( a , b ) . Because f f has a maximum at an interior point c , c , and f f is differentiable at c , c , by Fermat’s theorem, f ′ ( c ) = 0 . f ′ ( c ) = 0 .

Case 3: The case when there exists a point x ∈ ( a , b ) x ∈ ( a , b ) such that f ( x ) < k f ( x ) < k is analogous to case 2, with maximum replaced by minimum.

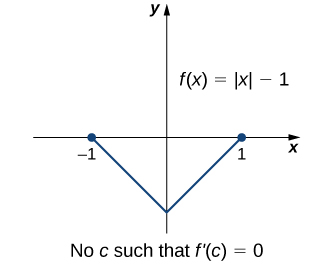
An important point about Rolle’s theorem is that the differentiability of the function f f is critical. If f f is not differentiable, even at a single point, the result may not hold. For example, the function f ( x ) = | x | − 1 f ( x ) = | x | − 1 is continuous over [ −1 , 1 ] [ −1 , 1 ] and f ( −1 ) = 0 = f ( 1 ) , f ( −1 ) = 0 = f ( 1 ) , but f ′ ( c ) ≠ 0 f ′ ( c ) ≠ 0 for any c ∈ ( −1 , 1 ) c ∈ ( −1 , 1 ) as shown in the following figure.

Let’s now consider functions that satisfy the conditions of Rolle’s theorem and calculate explicitly the points c c where f ′ ( c ) = 0 . f ′ ( c ) = 0 .

Example 4.14

Using rolle’s theorem.

For each of the following functions, verify that the function satisfies the criteria stated in Rolle’s theorem and find all values c c in the given interval where f ′ ( c ) = 0 . f ′ ( c ) = 0 .

• f ( x ) = x 2 + 2 x f ( x ) = x 2 + 2 x over [ −2 , 0 ] [ −2 , 0 ]
• f ( x ) = x 3 − 4 x f ( x ) = x 3 − 4 x over [ −2 , 2 ] [ −2 , 2 ]

Checkpoint 4.14

Verify that the function f ( x ) = 2 x 2 − 8 x + 6 f ( x ) = 2 x 2 − 8 x + 6 defined over the interval [ 1 , 3 ] [ 1 , 3 ] satisfies the conditions of Rolle’s theorem. Find all points c c guaranteed by Rolle’s theorem.

The Mean Value Theorem and Its Meaning

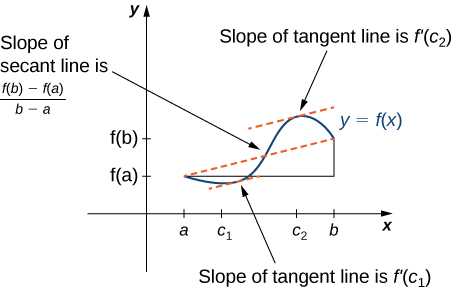
Rolle’s theorem is a special case of the Mean Value Theorem. In Rolle’s theorem, we consider differentiable functions f f defined on a closed interval [ a , b ] [ a , b ] with f ( a ) = f ( b ) f ( a ) = f ( b ) . The Mean Value Theorem generalizes Rolle’s theorem by considering functions that do not necessarily have equal value at the endpoints. Consequently, we can view the Mean Value Theorem as a slanted version of Rolle’s theorem ( Figure 4.25 ). The Mean Value Theorem states that if f f is continuous over the closed interval [ a , b ] [ a , b ] and differentiable over the open interval ( a , b ) , ( a , b ) , then there exists a point c ∈ ( a , b ) c ∈ ( a , b ) such that the tangent line to the graph of f f at c c is parallel to the secant line connecting ( a , f ( a ) ) ( a , f ( a ) ) and ( b , f ( b ) ) . ( b , f ( b ) ) .

Theorem 4.5

Mean value theorem.

Let f f be continuous over the closed interval [ a , b ] [ a , b ] and differentiable over the open interval ( a , b ) . ( a , b ) . Then, there exists at least one point c ∈ ( a , b ) c ∈ ( a , b ) such that

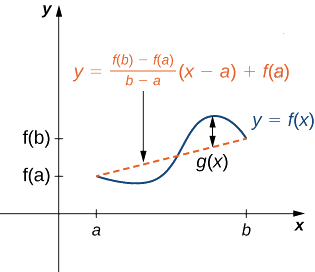
The proof follows from Rolle’s theorem by introducing an appropriate function that satisfies the criteria of Rolle’s theorem. Consider the line connecting ( a , f ( a ) ) ( a , f ( a ) ) and ( b , f ( b ) ) . ( b , f ( b ) ) . Since the slope of that line is

and the line passes through the point ( a , f ( a ) ) , ( a , f ( a ) ) , the equation of that line can be written as

Let g ( x ) g ( x ) denote the vertical difference between the point ( x , f ( x ) ) ( x , f ( x ) ) and the point ( x , y ) ( x , y ) on that line. Therefore,

Since the graph of f f intersects the secant line when x = a x = a and x = b , x = b , we see that g ( a ) = 0 = g ( b ) . g ( a ) = 0 = g ( b ) . Since f f is a differentiable function over ( a , b ) , ( a , b ) , g g is also a differentiable function over ( a , b ) . ( a , b ) . Furthermore, since f f is continuous over [ a , b ] , [ a , b ] , g g is also continuous over [ a , b ] . [ a , b ] . Therefore, g g satisfies the criteria of Rolle’s theorem. Consequently, there exists a point c ∈ ( a , b ) c ∈ ( a , b ) such that g ′ ( c ) = 0 . g ′ ( c ) = 0 . Since

we see that

Since g ′ ( c ) = 0 , g ′ ( c ) = 0 , we conclude that

In the next example, we show how the Mean Value Theorem can be applied to the function f ( x ) = x f ( x ) = x over the interval [ 0 , 9 ] . [ 0 , 9 ] . The method is the same for other functions, although sometimes with more interesting consequences.

Example 4.15

Verifying that the mean value theorem applies.

For f ( x ) = x f ( x ) = x over the interval [ 0 , 9 ] , [ 0 , 9 ] , show that f f satisfies the hypothesis of the Mean Value Theorem, and therefore there exists at least one value c ∈ ( 0 , 9 ) c ∈ ( 0 , 9 ) such that f ′ ( c ) f ′ ( c ) is equal to the slope of the line connecting ( 0 , f ( 0 ) ) ( 0 , f ( 0 ) ) and ( 9 , f ( 9 ) ) . ( 9 , f ( 9 ) ) . Find these values c c guaranteed by the Mean Value Theorem.

We know that f ( x ) = x f ( x ) = x is continuous over [ 0 , 9 ] [ 0 , 9 ] and differentiable over ( 0 , 9 ) . ( 0 , 9 ) . Therefore, f f satisfies the hypotheses of the Mean Value Theorem, and there must exist at least one value c ∈ ( 0 , 9 ) c ∈ ( 0 , 9 ) such that f ′ ( c ) f ′ ( c ) is equal to the slope of the line connecting ( 0 , f ( 0 ) ) ( 0 , f ( 0 ) ) and ( 9 , f ( 9 ) ) ( 9 , f ( 9 ) ) ( Figure 4.27 ). To determine which value(s) of c c are guaranteed, first calculate the derivative of f . f . The derivative f ′ ( x ) = 1 ( 2 x ) . f ′ ( x ) = 1 ( 2 x ) . The slope of the line connecting ( 0 , f ( 0 ) ) ( 0 , f ( 0 ) ) and ( 9 , f ( 9 ) ) ( 9 , f ( 9 ) ) is given by

We want to find c c such that f ′ ( c ) = 1 3 . f ′ ( c ) = 1 3 . That is, we want to find c c such that

Solving this equation for c , c , we obtain c = 9 4 . c = 9 4 . At this point, the slope of the tangent line equals the slope of the line joining the endpoints.

One application that helps illustrate the Mean Value Theorem involves velocity. For example, suppose we drive a car for 1 h down a straight road with an average velocity of 45 mph. Let s ( t ) s ( t ) and v ( t ) v ( t ) denote the position and velocity of the car, respectively, for 0 ≤ t ≤ 1 0 ≤ t ≤ 1 h. Assuming that the position function s ( t ) s ( t ) is differentiable, we can apply the Mean Value Theorem to conclude that, at some time c ∈ ( 0 , 1 ) , c ∈ ( 0 , 1 ) , the speed of the car was exactly

Example 4.16

Mean value theorem and velocity.

If a rock is dropped from a height of 100 ft, its position t t seconds after it is dropped until it hits the ground is given by the function s ( t ) = −16 t 2 + 100 . s ( t ) = −16 t 2 + 100 .

• Determine how long it takes before the rock hits the ground.
• Find the average velocity v avg v avg of the rock for when the rock is released and the rock hits the ground.
• Find the time t t guaranteed by the Mean Value Theorem when the instantaneous velocity of the rock is v avg . v avg .
• When the rock hits the ground, its position is s ( t ) = 0 . s ( t ) = 0 . Solving the equation −16 t 2 + 100 = 0 −16 t 2 + 100 = 0 for t , t , we find that t = ± 5 2 sec . t = ± 5 2 sec . Since we are only considering t ≥ 0 , t ≥ 0 , the ball will hit the ground 5 2 5 2 sec after it is dropped.
• The average velocity is given by v avg = s ( 5 / 2 ) − s ( 0 ) 5 / 2 − 0 = 0 − 100 5 / 2 = −40 ft/sec . v avg = s ( 5 / 2 ) − s ( 0 ) 5 / 2 − 0 = 0 − 100 5 / 2 = −40 ft/sec .

Checkpoint 4.15

Suppose a ball is dropped from a height of 200 ft. Its position at time t t is s ( t ) = −16 t 2 + 200 . s ( t ) = −16 t 2 + 200 . Find the time t t when the instantaneous velocity of the ball equals its average velocity.

Corollaries of the Mean Value Theorem

Let’s now look at three corollaries of the Mean Value Theorem. These results have important consequences, which we use in upcoming sections.

At this point, we know the derivative of any constant function is zero. The Mean Value Theorem allows us to conclude that the converse is also true. In particular, if f ′ ( x ) = 0 f ′ ( x ) = 0 for all x x in some interval I , I , then f ( x ) f ( x ) is constant over that interval. This result may seem intuitively obvious, but it has important implications that are not obvious, and we discuss them shortly.

Theorem 4.6

Corollary 1: functions with a derivative of zero.

Let f f be differentiable over an interval I . I . If f ′ ( x ) = 0 f ′ ( x ) = 0 for all x ∈ I , x ∈ I , then f ( x ) = f ( x ) = constant for all x ∈ I . x ∈ I .

Since f f is differentiable over I , I , f f must be continuous over I . I . Suppose f ( x ) f ( x ) is not constant for all x x in I . I . Then there exist a , b ∈ I , a , b ∈ I , where a ≠ b a ≠ b and f ( a ) ≠ f ( b ) . f ( a ) ≠ f ( b ) . Choose the notation so that a < b . a < b . Therefore,

Since f f is a differentiable function, by the Mean Value Theorem, there exists c ∈ ( a , b ) c ∈ ( a , b ) such that

Therefore, there exists c ∈ I c ∈ I such that f ′ ( c ) ≠ 0 , f ′ ( c ) ≠ 0 , which contradicts the assumption that f ′ ( x ) = 0 f ′ ( x ) = 0 for all x ∈ I . x ∈ I .

From Corollary 1: Functions with a Derivative of Zero , it follows that if two functions have the same derivative, they differ by, at most, a constant.

Theorem 4.7

Corollary 2: constant difference theorem.

If f f and g g are differentiable over an interval I I and f ′ ( x ) = g ′ ( x ) f ′ ( x ) = g ′ ( x ) for all x ∈ I , x ∈ I , then f ( x ) = g ( x ) + C f ( x ) = g ( x ) + C for some constant C . C .

Let h ( x ) = f ( x ) − g ( x ) . h ( x ) = f ( x ) − g ( x ) . Then, h ′ ( x ) = f ′ ( x ) − g ′ ( x ) = 0 h ′ ( x ) = f ′ ( x ) − g ′ ( x ) = 0 for all x ∈ I . x ∈ I . By Corollary 1, there is a constant C C such that h ( x ) = C h ( x ) = C for all x ∈ I . x ∈ I . Therefore, f ( x ) = g ( x ) + C f ( x ) = g ( x ) + C for all x ∈ I . x ∈ I .

The third corollary of the Mean Value Theorem discusses when a function is increasing and when it is decreasing. Recall that a function f f is increasing over I I if f ( x 1 ) < f ( x 2 ) f ( x 1 ) < f ( x 2 ) whenever x 1 < x 2 , x 1 < x 2 , whereas f f is decreasing over I I if f ( x ) 1 > f ( x 2 ) f ( x ) 1 > f ( x 2 ) whenever x 1 < x 2 . x 1 < x 2 . Using the Mean Value Theorem, we can show that if the derivative of a function is positive, then the function is increasing; if the derivative is negative, then the function is decreasing ( Figure 4.29 ). We make use of this fact in the next section, where we show how to use the derivative of a function to locate local maximum and minimum values of the function, and how to determine the shape of the graph.

This fact is important because it means that for a given function f , f , if there exists a function F F such that F ′ ( x ) = f ( x ) ; F ′ ( x ) = f ( x ) ; then, the only other functions that have a derivative equal to f f are F ( x ) + C F ( x ) + C for some constant C . C . We discuss this result in more detail later in the chapter.

Theorem 4.8

Corollary 3: increasing and decreasing functions.

Let f f be continuous over the closed interval [ a , b ] [ a , b ] and differentiable over the open interval ( a , b ) . ( a , b ) .

• If f ′ ( x ) > 0 f ′ ( x ) > 0 for all x ∈ ( a , b ) , x ∈ ( a , b ) , then f f is an increasing function over [ a , b ] . [ a , b ] .
• If f ′ ( x ) < 0 f ′ ( x ) < 0 for all x ∈ ( a , b ) , x ∈ ( a , b ) , then f f is a decreasing function over [ a , b ] . [ a , b ] .

We will prove i.; the proof of ii. is similar. Suppose f f is not an increasing function on I . I . Then there exist a a and b b in I I such that a < b , a < b , but f ( a ) > f ( b ) . f ( a ) > f ( b ) . Since f f is a differentiable function over I , I , by the Mean Value Theorem there exists c ∈ ( a , b ) c ∈ ( a , b ) such that

Since f ( a ) > f ( b ) , f ( a ) > f ( b ) , we know that f ( b ) − f ( a ) < 0 . f ( b ) − f ( a ) < 0 . Also, a < b a < b tells us that b − a > 0 . b − a > 0 . We conclude that

However, f ′ ( x ) > 0 f ′ ( x ) > 0 for all x ∈ I . x ∈ I . This is a contradiction, and therefore f f must be an increasing function over I . I .

Section 4.4 Exercises

Why do you need continuity to apply the Mean Value Theorem? Construct a counterexample.

Why do you need differentiability to apply the Mean Value Theorem? Find a counterexample.

When are Rolle’s theorem and the Mean Value Theorem equivalent?

If you have a function with a discontinuity, is it still possible to have f ′ ( c ) ( b − a ) = f ( b ) − f ( a ) ? f ′ ( c ) ( b − a ) = f ( b ) − f ( a ) ? Draw such an example or prove why not.

For the following exercises, determine over what intervals (if any) the Mean Value Theorem applies. Justify your answer.

y = sin ( π x ) y = sin ( π x )

y = 1 x 3 y = 1 x 3

y = 4 − x 2 y = 4 − x 2

y = x 2 − 4 y = x 2 − 4

y = ln ( 3 x − 5 ) y = ln ( 3 x − 5 )

For the following exercises, graph the functions on a calculator and draw the secant line that connects the endpoints. Estimate the number of points c c such that f ′ ( c ) ( b − a ) = f ( b ) − f ( a ) . f ′ ( c ) ( b − a ) = f ( b ) − f ( a ) .

[T] y = 3 x 3 + 2 x + 1 y = 3 x 3 + 2 x + 1 over [ −1 , 1 ] [ −1 , 1 ]

[T] y = tan ( π 4 x ) y = tan ( π 4 x ) over [ − 3 2 , 3 2 ] [ − 3 2 , 3 2 ]

[T] y = x 2 cos ( π x ) y = x 2 cos ( π x ) over [ −2 , 2 ] [ −2 , 2 ]

[T] y = x 6 − 3 4 x 5 − 9 8 x 4 + 15 16 x 3 + 3 32 x 2 + 3 16 x + 1 32 y = x 6 − 3 4 x 5 − 9 8 x 4 + 15 16 x 3 + 3 32 x 2 + 3 16 x + 1 32 over [ −1 , 1 ] [ −1 , 1 ]

For the following exercises, use the Mean Value Theorem and find all points 0 < c < 2 0 < c < 2 such that f ( 2 ) − f ( 0 ) = f ′ ( c ) ( 2 − 0 ) . f ( 2 ) − f ( 0 ) = f ′ ( c ) ( 2 − 0 ) .

f ( x ) = x 3 f ( x ) = x 3

f ( x ) = sin ( π x ) f ( x ) = sin ( π x )

f ( x ) = cos ( 2 π x ) f ( x ) = cos ( 2 π x )

f ( x ) = 1 + x + x 2 f ( x ) = 1 + x + x 2

f ( x ) = ( x − 1 ) 10 f ( x ) = ( x − 1 ) 10

f ( x ) = ( x − 1 ) 9 f ( x ) = ( x − 1 ) 9

For the following exercises, show there is no c c such that f ( 1 ) − f ( −1 ) = f ′ ( c ) ( 2 ) . f ( 1 ) − f ( −1 ) = f ′ ( c ) ( 2 ) . Explain why the Mean Value Theorem does not apply over the interval [ −1 , 1 ] . [ −1 , 1 ] .

f ( x ) = | x − 1 2 | f ( x ) = | x − 1 2 |

f ( x ) = 1 x 2 f ( x ) = 1 x 2

f ( x ) = | x | f ( x ) = | x |

f ( x ) = ⌊ x ⌋ f ( x ) = ⌊ x ⌋ ( Hint : This is called the floor function and it is defined so that f ( x ) f ( x ) is the largest integer less than or equal to x . ) x . )

For the following exercises, determine whether the Mean Value Theorem applies for the functions over the given interval [ a , b ] . [ a , b ] . Justify your answer.

y = e x y = e x over [ 0 , 1 ] [ 0 , 1 ]

y = ln ( 2 x + 3 ) y = ln ( 2 x + 3 ) over [ − 3 2 , 0 ] [ − 3 2 , 0 ]

f ( x ) = tan ( 2 π x ) f ( x ) = tan ( 2 π x ) over [ 0 , 2 ] [ 0 , 2 ]

y = 9 − x 2 y = 9 − x 2 over [ −3 , 3 ] [ −3 , 3 ]

y = 1 | x + 1 | y = 1 | x + 1 | over [ 0 , 3 ] [ 0 , 3 ]

y = x 3 + 2 x + 1 y = x 3 + 2 x + 1 over [ 0 , 6 ] [ 0 , 6 ]

y = x 2 + 3 x + 2 x y = x 2 + 3 x + 2 x over [ −1 , 1 ] [ −1 , 1 ]

y = x sin ( π x ) + 1 y = x sin ( π x ) + 1 over [ 0 , 1 ] [ 0 , 1 ]

y = ln ( x + 1 ) y = ln ( x + 1 ) over [ 0 , e − 1 ] [ 0 , e − 1 ]

y = x sin ( π x ) y = x sin ( π x ) over [ 0 , 2 ] [ 0 , 2 ]

y = 5 + | x | y = 5 + | x | over [ −1 , 1 ] [ −1 , 1 ]

For the following exercises, consider the roots of the equation.

Show that the equation y = x 3 + 4 x + 16 y = x 3 + 4 x + 16 has exactly one real root. What is it?

Find the conditions for exactly one root (double root) for the equation y = x 2 + b x + c y = x 2 + b x + c

Find the conditions for y = e x − b y = e x − b to have one root. Is it possible to have more than one root?

For the following exercises, use a calculator to graph the function over the interval [ a , b ] [ a , b ] and graph the secant line from a a to b . b . Use the calculator to estimate all values of c c as guaranteed by the Mean Value Theorem. Then, find the exact value of c , c , if possible, or write the final equation and use a calculator to estimate to four digits.

[T] y = tan ( π x ) y = tan ( π x ) over [ − 1 4 , 1 4 ] [ − 1 4 , 1 4 ]

[T] y = 1 x + 1 y = 1 x + 1 over [ 0 , 3 ] [ 0 , 3 ]

[T] y = | x 2 + 2 x − 4 | y = | x 2 + 2 x − 4 | over [ −4 , 0 ] [ −4 , 0 ]

[T] y = x + 1 x y = x + 1 x over [ 1 2 , 4 ] [ 1 2 , 4 ]

[T] y = x + 1 + 1 x 2 y = x + 1 + 1 x 2 over [ 3 , 8 ] [ 3 , 8 ]

At 10:17 a.m., you pass a police car at 55 mph that is stopped on the freeway. You pass a second police car at 55 mph at 10:53 a.m., which is located 39 mi from the first police car. If the speed limit is 60 mph, can the police cite you for speeding?

Two cars drive from one stoplight to the next, leaving at the same time and arriving at the same time. Is there ever a time when they are going the same speed? Prove or disprove.

Show that y = sec 2 x y = sec 2 x and y = tan 2 x y = tan 2 x have the same derivative. What can you say about y = sec 2 x − tan 2 x ? y = sec 2 x − tan 2 x ?

Show that y = csc 2 x y = csc 2 x and y = cot 2 x y = cot 2 x have the same derivative. What can you say about y = csc 2 x − cot 2 x ? y = csc 2 x − cot 2 x ?

As an Amazon Associate we earn from qualifying purchases.

This book may not be used in the training of large language models or otherwise be ingested into large language models or generative AI offerings without OpenStax's permission.

Want to cite, share, or modify this book? This book uses the Creative Commons Attribution-NonCommercial-ShareAlike License and you must attribute OpenStax.

Access for free at https://openstax.org/books/calculus-volume-1/pages/1-introduction

• Authors: Gilbert Strang, Edwin “Jed” Herman
• Publisher/website: OpenStax
• Book title: Calculus Volume 1
• Publication date: Mar 30, 2016
• Location: Houston, Texas
• Book URL: https://openstax.org/books/calculus-volume-1/pages/1-introduction
• Section URL: https://openstax.org/books/calculus-volume-1/pages/4-4-the-mean-value-theorem

© Feb 5, 2024 OpenStax. Textbook content produced by OpenStax is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike License . The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons
• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

4.4: Rolle’s Theorem and The Mean Value Theorem

• Last updated
• Save as PDF
• Page ID 102732

Learning Objectives

• Explain the meaning of Rolle’s theorem.
• Describe the significance of the Mean Value Theorem.
• State three important consequences of the Mean Value Theorem.

The Mean Value Theorem is one of the most important theorems in calculus. We look at some of its implications at the end of this section. First, let’s start with a special case of the Mean Value Theorem, called Rolle’s theorem.

Rolle’s Theorem

Informally, Rolle’s theorem states that if the outputs of a differentiable function \(f\) are equal at the endpoints of an interval, then there must be an interior point \(c\) where \(f'(c)=0\). Figure \(\PageIndex{1}\) illustrates this theorem.

Let \(f\) be a continuous function over the closed interval \([a,b]\) and differentiable over the open interval \((a,b)\) such that \(f(a)=f(b)\). There then exists at least one \(c∈(a,b)\) such that \(f'(c)=0.\)

Let \(k=f(a)=f(b).\) We consider three cases:

• \(f(x)=k\) for all \(x∈(a,b).\)
• There exists \(x∈(a,b)\) such that \(f(x)>k.\)
• There exists \(x∈(a,b)\) such that \(f(x)<k.\)

Case 1 : If \(f(x)=k\) for all \(x∈(a,b)\), then \(f'(x)=0\) for all \(x∈(a,b).\)

Case 2 : Since \(f\) is a continuous function over the closed, bounded interval \([a,b]\), by the extreme value theorem, it has an absolute maximum. Also, since there is a point \(x∈(a,b)\) such that \(f(x)>k\), the absolute maximum is greater than \(k\). Therefore, the absolute maximum does not occur at either endpoint. As a result, the absolute maximum must occur at an interior point \(c∈(a,b)\). Because \(f\) has a maximum at an interior point \(c\), and \(f\) is differentiable at \(c\), by Fermat’s theorem, \(f'(c)=0.\)

Case 3 : The case when there exists a point \(x∈(a,b)\) such that \(f(x)<k\) is analogous to case 2, with maximum replaced by minimum.

An important point about Rolle’s theorem is that the differentiability of the function \(f\) is critical. If \(f\) is not differentiable, even at a single point, the result may not hold. For example, the function \(f(x)=|x|−1\) is continuous over \([−1,1]\) and \(f(−1)=0=f(1)\), but \(f'(c)≠0\) for any \(c∈(−1,1)\) as shown in the following figure.

Let’s now consider functions that satisfy the conditions of Rolle’s theorem and calculate explicitly the points \(c\) where \(f'(c)=0.\)

Example \(\PageIndex{1}\): Using Rolle’s Theorem

For each of the following functions, verify that the function satisfies the criteria stated in Rolle’s theorem and find all values \(c\) in the given interval where \(f'(c)=0.\)

• \(f(x)=x^2+2x\) over \([−2,0]\)
• \(f(x)=x^3−4x\) over \([−2,2]\)

a. Since \(f\) is a polynomial, it is continuous and differentiable everywhere. In addition, \(f(−2)=0=f(0).\) Therefore, \(f\) satisfies the criteria of Rolle’s theorem. We conclude that there exists at least one value \(c∈(−2,0)\) such that \(f'(c)=0\). Since \(f'(x)=2x+2=2(x+1),\) we see that \(f'(c)=2(c+1)=0\) implies \(c=−1\) as shown in the following graph.

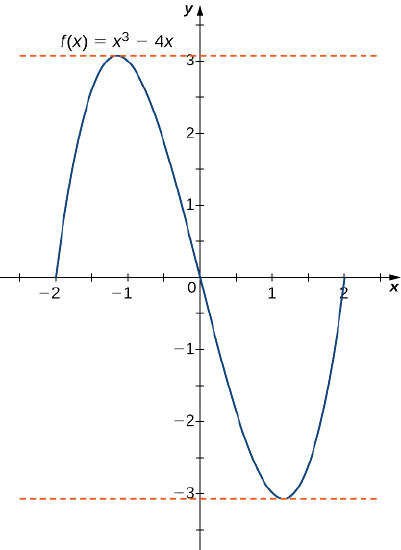
b. As in part a. \(f\) is a polynomial and therefore is continuous and differentiable everywhere. Also, \(f(−2)=0=f(2).\) That said, \(f\) satisfies the criteria of Rolle’s theorem. Differentiating, we find that \(f'(x)=3x^2−4.\) Therefore, \(f'(c)=0\) when \(x=±\frac{2}{\sqrt{3}}\). Both points are in the interval \([−2,2]\), and, therefore, both points satisfy the conclusion of Rolle’s theorem as shown in the following graph.

Exercise \(\PageIndex{1}\)

Verify that the function \(f(x)=2x^2−8x+6\) defined over the interval \([1,3]\) satisfies the conditions of Rolle’s theorem. Find all points \(c\) guaranteed by Rolle’s theorem.

Find all values \(c\), where \(f'(c)=0\).

The Mean Value Theorem and Its Meaning

Rolle’s theorem is a special case of the Mean Value Theorem. In Rolle’s theorem, we consider differentiable functions \(f\) that are zero at the endpoints. The Mean Value Theorem generalizes Rolle’s theorem by considering functions that are not necessarily zero at the endpoints. Consequently, we can view the Mean Value Theorem as a slanted version of Rolle’s theorem (Figure \(\PageIndex{5}\)). The Mean Value Theorem states that if \(f\) is continuous over the closed interval \([a,b]\) and differentiable over the open interval \((a,b)\), then there exists a point \(c∈(a,b)\) such that the tangent line to the graph of \(f\) at \(c\) is parallel to the secant line connecting \((a,f(a))\) and \((b,f(b)).\)

Mean Value Theorem

Let \(f\) be continuous over the closed interval \([a,b]\) and differentiable over the open interval \((a,b)\). Then, there exists at least one point \(c∈(a,b)\) such that

\[f'(c)=\frac{f(b)−f(a)}{b−a} \nonumber \]

The proof follows from Rolle’s theorem by introducing an appropriate function that satisfies the criteria of Rolle’s theorem. Consider the line connecting \((a,f(a))\) and \((b,f(b)).\) Since the slope of that line is

\[\frac{f(b)−f(a)}{b−a} \nonumber \]

and the line passes through the point \((a,f(a)),\) the equation of that line can be written as

\[y=\frac{f(b)−f(a)}{b−a}(x−a)+f(a). \nonumber \]

Let \(g(x)\) denote the vertical difference between the point \((x,f(x))\) and the point \((x,y)\) on that line. Therefore,

\[g(x)=f(x)−\left[\frac{f(b)−f(a)}{b−a}(x−a)+f(a)\right]. \nonumber \]

Since the graph of \(f\) intersects the secant line when \(x=a\) and \(x=b\), we see that \(g(a)=0=g(b)\). Since \(f\) is a differentiable function over \((a,b)\), \(g\) is also a differentiable function over \((a,b)\). Furthermore, since \(f\) is continuous over \([a,b], \, g\) is also continuous over \([a,b]\). Therefore, \(g\) satisfies the criteria of Rolle’s theorem. Consequently, there exists a point \(c∈(a,b)\) such that \(g'(c)=0.\) Since

\[g'(x)=f'(x)−\frac{f(b)−f(a)}{b−a}, \nonumber \]

we see that

\[g'(c)=f'(c)−\frac{f(b)−f(a)}{b−a}. \nonumber \]

Since \(g'(c)=0,\) we conclude that

\[f'(c)=\frac{f(b)−f(a)}{b−a}. \nonumber \]

In the next example, we show how the Mean Value Theorem can be applied to the function \(f(x)=\sqrt{x}\) over the interval \([0,9]\). The method is the same for other functions, although sometimes with more interesting consequences.

Example \(\PageIndex{2}\): Verifying that the Mean Value Theorem Applies

For \(f(x)=\sqrt{x}\) over the interval \([0,9]\), show that \(f\) satisfies the hypothesis of the Mean Value Theorem, and therefore there exists at least one value \(c∈(0,9)\) such that \(f′(c)\) is equal to the slope of the line connecting \((0,f(0))\) and \((9,f(9))\). Find these values \(c\) guaranteed by the Mean Value Theorem.

We know that \(f(x)=\sqrt{x}\) is continuous over \([0,9]\) and differentiable over \((0,9).\) Therefore, \(f\) satisfies the hypotheses of the Mean Value Theorem, and there must exist at least one value \(c∈(0,9)\) such that \(f′(c)\) is equal to the slope of the line connecting \((0,f(0))\) and \((9,f(9))\) (Figure \(\PageIndex{7}\)). To determine which value(s) of \(c\) are guaranteed, first calculate the derivative of \(f\). The derivative \(f′(x)=\frac{1}{(2\sqrt{x})}\). The slope of the line connecting \((0,f(0))\) and \((9,f(9))\) is given by

\[\frac{f(9)−f(0)}{9−0}=\frac{\sqrt{9}−\sqrt{0}}{9−0}=\frac{3}{9}=\frac{1}{3}. \nonumber \]

We want to find \(c\) such that \(f′(c)=\frac{1}{3}\). That is, we want to find \(c\) such that

\[\frac{1}{2\sqrt{c}}=\frac{1}{3}. \nonumber \]

Solving this equation for \(c\), we obtain \(c=\frac{9}{4}\). At this point, the slope of the tangent line equals the slope of the line joining the endpoints.

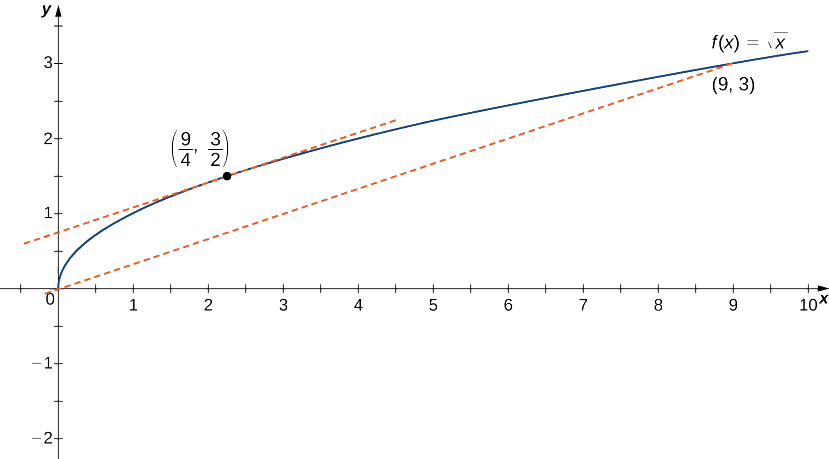
One application that helps illustrate the Mean Value Theorem involves velocity. For example, suppose we drive a car for 1 h down a straight road with an average velocity of 45 mph. Let \(s(t)\) and \(v(t)\) denote the position and velocity of the car, respectively, for \(0≤t≤1\) h. Assuming that the position function \(s(t)\) is differentiable, we can apply the Mean Value Theorem to conclude that, at some time \(c∈(0,1)\), the speed of the car was exactly

\[v(c)=s′(c)=\frac{s(1)−s(0)}{1−0}=45\,\text{mph.} \nonumber \]

Example \(\PageIndex{3}\): Mean Value Theorem and Velocity

If a rock is dropped from a height of 100 ft, its position \(t\) seconds after it is dropped until it hits the ground is given by the function \(s(t)=−16t^2+100.\)

• Determine how long it takes before the rock hits the ground.
• Find the average velocity \(v_{avg}\) of the rock for when the rock is released and the rock hits the ground.
• Find the time \(t\) guaranteed by the Mean Value Theorem when the instantaneous velocity of the rock is \(v_{avg}.\)

a. When the rock hits the ground, its position is \(s(t)=0\). Solving the equation \(−16t^2+100=0\) for \(t\), we find that \(t=±\frac{5}{2}sec\). Since we are only considering \(t≥0\), the ball will hit the ground \(\frac{5}{2}\) sec after it is dropped.

b. The average velocity is given by

\[v_{avg}=\frac{s(5/2)−s(0)}{5/2−0}=\frac{0−100}{5/2}=−40\,\text{ft/sec}. \nonumber \]

c. The instantaneous velocity is given by the derivative of the position function. Therefore, we need to find a time \(t\) such that \(v(t)=s′(t)=v_{avg}=−40\) ft/sec. Since \(s(t)\) is continuous over the interval \([0,5/2]\) and differentiable over the interval \((0,5/2),\) by the Mean Value Theorem, there is guaranteed to be a point \(c∈(0,5/2)\) such that

\[s′(c)=\frac{s(5/2)−s(0)}{5/2−0}=−40. \nonumber \]

Taking the derivative of the position function \(s(t)\), we find that \(s′(t)=−32t.\) Therefore, the equation reduces to \(s′(c)=−32c=−40.\) Solving this equation for \(c\), we have \(c=\frac{5}{4}\). Therefore, \(\frac{5}{4}\) sec after the rock is dropped, the instantaneous velocity equals the average velocity of the rock during its free fall: \(−40\) ft/sec.

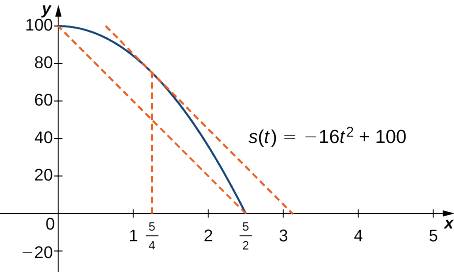
Exercise \(\PageIndex{2}\)

Suppose a ball is dropped from a height of 200 ft. Its position at time \(t\) is \(s(t)=−16t^2+200.\) Find the time \(t\) when the instantaneous velocity of the ball equals its average velocity.

First, determine how long it takes for the ball to hit the ground. Then, find the average velocity of the ball from the time it is dropped until it hits the ground.

\(\frac{5}{2\sqrt{2}}\) sec

Corollaries of the Mean Value Theorem

Let’s now look at three corollaries of the Mean Value Theorem. These results have important consequences, which we use in upcoming sections.

At this point, we know the derivative of any constant function is zero. The Mean Value Theorem allows us to conclude that the converse is also true. In particular, if \(f′(x)=0\) for all \(x\) in some interval \(I\), then \(f(x)\) is constant over that interval. This result may seem intuitively obvious, but it has important implications that are not obvious, and we discuss them shortly.

Corollary 1: Functions with a Derivative of Zero

Let \(f\) be differentiable over an interval \(I\). If \(f′(x)=0\) for all \(x∈I\), then \(f(x)=\) constant for all \(x∈I.\)

Since \(f\) is differentiable over \(I\), \(f\) must be continuous over \(I\). Suppose \(f(x)\) is not constant for all \(x\) in \(I\). Then there exist \(a,b∈I\), where \(a≠b\) and \(f(a)≠f(b).\) Choose the notation so that \(a<b.\) Therefore,

\[\frac{f(b)−f(a)}{b−a}≠0. \nonumber \]

Since \(f\) is a differentiable function, by the Mean Value Theorem, there exists \(c∈(a,b)\) such that

\[f′(c)=\frac{f(b)−f(a)}{b−a}. \nonumber \]

Therefore, there exists \(c∈I\) such that \(f′(c)≠0\), which contradicts the assumption that \(f′(x)=0\) for all \(x∈I\).

From "Corollary 1: Functions with a Derivative of Zero," it follows that if two functions have the same derivative, they differ by, at most, a constant.

Corollary 2: Constant Difference Theorem

If \(f\) and \(g\) are differentiable over an interval \(I\) and \(f′(x)=g′(x)\) for all \(x∈I\), then \(f(x)=g(x)+C\) for some constant \(C\).

Let \(h(x)=f(x)−g(x).\) Then, \(h′(x)=f′(x)−g′(x)=0\) for all \(x∈I.\) By Corollary 1, there is a constant \(C\) such that \(h(x)=C\) for all \(x∈I\). Therefore, \(f(x)=g(x)+C\) for all \(x∈I.\)

The third corollary of the Mean Value Theorem discusses when a function is increasing and when it is decreasing. Recall that a function \(f\) is increasing over \(I\) if \(f(x_1)<f(x_2)\) whenever \(x_1<x_2\), whereas \(f\) is decreasing over \(I\) if \(f(x_1)>f(x_2)\) whenever \(x_1<x_2\). Using the Mean Value Theorem, we can show that if the derivative of a function is positive, then the function is increasing; if the derivative is negative, then the function is decreasing (Figure \(\PageIndex{9}\)). We make use of this fact in the next section, where we show how to use the derivative of a function to locate local maximum and minimum values of the function, and how to determine the shape of the graph.

This fact is important because it means that for a given function \(f\), if there exists a function \(F\) such that \(F′(x)=f(x)\); then, the only other functions that have a derivative equal to \(f\) are \(F(x)+C\) for some constant \(C\). We discuss this result in more detail later in the chapter.

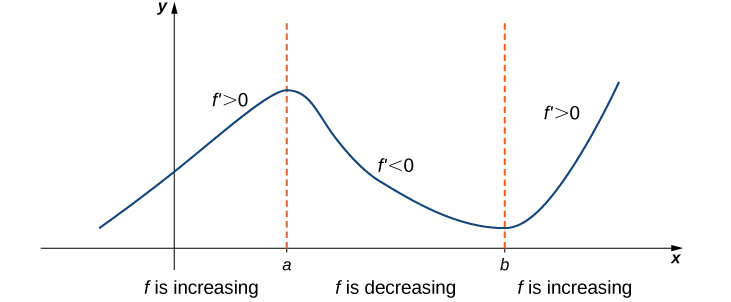
Corollary 3: Increasing and Decreasing Functions

Let \(f\) be continuous over the closed interval \([a,b]\) and differentiable over the open interval \((a,b)\).

• If \(f′(x)>0\) for all \(x∈(a,b)\), then \(f\) is an increasing function over \([a,b].\)
• If \(f′(x)<0\) for all \(x∈(a,b)\), then \(f\) is a decreasing function over \([a,b].\)

We will prove i.; the proof of ii. is similar. Suppose \(f\) is not an increasing function on \(I\). Then there exist \(a\) and \(b\) in \(I\) such that \(a<b\), but \(f(a)≥f(b)\). Since \(f\) is a differentiable function over \(I\), by the Mean Value Theorem there exists \(c∈(a,b)\) such that

Since \(f(a)≥f(b)\), we know that \(f(b)−f(a)≤0\). Also, \(a<b\) tells us that \(b−a>0.\) We conclude that

\[f′(c)=\frac{f(b)−f(a)}{b−a}≤0. \nonumber \]

However, \(f′(x)>0\) for all \(x∈I\). This is a contradiction, and therefore \(f\) must be an increasing function over \(I\).

Key Concepts

• If \(f\) is continuous over \([a,b]\) and differentiable over \((a,b)\) and \(f(a)=f(b)\), then there exists a point \(c∈(a,b)\) such that \(f′(c)=0.\) This is Rolle’s theorem.
• If \(f\) is continuous over \([a,b]\) and differentiable over \((a,b)\), then there exists a point \(c∈(a,b)\) such that \[f'(c)=\frac{f(b)−f(a)}{b−a}. \nonumber \] This is the Mean Value Theorem.
• If \(f'(x)=0\) over an interval \(I\), then \(f\) is constant over \(I\).
• If two differentiable functions \(f\) and \(g\) satisfy \(f′(x)=g′(x)\) over \(I\), then \(f(x)=g(x)+C\) for some constant \(C\).
• If \(f′(x)>0\) over an interval \(I\), then \(f\) is increasing over \(I\). If \(f′(x)<0\) over \(I\), then \(f\) is decreasing over \(I\).

if \(f\) is continuous over \([a,b]\) and differentiable over \((a,b)\), then there exists \(c∈(a,b)\) such that \(f′(c)=\frac{f(b)−f(a)}{b−a}\)