presentation about first order differential equation

If you're seeing this message, it means we're having trouble loading external resources on our website.

If you're behind a web filter, please make sure that the domains *.kastatic.org and *.kasandbox.org are unblocked.

To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

Unit 1: First order differential equations

About this unit, intro to differential equations.

• Differential equations introduction (Opens a modal)
• Writing a differential equation (Opens a modal)
• Worked example: linear solution to differential equation (Opens a modal)
• Verify solutions to differential equations 4 questions Practice
• Write differential equations 4 questions Practice
• Differential equations challenge 4 questions Practice

Slope fields

• Slope fields introduction (Opens a modal)
• Worked example: equation from slope field (Opens a modal)
• Worked example: slope field from equation (Opens a modal)
• Worked example: forming a slope field (Opens a modal)
• Approximating solution curves in slope fields (Opens a modal)
• Worked example: range of solution curve from slope field (Opens a modal)
• Slope fields & equations 4 questions Practice
• Reasoning using slope fields 4 questions Practice

Euler's Method

• Euler's method (Opens a modal)
• Worked example: Euler's method (Opens a modal)
• Euler's method 4 questions Practice

Separable equations

• Separable equations introduction (Opens a modal)
• Addressing treating differentials algebraically (Opens a modal)
• Worked example: identifying separable equations (Opens a modal)
• Worked example: finding a specific solution to a separable equation (Opens a modal)
• Worked example: separable equation with an implicit solution (Opens a modal)
• Separable equations (old) (Opens a modal)
• Separable equations example (old) (Opens a modal)
• Particular solutions to separable differential equations 4 questions Practice

Exponential models

• Exponential models & differential equations (Part 1) (Opens a modal)
• Exponential models & differential equations (Part 2) (Opens a modal)
• Worked example: exponential solution to differential equation (Opens a modal)
• Newton's Law of Cooling (Opens a modal)
• Worked example: Newton's law of cooling (Opens a modal)
• Differential equations: exponential model equations 4 questions Practice

Logistic models

• Growth models: introduction (Opens a modal)
• The logistic growth model (Opens a modal)
• Logistic equations (Part 1) (Opens a modal)
• Logistic equations (Part 2) (Opens a modal)
• Worked example: logistic model equations (Opens a modal)

Exact equations and integrating factors

• Exact equations intuition 1 (proofy) (Opens a modal)
• Exact equations intuition 2 (proofy) (Opens a modal)
• Exact equations example 1 (Opens a modal)
• Exact equations example 2 (Opens a modal)
• Exact equations example 3 (Opens a modal)
• Integrating factors 1 (Opens a modal)
• Integrating factors 2 (Opens a modal)

Homogeneous equations

• First order homogenous equations (Opens a modal)
• First order homogeneous equations 2 (Opens a modal)

Browse Course Material

Course info, instructors.

• Prof. Haynes Miller
• Prof. Arthur Mattuck

Departments

• Mathematics

As Taught In

• Differential Equations
• Linear Algebra

Learning Resource Types

Lecture notes.

Below are the lecture notes for every lecture session along with links to the Mathlets used during lectures.

You are leaving MIT OpenCourseWare

Forgot password? New user? Sign up

Existing user? Log in

First Order Linear Differential Equations

Already have an account? Log in here.

• A Former Brilliant Member
• Andrew Hayes

A first order linear differential equation is a differential equation of the form \(y'+p(x) y=q(x)\). The left-hand side of this equation looks almost like the result of using the product rule , so we solve the equation by multiplying through by a factor that will make the left-hand side exactly the result of a product rule, and then integrating. This factor is called an integrating factor.

First order linear differential equations are the only differential equations that can be solved even with variable coefficients - almost every other kind of equation that can be solved explicitly requires the coefficients to be constant, making these one of the broadest classes of differential equations that can be solved.

Integrating Factors

Solving equations, applications.

An integrating factor will be a function \(f(x)\) such that multiplying it to both sides of the equation,

\[\begin{align} f(x) \left[ y'+p(x)y \right] &= f(x)q(x) \\ \\ f(x)y'+f(x)p(x)y &= f(x)q(x), \end{align}\]

gives an expression on the left-hand side that can be integrated with the anti-product rule. In other words, it is the function that satisfies:

\[\begin{align} f(x)y'+f(x)p(x)y &= f(x)y'+f'(x)y \\ \\ \int\left[ f(x)y'+f(x)p(x)y \right]\ dx &= f(x)y+C \end{align}\]

This means the function \(f\) should satisfy \(f'(x)=f(x)p(x)\), which is a separable differential equation . This can be solved as

\[\begin{equation}\begin{split} \frac{f'(x)}{f(x)}\, dx= p(x)\, dx &\implies \int \frac{f'(x)}{f(x)}\, dx=\int p(x)\, dx \\ \\ & \implies \ln |f(x)|=\int p(x)\, dx \\ \\ &\implies f(x)= {\large e}^{\int p(x)\, dx}. \end{split}\end{equation}\]

This is the integrating factor needed to solve first order linear differential equations.

With the integrating factor, solving the equations is relatively straightforward.

Solve the differential equation \(y'+e^xy=e^x\). Here, \(p(x)=q(x)=e^x\). Our integrating factor is \[e^{\int e^x\, dx}=e^{e^x}.\] Note that we omit the constant of integration, since it would just produce a shifted solution to the equation. Now we multiply both sides by \(e^{e^x}\) to get \[e^{e^x}y'+e^{e^x}e^xy=e^xe^{e^x}\implies \left[e^{e^x}y\right]'=e^xe^{e^x},\] and now integrating both sides gives \[e^{e^x}y=e^{e^x}+C\implies y=Ce^{-e^x}+1.\]

Solve \[\dfrac{dy}{dx} - 3y = e^{2x}, \quad y(0) = 3\] \[v(x) = e^{-3x} \Rightarrow e^{-3x} \dfrac{dy}{dx} - 3e^{-3x}y = e^{-x} \Rightarrow\] \[\dfrac{d}{dx} \left(e^{-3x}y\right) = e^{-x} \Rightarrow\] \[e^{-3x}y = -e^{-x} + C \Rightarrow y(x) = Ce^{3x} - e^{2x}\] along the condition \(y(0) = 3\) we get \(C = 4\) so the function is \[y(x) = 4e^{3x} - e^{2x}\]

Solve \[\begin{cases} ty' +(2t +1)y =te^{-2t},\quad t \in [1,\infty)\\ y(1) = 0 \end{cases}\] \[y' + \frac{2t +1}{t}y = e^{-2t} \rightarrow v(t) = te^{2t} \rightarrow \dfrac{d}{dt} (te^{2t}y) = t \Rightarrow\] \[y(t) =\frac{1}{t}e^{-2t}\left(\frac{t^2}{2} + C\right),\quad y(1) = 0 \Rightarrow C = \frac{-1}{2} \Rightarrow\] \[y(t) = \frac{1}{2}\left(t -\frac{1}{t}\right)e^{-2t}\]

Suppose that \(y\) satisfies the equation \(y'+y\tan x=\sec x.\) \(y\left (\dfrac{\pi}{4}\right) = \dfrac{1}{\sqrt{2}}. \)

What is the value of \(y\left (\dfrac{\pi}{2}\right)?\)

Many physical systems can be described by first order linear differential equations.

Suppose there is a circuit with a switch, a battery, a resistor, and an inductor in series. The battery produces a voltage of 45 volts and a current of \(I(t\) amperes at time \(t\). The resistor is an \(9\) ohm resistor and the inductor has an inductance of \(3\) henries. If the switch is initially closed, what is the current in the circuit a long time after the switch has been opened? Ohm's law says that the voltage drop due to the resistor is \(9I(t) \) volts. The voltage drop from the inductor is \(3 I'(t)\). Kirchoff's laws say that the sum of the voltage drops is equal to the supplied voltage, so we find \[ 3I'(t)+9I(t)=45\implies I'(t)+3I(t)=15.\] The integrating factor is \(e^{\int 3\, dt}=e^{3t}\), and multiplying through by this gives \[I'(t)e^{3t}+3e^{3t}I'(t)=15e^{3t}\implies \left[I(t)e^{3t}\right]'=15e^{3t}\implies I(t)e^{3t}=5e^{3t}+C\implies I(t)=Ce^{-3t}+5.\] As \(t\to\infty\), we see that \(I(t)\to 5\), so the current after a long time is 5 amperes.

Problem Loading...

Note Loading...

Set Loading...

Academia.edu no longer supports Internet Explorer.

To browse Academia.edu and the wider internet faster and more securely, please take a few seconds to  upgrade your browser .

Enter the email address you signed up with and we'll email you a reset link.

• We're Hiring!
• Help Center

First-Order Differential Equations PPT

Related Papers

kobie de dion Gomez

Astera ab. Jabar

Melkamu Baza

Jessica R PT

Arslan Shakeel

1. Second order; linear 2. Third order; nonlinear because of (dy/dx) 4 3. Fourth order; linear 4. Second order; nonlinear because of cos(r + u) 5. Second order; nonlinear because of (dy/dx) 2 or 1 + (dy/dx) 2 6. Second order; nonlinear because of R 2 7. Third order; linear 8. Second order; nonlinear because of ˙ x 2 9. Writing the differential equation in the form x(dy/dx) + y 2 = 1, we see that it is nonlinear in y because of y 2. However, writing it in the form (y 2 − 1)(dx/dy) + x = 0, we see that it is linear in x. 10. Writing the differential equation in the form u(dv/du) + (1 + u)v = ue u we see that it is linear in v. However, writing it in the form (v + uv − ue u)(du/dv) + u = 0, we see that it is nonlinear in u. 11. From y = e −x/2 we obtain y = − 1 2 e −x/2. Then 2y + y = −e −x/2 + e −x/2 = 0. 12. From y = 6 5 − 6 5 e −20t we obtain dy/dt = 24e −20t , so that dy dt + 20y = 24e −20t + 20 6 5 − 6 5 e −20t = 24. 13. From y = e 3x cos 2x we obtain y = 3e 3x cos 2x − 2e 3x sin 2x and y = 5e 3x cos 2x − 12e 3x sin 2x, so that y − 6y + 13y = 0. 14. From y = − cos x ln(sec x + tan x) we obtain y = −1 + sin x ln(sec x + tan x) and y = tan x + cos x ln(sec x + tan x). Then y + y = tan x. 15. The domain of the function, found by solving x + 2 ≥ 0, is [−2, ∞). From y = 1 + 2(x + 2) −1/2 we have (y − x)y = (y − x)[1 + (2(x + 2) −1/2 ] = y − x + 2(y − x)(x + 2) −1/2 = y − x + 2[x + 4(x + 2) 1/2 − x](x + 2) −1/2 = y − x + 8(x + 2) 1/2 (x + 2) −1/2 = y − x + 8.

Seon Jae Jin

We discuss types of differential equations, examples and solutions.

Loading Preview

Sorry, preview is currently unavailable. You can download the paper by clicking the button above.

RELATED PAPERS

Revista Investigaciones Turísticas

Margarita Capdepón Frías

Revista (Entre Parênteses)

Maria Elizabeth Bueno de Godoy

Australian Journal of Crop Science

Paulo Márcio Fernandes Viana

Journal of Children's Rights and Education

Halis Sakız

John Hodges

Ethan Katsh

Elucidario: Seminario bio-bibliográfico …

Encarnación Medina Arjona

Dentino : Jurnal Kedokteran Gigi

RENIE KUMALA DEWI

Stipe Kutleša

Manuela GARAU

J Am Acad Dermatol

Julie Charles

The Astrophysical Journal

Geoffrey Marcy

Preparative Biochemistry & Biotechnology

siddhi patil

The Open Public Health Journal

Razia Gaida

Archives of Medical Science

hande karpuzoglu

Engenharia Agrícola

Renato Lara de Assis

Heterotopías

Renata Defelice

Dyslexi Aktuellt Om Las Och Skrivsvarigheter

Ann-Katrin Swärd

Environmental Research

Melania Gaggini

DOAJ (DOAJ: Directory of Open Access Journals)

Mohamed Yaya Soumah

Global Journal of Politics and Law Research

DESMOND AGWOR

Cancer Studies and Molecular Medicine – Open Journal

Zhengyan Huang

BioMed Research International

Serena Trapasso

Justin Xavier Carteret, DMA

Lsa77 Ryerso毕业证

See More Documents Like This

•   We're Hiring!
•   Help Center
• Find new research papers in:
• Health Sciences
• Earth Sciences
• Cognitive Science
• Mathematics
• Computer Science
• Academia ©2024

• Preferences

First-Order Differential Equations - PowerPoint PPT Presentation

First-Order Differential Equations

Chapter 2 first-order differential equations contents 2.1 solution curves without a solution 2.2 separable variables 2.3 linear equations 2.4 exact equations 2.5 ... – powerpoint ppt presentation.

• 2.1 Solution Curves Without a Solution
• 2.2 Separable Variables
• 2.3 Linear Equations
• 2.4 Exact Equations
• 2.5 Solutions by Substitutions
• 2.6 A Numerical Methods
• 2.7 Linear Models
• 2.8 Nonlinear Model
• 2.9 Modeling with Systems of First-Order DEs
• Introduction Begin our study of first-order DE with analyzing a DE qualitatively.
• SlopeA derivative dy/dx of y y(x) gives slopes of tangent lines at points.
• Lineal ElementAssume dy/dx f(x, y(x)). The value f(x, y) represents the slope of a line, or a line element is called a lineal element. See Fig2.1
• If we evaluate f over a rectangular grid of points, and draw a lineal element at each point (x, y) of the grid with slope f(x, y), then the collection is called a direction field or a slope field of the following DE dy/dx f(x, y)
• The direction field of dy/dx 0.2xy is shown in Fig2.2(a) and for comparison with Fig2.2(a), some representative graphs of this family are shown in Fig2.2(b).
• Use a direction field to draw an approximate solution curve for dy/dx sin y, y(0) -3/2.
• SolutionRecall from the continuity of f(x, y) and ?f/?y cos y. Theorem 2.1 guarantees the existence of a unique solution curve passing any specified points in the plane. Now split the region containing (-3/2, 0) into grids. We calculate the lineal element of each grid to obtain Fig2.3.
• Increasing/DecreasingIf dy/dx gt 0 for all x in I, then y(x) is increasing in I.If dy/dx lt 0 for all x in I, then y(x) is decreasing in I.
• DEs Free of the Independent variable dy/dx f(y) (1)is called autonomous. We shall assume f and f ? are continuous on some I.
• The zeros of f in (1) are important. If f(c) 0, then c is a critical point, equilibrium point or stationary point.Substitute y(x) c into (1), then we have 0 f(c) 0.
• If c is a critical point, then y(x) c, is a solution of (1).
• A constant solution y(x) c of (1) is called an equilibrium solution.
• The following DE dP/dt P(a bP)
• where a and b are positive constants, is autonomous.From f(P) P(a bP) 0, the equilibrium solutions are P(t) 0 and P(t) a/b.
• Put the critical points on a vertical line. The arrows in Fig 2.4 indicate the algebraic sign of f(P) P(a bP). If the sign is positive or negative, then P is increasing or decreasing on that interval.
• If we guarantee the existence and uniqueness of (1), through any point (x0, y0) in R, there is only one solution curve. See Fig 2.5(a).
• Suppose (1) possesses exactly two critical points, c1, and c2, where c1 lt c2. The graph of the equilibrium solution y(x) c1, y(x) c2 are horizontal lines and split R into three regions, say R1, R2 and R3 as in Fig 2.5(b).
• Some discussions without proof
• (1) If (x0, y0) in Ri, i 1, 2, 3, when y(x) passes through (x0, y0), will remain in the same subregion. See Fig 2.5(b).
• (2) By continuity of f , f(y) can not change signs in a subregion.
• (3) Since dy/dx f(y(x)) is either positive or negative in Ri, a solution y(x) is monotonic.
• (4)If y(x) is bounded above by c1, (y(x) lt c1), the graph of y(x) will approach y(x) c1If c1 lt y(x) lt c2, it will approach y(x) c1 and y(x) c2If c2 lt y(x) , it will approach y(x) c2
• Referring to example 3, P 0 and P a/b are two critical points, so we have three intervals for P R1 (-?, 0), R2 (0, a/b), R3 (a/b, ?) Let P(0) P0 and when a solution pass through P0, we have three kind of graph according to the interval where P0 lies on. See Fig 2.6.
• The DE dy/dx (y 1)2 possesses the single critical point 1. From Fig 2.7(a), we conclude a solution y(x) is increasing in -? lt y lt 1 and 1 lt y lt ?, where -? lt x lt ?. See Fig 2.7.
• See Fig 2.8(a). When y0 lies on either side of c, it will approach c. This kind of critical point is said to be asymptotically stable, also called an attractor.
• See Fig 2.8(b). When y0 lies on either side of c, it will move away from c. This kind of critical point is said to be unstable, also called a repeller.
• See Fig 2.8(c) and (d). When y0 lies on one side of c, it will be attracted to c and repelled from the other side. This kind of critical point is said to be semi-stable.
• Fig 2.9 shows the direction field of dy/dx 2y 2.It can be seen that lineal elements passing through points on any horizontal line must have the same slope. Since the DE has the form dy/dx f(y), the slope depends only on y.
• Introduction Consider dy/dx f(x, y) g(x). The DE dy/dx g(x) (1)can be solved by integration. Integrating both sides to get y ? g(x) dx G(x) c.eg dy/dx 1 e2x, then y ? (1 e2x) dx x ½ e2x c
• Rewrite the above equation as (2)where p(y) 1/h(y). When h(y) 1, (2) reduces to (1).
• If y ?(x) is a solution of (2), we must have and (3)But dy ? ?(x) dx, (3) is the same as (4)
• Solve (1 x) dy y dx 0.
• SolutionSince dy/y dx/(1 x), we have Replacing by c, gives y c(1 x).
• Solution We also can rewrite the solution as x2 y2 c2, where c2 2c1Apply the initial condition, 16 9 25 c2See Fig2.18.
• When r is a zero of h(y), then y r is also a solution of dy/dx g(x)h(y). However, this solution will not show up after integration. That is a singular solution.
• Solve dy/dx y2 4.
• SolutionRewrite this DE as (5)then
• Replacing exp(c2) by c and solving for y, we have (6)If we rewrite the DE as dy/dx (y 2)(y 2), from the previous discussion, we have y ? 2 is a singular solution.
• SolutionRewrite this DE as using sin 2x 2 sin x cos x, then ? (ey ye-y) dy 2 ? sin x dx from integration by parts, ey ye-y e-y -2 cos x c (7)From y(0) 0, we have c 4 to get ey ye-y e-y 4 -2 cos x (8)
• Let G(x, y) ey ye-y e-y 2 cos x. Using some computer software, we plot the level curves of G(x, y) c. The resulting graphs are shown in Fig2.19 and Fig2.20.
• If we solve dy/dx xy½ , y(0) 0 (9)The resulting graphs are shown in Fig2.21.
• Introduction Linear DEs are friendly to be solved. We can find some smooth methods to deal with.
• Standard FormThe standard for of a first-order DE can be written as dy/dx P(x)y f(x) (2)
• The PropertyDE (2) has the property that its solution is sum of two solutions, y yc yp, where yc is a solution of the homogeneous equation dy/dx P(x)y 0 (3)and yp is a particular solution of (2).
• Verification Now (3) is also separable. Rewrite (3) as
• Solving for y gives
• Let yp u(x) y1(x), where y1(x) is defined as above.We want to find u(x) so that yp is also a solution. Substituting yp into (2) gives
• Since dy1/dx P(x)y1 0, so that y1(du/dx) f(x) Rearrange the above equation, From the definition of y1(x), we have (4)
• If (4) is multiplied by (5)then ( 6)is differentiated (7)we get (8)Dividing (8) by gives (2).
• We call y1(x) is an integrating factor and we should only memorize this to solve problems.
• Solve dy/dx 3y 6.
• SolutionSince P(x) 3, we have the integrating factor is then is the same as So e-3xy -2e-3x c, a solution is y -2 ce-3x, -? lt x lt ?.
• The DE of example 1 can be written as so that y 2 is a critical point.
• Equation (4) is called the general solution on some interval I. Suppose again P and f are continuous on I. Writing (2) as Suppose again P and f are continuous on I. Writing (2) as y? F(x, y) we identify F(x, y) P(x)y f(x), ?F/?y P(x)which are continuous on I.Then we can conclude that there exists one and only one solution of (9)
• SolutionDividing both sides by x, we have (10)So, P(x) 4/x, f(x) x5ex, P and f are continuous on (0, ?).Since x gt 0, we write the integrating factor as
• Multiply (10) by x-4, Using integration by parts, it follows that the general solution on (0, ?) is x-4y xex ex c or y x5ex x4ex cx4
• Find the general solution of
• SolutionRewrite as (11)So, P(x) x/(x2 9). Though P(x) is continuous on (-?, -3), (-3, 3) and (3, ?), we shall solve this DE on the first and third intervals. The integrating factor is
• then multiply (11) by this factor to get and Thus, either for x gt 3 or x lt -3, the general solution is
• Notes x 3 and x -3 are singular points of the DE and is discontinuous at these points.
• SolutionWe first have P(x) 1 and f(x) x, and are continuous on (-?, ?). The integrating factor is , so gives exy xex ex c and y x 1 ce-xSince y(0) 4, then c 5. The solution is y x 1 5e-x, ? lt x lt ? (12)
• Notes From the above example, we find yc ce-x and yp x 1 we call yc is a transient term, since yc ? 0 as x? ?.Some solutions are shown in Fig2.24.
• Solve , where
• SolutionFirst we see the graph of f(x) in Fig2.25.
• We solve this problem on 0 ? x ? 1 and 1 lt x lt ?.For 0 ? x ? 1, then y 1 c1e-xSince y(0) 0, c1 -1, y 1 - e-xFor x gt 1, dy/dx y 0 then y c2e-x
• We haveFurthermore, we want y(x) is continuous at x 1, that is, when x ? 1, y(x) y(1) implies c2 e 1.As in Fig2.26, the function (13)are continuous on 0, ?).
• We are interested in the error function and complementary error function and (14)Since , we find erf(x) erfc(x) 1
• Solve dy/dx 2xy 2, y(0) 1.
• SolutionWe find the integrating factor is exp-x2, we get (15)Applying y(0) 1, we have c 1. See Fig2.27
• Introduction Though ydx xdy 0 is separable, we can solve it in an alternative way to get the implicit solution xy c.
• If z f(x, y), its differential or total differential is (1)Now if z f(x, y) c, (2)eg if x2 5xy y3 c, then (2) gives (2x 5y) dx (-5x 3y2) dy 0 (3)
• If M(x, y) dx N(x, y) dy is exact, there exists some function f such that for all x in R M(x, y) dx N(x, y) dy (?f/?x) dx (?f/?y) dyTherefore M(x, y) , N(x, y) and The sufficient part consists of showing that there is a function f for which M(x, y) and N(x, y)
• Since ?f/?x M(x, y), we have (5)Differentiating (5) with respect to y and assume ?f/?y N(x, y)Then and (6)
• Integrate (6) with respect to y to get g(y), and substitute the result into (5) to obtain the implicit solution f(x, y) c.
• Solve 2xy dx (x2 1) dy 0.
• SolutionWith M(x, y) 2xy, N(x, y) x2 1, we have ?M/?y 2x ?N/?xThus it is exact. There exists a function f such that ?f/?x 2xy, ?f/?y x2 1Then f(x, y) x2y g(y) ?f/?y x2 g(y) x2 1 g(y) -1, g(y) -y
• Hence f(x, y) x2y y, and the solution is x2y y c, y c/(1 x2)The interval of definition is any interval not containing x 1 or x -1.
• Solve (e2y y cos xy)dx(2xe2y x cos xy 2y)dy 0.
• SolutionThis DE is exact because ?M/?y 2e2y xy sin xy cos xy ?N/?xHence a function f exists, and ?f/?y 2xe2y x cos xy 2ythat is,
• Thus h(x) 0, h(x) c. The solution is xe2y sin xy y2 c 0
• SolutionRewrite the DE in the form (cos x sin x xy2) dx y(1 x2) dy 0 Since ?M/?y 2xy ?N/?x (This DE is exact)Now ?f/?y y(1 x2) f(x, y) ½y2(1 x2) h(x) ?f/?x xy2 h(x) cos x sin x xy2
• We have h?(x) cos x sin x h(x) -½ cos2 xThus ½y2(1 x2) ½ cos2 x c1 or y2(1 x2) cos2 x c (7)where c 2c1. Now y(0) 2, so c 3.The solution is y2(1 x2) cos2 x 3
• Fig 2.28 shows the family curves of the above example and the curve of the specialized VIP is drawn in color.
• It is sometimes possible to find an integrating factor?(x, y), such that ?(x, y)M(x, y)dx ?(x, y)N(x, y)dy 0 (8)is an exact differential.Equation (8) is exact if and only if (?M)y (?N)x Then ?My ?yM ?Nx ?xN, or ?xN ?yM (My Nx) ? (9)
• Suppose ? is a function of one variable, say x, then ?x d? /dx(9) becomes (10)If we have (My Nx) / N depends only on x, then (10) is a first-order ODE and is separable. Similarly, if ? is a function of y only, then (11)In this case, if (Nx My) / M is a function of y only, then we can solve (11) for ?.
• We summarize the results for M(x, y) dx N(x, y) dy 0 (12)If (My Nx) / N depends only on x, then (13)If (Nx My) / M depends only on y, then (14)
• The nonlinear DE xy dx (2x2 3y2 20) dy 0 is not exact. With M xy, N 2x2 3y2 20, we find My x, Nx 4x. Since depends on both x and y. depends only on y.The integrating factor is e ? 3dy/y e3lny y3 ?(y)
• then the resulting equation is xy4 dx (2x2y3 3y5 20y3) dy 0It is left to you to verify the solution is ½ x2y4 ½ y6 5y4 c
• IntroductionIf we want to transform the first-order DE dx/dy f(x, y)by the substitution y g(x, u), where u is a function of x, then Since dy/dx f(x, y), y g(x, u), Solving for du/dx, we have the form du/dx F(x, u). If we can get u ?(x), a solution is y g(x, ?(x)).
• If a function f has the property f(tx, ty) t?f(x, y), then f is called a homogeneous function of degree ?.eg f(x, y) x3 y3 is homogeneous of degree 3, f(tx, ty) (tx)3 (ty)3 t3f(x, y)
• A first-order DE M(x, y) dx N(x, y) dy 0 (1)is said to be homogeneous, if both M and N are homogeneous of the same degree, that is, if M(tx, ty) t?M(x, y), N(tx, ty) t?N(x, y)
• Note Here the word homogeneous is not the same as in Sec 2.3.
• If M and N are homogeneous of degree ?, M(x, y)x? M(1, u), N(x, y)x?N(1, u), uy/x (2)M(x, y)y? M(v, 1), N(x, y)y?N(v, 1), vx/y (3)Then (1) becomes x? M(1, u) dx x? N(1, u) dy 0, or M(1, u) dx N(1, u) dy 0where u y/x or y ux and dy udx xdu,
• then M(1, u) dx N(1, u)(u dx x du) 0, and M(1, u) u N(1, u) dx xN(1, u) du 0or
• Solve (x2 y2) dx (x2 xy) dy 0.
• Solution We have M x2 y2, N x2 xy are homogeneous of degree 2. Let y ux, dy u dx x du, then (x2 u2x2) dx (x2 - ux2)(u dx x du) 0
• Then Simplify to get
• Note We may also try x vy.
• The DE dy/dx P(x)y f(x)yn (4)where n is any real number, is called Bernoullis Equation.
• Note for n 0 and n 1, (4) is linear, otherwise, let u y1-n to reduce (4) to a linear equation.
• Solve x dy/dx y x2y2.
• SolutionRewrite the DE as dy/dx (1/x)y xy2With n 2, then y u-1, and dy/dx -u-2(du/dx) From the substitution and simplification, du/dx (1/x)u -xThe integrating factor on (0, ?) is
• Integrating gives x-1u -x c, or u -x2 cx. Since u y-1, we have y 1/u and a solution of the DE is y 1/(-x2 cx).
• A DE of the form dy/dx f(Ax By C) (5)can always be reduced to a separable equation by means of substitution u Ax By C.
• Solve dy/dx (-2x y)2 7, y(0) 0.
• SolutionLet u -2x y, then du/dx -2 dy/dx, du/dx 2 u2 7 or du/dx u2 9This is separable. Using partial fractions, or
• then we have or Solving the equation for u and the solution is or (6)Applying y(0) 0 gives c -1.
• The graph of the particular solution is shown in Fig 2.30 in solid color.
• Using the Tangent LineLet us assume y f(x, y), y(x0) y0 (1)possess a solution. For example, the resulting graph is shown in Fig 2.31.
• Using the linearization of the unknown solution y(x) of (1) at x0, L(x) f(x0, y0)(x - x0) y0 (2)Replacing x by x1 x0 h, we have L(x1) f(x0, y0)(x0 h - x0) y0 or y1 y0 h f(x0, y0)and yn1 yn h f(xn, yn) (3)where xn x0 nh. See Fig 2.32
• Consider Use Eulers method to obtain y(2.5) using h 0.1 and then h 0.05.
• SolutionLet the results step by step are shown in Table 2.1 and table 2.2.
• Consider y 0.2xy, y(1) 1. Use Eulers method to obtain y(1.5) using h 0.1 and then h 0.05.
• SolutionWe have f(x, y) 0.2xy, the results step by step are shown in Table 2.3 and table 2.4.
• See Fig 2.33 to know the comparisons of numerical methods.
• When the result is not helpful by numerical solvers, as in Fig 2.34, we may decrease the step size, use another method, or use another solver.
• Growth and Decay (1)
• P0 initial number of bacterial P(0)P(1) 3/2 P(0)Find the time necessary for triple number.
• SolutionSince dP/dt kt, dP/dt kt 0, we have P(t) cekt, using P(0) P0then c P0 and P(t) P0ekt Since P(1) 3/2 P(0), then P(1) P0ek 3/2 P(0)So, k ln(3/2) 0.4055.Now P(t) P0e0.4055t 3P0 , t ln3/0.4055 2.71.See Fig 2.35.
• k gt 0 is called a growth constant, and k gt 0 is called a decay constant. See Fig 2.36.
• A reactor converts U-238 into the isotope plutonium-239. After 15 years, there is 0.043 of the initial amount A0 of the plutonium has disintegrated. Find the half-life of this isotope.
• SolutionLet A(t) denote the amount of plutonium remaining at time t. The DE is as (2)The solution is A(t) A0ekt. If 0.043 of A0 has disintegrated, then 99.957 remains.
• Then, 0.99957A0 A(15) A0e15k, then k (ln 0.99957) / 15 -0.00002867 Let A(t) A0e-0.00002867t ½ A0Then
• A fossilized bone contains 1/1000 the original amount C-14. Determine the age of the fossil.
• SolutionWe know the half-life of C-14 is 5600 years.Then A0 /2 A0e5600k, k -(ln 2)/5600 -0.00012378.And A(t) A0 /1000 A0e -0.00012378t
• (3)where Tm is the temperature of the medium around the object.
• A cakes temperature is 300?F. Three minutes later its temperature is 200?F. How long will it for this cake to cool off to a room temperature of 70?F?
• SolutionWe identify Tm 70, then (4)a nd T(3) 200. From (4), we have
• Using T(0) 300 then c2 230Using T(3) 200 then e3k 13/23, k -0.19018Thus T(t) 70 230e-0.19018t (5)From (5), only t ?, T(t) 70. It means we need a reasonably long time to get T 70. See Fig 2.37.
• Recall from example 5 of Sec 1.3, we have How much salt is in the tank after along time?
• SolutionSince Using x(0) 50, we have x(t) 600 - 550e-t/100 (7)When t is large enough, x(t) 600.
• See Fig 2.39. (8)See Fig 2.40. (9) (10)
• Refer to Fig 2.39, where E(t) 12 Volt, L ½ HenryR 10 Ohms. Determine i(t) where i(0) 0.
• SolutionFrom (8), Then Using i(0) 0, c -6/5, then i(t) (6/5) (6/5)e-20t.
• A general solution of (8) is (11)When E(t) E0 is a constant, (11) becomes (12)where the first term is called a steady-state part, and the second term is a transient term.
• Referring to example 1, P(t) is a continuous function. However, it should be discrete. Keeping in mind, a mathematical model is not reality. See Fig 2.41.
• Population DynamicsIf P(t) denotes the size of population at t, the relative (or specific), growth rate is defined by (1)When a population growth rate depends on the present number , the DE is (2)which is called density-dependent hypothesis.
• If K is the carrying capacity, from (2) we have f(K) 0, and simply set f(0) r. Fig 2.46 shows three functions that satisfy these two conditions.
• Suppose f (P) c1P c2. Using the conditions, we have c2 r, c1 -r/K. Then (2) becomes (3)Relabel (3), then (4)whi ch is known as a logistic equation, its solution is called the logistic function and its graph is called a logistic curve.
• From After simplification, we have
• If P(0) P0 ? a/b, then c1 P0/(a bP0) (5)
• Form (5), we have the graph as in Fig 2.47. When 0 lt P0 lt a/2b, see Fig 2.47(a).When a/2b lt P0 lt a/b, see Fig 2.47(b).
• Form the previous discussion, assume an isolated campus of 1000 students, then we have the DE Determine x(6).
• SolutionIdentify a 1000k, b k, from (5)
• Since x(4) 50, then -1000k -0.9906, Thus x(t) 1000/(1 999e-0.9906t)
• See Fig 2.48.
• or (6)or (7)which is known as the Gompertz DE.
• The chemical reaction is described as Then By separation of variables and partial fractions, (10)Using X(10) 30, 210k 0.1258, finally (11)See Fig 2.49.
• Systems (1)where g1 and g2 are linear in x and y.
• Radioactive Decay Series (2)
• From Fig 2.52, we have (3)
• Let x, y denote the fox and rabbit populations at t.When lacking of food, dx/dt ax, a gt 0 (4)When rabbits are present, dx/dt ax bxy (5)When lacking of foxes, dy/dt dy, d gt 0 (6) When foxes are present, dy/dt dy cxy (7)
• Then (8)which is known as the Lotka-Volterra predator-prey model.
• Suppose Figure 2.53 shows the graph of the solution.
• dx/dt ax, dy/dt cy (9)Two species compete, then dx/dt ax by dy/dt cy dx (10)or dx/dt ax bxy dy/dt cy dxy (11)or dx/dt a1x b1x2 dy/dt a2y b2y2 (12)
• or dx/dt a1x b1x2 c1xy dy/dt a2y b2y2 c2xy (13)
• Referring to Fig 2.54, we have i1(t) i2(t) i3(t) (14) (15) (16)
• Using (14) to eliminate i1, then (17) Referring to Fig 2.55, please verify (18)

PowerShow.com is a leading presentation sharing website. It has millions of presentations already uploaded and available with 1,000s more being uploaded by its users every day. Whatever your area of interest, here you’ll be able to find and view presentations you’ll love and possibly download. And, best of all, it is completely free and easy to use.

You might even have a presentation you’d like to share with others. If so, just upload it to PowerShow.com. We’ll convert it to an HTML5 slideshow that includes all the media types you’ve already added: audio, video, music, pictures, animations and transition effects. Then you can share it with your target audience as well as PowerShow.com’s millions of monthly visitors. And, again, it’s all free.

About the Developers

PowerShow.com is brought to you by  CrystalGraphics , the award-winning developer and market-leading publisher of rich-media enhancement products for presentations. Our product offerings include millions of PowerPoint templates, diagrams, animated 3D characters and more.

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons
• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

17.2: First Order Homogeneous Linear Equations

• Last updated
• Save as PDF
• Page ID 4842

• David Guichard
• Whitman College

A simple, but important and useful, type of separable equation is the first order homogeneous linear equation :

Definition: first order homogeneous linear differential equation

A first order homogeneous linear differential equation is one of the form

\[\dot y + p(t)y=0\]

or equivalently

\[\dot y = -p(t)y.\]

"Linear'' in this definition indicates that both \(\dot y\) and \(y\) occur to the first power; "homogeneous'' refers to the zero on the right hand side of the first form of the equation.

Example \(\PageIndex{2}\)

The equation \(\dot y = 2t(25-y)\) can be written \(\dot y + 2ty= 50t\). This is linear, but not homogeneous. The equation \(\dot y=ky\), or \(\dot y-ky=0\) is linear and homogeneous, with a particularly simple \(p(t)=-k\).

Because first order homogeneous linear equations are separable, we can solve them in the usual way:

\[\eqalign{ \dot y &= -p(t)y\cr \int {1\over y}\,dy &= \int -p(t)\,dt\cr \ln|y| &= P(t)+C\cr y&=\pm\,e^{P(t)}\cr y&=Ae^{P(t)},\cr} \]

where \(P(t)\) is an anti-derivative of \(-p(t)\). As in previous examples, if we allow \(A=0\) we get the constant solution \(y=0\).

Example \(\PageIndex{3}\)

Solve the initial value problems \(\dot y + y\cos t =0\), \(y(0)=1/2\) and \(y(2)=1/2\).

We start with

\[P(t)=\int -\cos t\,dt = -\sin t,\]

so the general solution to the differential equation is

\[y=Ae^{-\sin t}.\]

To compute \(A\) we substitute:

\[ {1\over 2} = Ae^{-\sin 0} = A,\]

so the solutions is

\[ y = {1\over 2} e^{-\sin t}.\]

For the second problem,

\[ \eqalign{{1\over 2} &= Ae^{-\sin 2}\cr A &= {1\over 2}e^{\sin 2}\cr}\]

so the solution is

\[ y = {1\over 2}e^{\sin 2}e^{-\sin t}.\]

Example \(\PageIndex{4}\)

Solve the initial value problem \(y\dot y+3y=0\), \(y(1)=2\), assuming \(t>0\).

We write the equation in standard form: \(\dot y+3y/t=0\). Then

\[P(t)=\int -{3\over t}\,dt=-3\ln t\]

\[ y=Ae^{-3\ln t}=At^{-3}.\]

Substituting to find \(A\): \(2=A(1)^{-3}=A\), so the solution is \(y=2t^{-3}\).

Contributors

First Order Linear Differential Equations

You might like to read about Differential Equations and Separation of Variables first!

A Differential Equation is an equation with a function and one or more of its derivatives :

Here we will look at solving a special class of Differential Equations called First Order Linear Differential Equations

First Order

They are "First Order" when there is only dy dx , not d 2 y dx 2 or d 3 y dx 3 etc

A first order differential equation is linear when it can be made to look like this:

dy dx + P(x)y = Q(x)

Where P(x) and Q(x) are functions of x.

To solve it there is a special method:

• We invent two new functions of x, call them u and v , and say that y=uv .
• We then solve to find u , and then find v , and tidy up and we are done!

And we also use the derivative of y=uv (see Derivative Rules (Product Rule) ):

dy dx = u dv dx + v du dx

Here is a step-by-step method for solving them:

• 2. Factor the parts involving v
• 3. Put the v term equal to zero (this gives a differential equation in u and x which can be solved in the next step)
• 4. Solve using separation of variables to find u
• 5. Substitute u back into the equation we got at step 2
• 6. Solve that to find v
• 7. Finally, substitute u and v into y = uv to get our solution!

Let's try an example to see:

Example 1: Solve this:   dy dx − y x = 1

First, is this linear? Yes, as it is in the form

dy dx + P(x)y = Q(x) where P(x) = − 1 x and Q(x) = 1

So let's follow the steps:

Step 1: Substitute y = uv , and   dy dx = u dv dx + v du dx

Step 2: Factor the parts involving v

Step 3: Put the v term equal to zero

Step 4: Solve using separation of variables to find u

Step 5: Substitute u back into the equation at Step 2

Step 6: Solve this to find v

Step 7: Substitute into y = uv to find the solution to the original equation.

And it produces this nice family of curves:

What is the meaning of those curves?

They are the solution to the equation   dy dx − y x = 1

In other words:

Anywhere on any of those curves the slope minus y x equals 1

Let's check a few points on the c=0.6 curve:

Estmating off the graph (to 1 decimal place):

Why not test a few points yourself? You can plot the curve here .

Perhaps another example to help you? Maybe a little harder?

Example 2: Solve this:   dy dx − 3y x = x

dy dx + P(x)y = Q(x) where P(x) = − 3 x and Q(x) = x

And one more example, this time even harder :

Example 3: Solve this:

  dy dx + 2xy= −2x 3

dy dx + P(x)y = Q(x) where P(x) = 2x and Q(x) = −2x 3

Let's see ... we can integrate by parts ... which says:

∫ RS dx = R ∫ S dx − ∫ R' ( ∫ S dx ) dx

(Side Note: we use R and S here, using u and v could be confusing as they already mean something else.)

Choosing R and S is very important, this is the best choice we found:

• R = −x 2 and
• S = 2x e x 2

So let's go:

Put in R = −x 2 and S = 2x e x 2

And also R' = −2x and ∫ S dx = e x 2

And we get this nice family of curves:

• My presentations

Auth with social network:

Download presentation

We think you have liked this presentation. If you wish to download it, please recommend it to your friends in any social system. Share buttons are a little bit lower. Thank you!

Presentation is loading. Please wait.

First Order Partial Differential Equations

Published by Spencer Greene Modified over 5 years ago

Similar presentations

Presentation on theme: "First Order Partial Differential Equations"— Presentation transcript:

Boyce/DiPrima 9th ed, Ch 2.4: Differences Between Linear and Nonlinear Equations Elementary Differential Equations and Boundary Value Problems, 9th edition,

1 Week 4 Complex numbers: analytic functions 1. Differentiation of complex functions 2. Cauchy’s Integral Theorem.

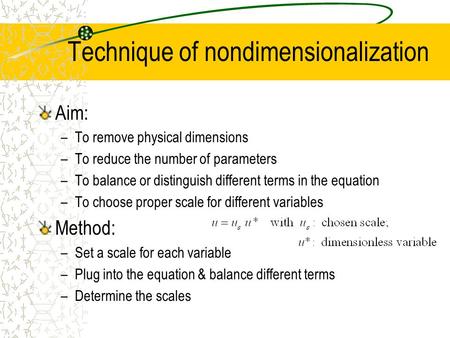
Technique of nondimensionalization Aim: –To remove physical dimensions –To reduce the number of parameters –To balance or distinguish different terms in.

Mathematics1 Mathematics 1 Applied Informatics Štefan BEREŽNÝ.

Equations of Tangent Lines

ESSENTIAL CALCULUS CH11 Partial derivatives

Jeopardy comments/answers April Existence Uniqueness Each kind of differential equation we studied had a corresponding existence and uniqueness.

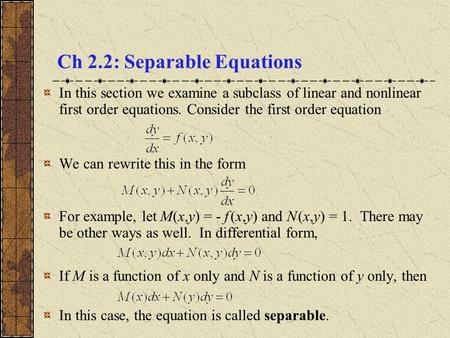
Ch 2.2: Separable Equations In this section we examine a subclass of linear and nonlinear first order equations. Consider the first order equation We can.

1 MECH 221 FLUID MECHANICS (Fall 06/07) Tutorial 6 FLUID KINETMATICS.

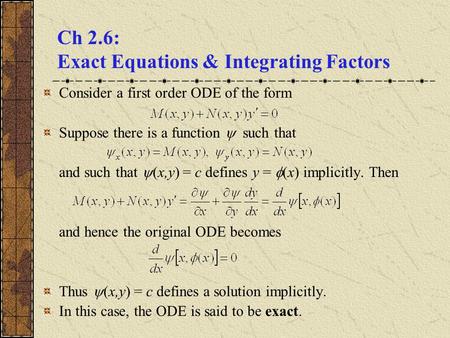
Ch 2.6: Exact Equations & Integrating Factors

Math 3120 Differential Equations with Boundary Value Problems

Ch 2.6: Exact Equations & Integrating Factors Consider a first order ODE of the form Suppose there is a function  such that and such that  (x,y) = c.

Ordinary Differential Equations S.-Y. Leu Sept. 21,28, 2005.

Implicit Differentiation. Objectives Students will be able to Calculate derivative of function defined implicitly. Determine the slope of the tangent.

Revision of Absolute Equations Mathematics Course ONLY (Does not include Ext 1 & 2) By I Porter.

DEPARTMENT OF MATHEMATI CS [ YEAR OF ESTABLISHMENT – 1997 ] DEPARTMENT OF MATHEMATICS, CVRCE.

(MTH 250) Lecture 24 Calculus. Previous Lecture’s Summary Multivariable functions Limits along smooth curves Limits of multivariable functions Continuity.

Mathematics. Session Differential Equations - 1 Session Objectives  Differential Equation  Order and Degree  Solution of a Differential Equation,

About project

© 2024 SlidePlayer.com Inc. All rights reserved.

First Order Partial Differential Equations

Mar 09, 2012

1.49k likes | 3.95k Views

First Order Partial Differential Equations. Method of characteristics. Web Lecture WI2607-2008. H.M. Schuttelaars . Delft Institute of Applied Mathematics. Contents . Linear First Order Partial Differential Equations Derivation of the Characteristic Equation Examples (solved using Maple)

Share Presentation

• partial differential equations
• initial condition
• characteristic curves
• first order
• its tangent vector

Presentation Transcript

First Order Partial Differential Equations Method of characteristics Web Lecture WI2607-2008 H.M. Schuttelaars Delft Institute of Applied Mathematics

Contents • Linear First Order Partial Differential Equations • Derivation of the Characteristic Equation • Examples (solved using Maple) • Quasi-Linear Partial Differential Equations • Nonlinear Partial Differential Equations • Derivation of Characteristic Equations • Example

Contents • Linear First Order Partial Differential Equations • Derivation of the Characteristic Equation • Examples (solved using Maple) After this lecture: • you can recognize a linear first order PDE • you can write down the corresponding characteristic equations • you can parameterize the initial condition and solve the characteristic equation using the initial condition, either analytically or using Maple

First Order Linear Partial Differential Equations Definition of a first order linear PDE:

First Order Linear Partial Differential Equations Definition of a first order linear PDE: This is the directional derivative of u in the direction <a,b>

First Order Linear Partial Differential Equations Example

First Order Linear Partial Differential Equations Plot the direction field:

First Order Linear Partial Differential Equations Plot the direction field: t x

First Order Linear Partial Differential Equations Direction field: Through every point, a curve exists that is tangent to <a,b> everywhere. t x

First Order Linear Partial Differential Equations Direction field: Through every point, a curve exists that is tangent to <a,b> everywhere: 1) Take points (0.5,0.5), (-0.1,0.5) and (0.2,0.01) X X X

First Order Linear Partial Differential Equations Direction field: Through every point, a curve exists that is tangent to <a,b>everywhere: 1) Take points (0.5,0.5), (-0.1,0.5) and (0.2,0.01) 2) Now draw the lines through those points that are tangent to <a,b> for all points on the lines.

First Order Linear Partial Differential Equations Zooming in on the line through (0.5,0.5), tangent to <a,b> for all x en t on the line: Direction field:

First Order Linear Partial Differential Equations Zooming in on the line through (0.5,0.5), tangent to <a,b> for all x en t on the line: Direction field: Parameterize these lines with a parameter s

First Order Linear Partial Differential Equations SHORT INTERMEZZO

First Order Linear Partial Differential Equations SHORT INTERMEZZO Parameterization of a line in 2 dimensions Parameter representation of a circle

First Order Linear Partial Differential Equations Or in 3 dimensions Parameter representation of a helix

First Order Linear Partial Differential Equations Or in 3 dimensions NOW BACK TO THE CHARACTERISTIC BASE CURVES Parameter representation of a helix

First Order Linear Partial Differential Equations • Parameterize these lines with a parameter s: Direction field: s=0.1 s=0.02 s=0 • For example: • s=0: • (x(0),t(0)) = (0.5,0.5) • changing s results in other points on this curve s=0.04 s=0.01

First Order Linear Partial Differential Equations • Parameterize these lines with a parameter s: • Its tangent vector is: Direction field: s=0.1 s=0.02 s=0 s=0.04 s=0.01

First Order Linear Partial Differential Equations • Parameterize these lines with a parameter s, x=x(s), t=t(s). • Its tangent vector is given by • On the curve:

First Order Linear Partial Differential Equations • Its tangent vector is given by • On the curve: IN WORDS: THE PDE REDUCES TO AN ODE ON THE CHARACTERISTIC CURVES

First Order Linear Partial Differential Equations • The PDE reduces to an ODE on the characteristic curves. • The characteristic equations (that define the characteristic curves) read:

First Order Linear Partial Differential Equations • The PDE reduces to an ODE on the characteristic curves. • The characteristic equations (that define the characteristic curves) read: One can solve for x(s) and t(s) without solving for u(s).

First Order Linear Partial Differential Equations • The PDE reduces to an ODE on the characteristic curves. • The characteristic equations (that define the characteristic curves) read: One can solve for x(s) and t(s) without solving for u(s). Gives the characteristic base curves

First Order Linear Partial Differential Equations The equations for the characteristic base were solved to get the base curves in the example: Solving

First Order Linear Partial Differential Equations The equations for the characteristic base were solved to get the base curves in the example: Solving gives

First Order Linear Partial Differential Equations This parameterisation, i.e., was plotted for (0.5,0.5) (x(0),t(0)) = (-0.1,0.5) (0.2,0.01) by varying s!

First Order Linear Partial Differential Equations To solve the original PDE, u(x,t) has to be prescribed at a certain curve C =C (x,t).

First Order Linear Partial Differential Equations To solve the original PDE, u(x,t) has to be prescribed at a certain curve C =C (x,t). The corresponding system of ODE’s has to be solved such that u(x,t) has the prescribed value at this curve C .

First Order Linear Partial Differential Equations The corresponding system of ODE’s has to be solved such that u(x,t) has the prescribed value at this curve C . • As a first step, parameterize the initial curve C with the parameter τ: x=x(τ), t=t(τ) and u=u(τ).

First Order Linear Partial Differential Equations The corresponding system of ODE’s has to be solved such that u(x,t) has the prescribed value at this curve C . • As a first step, parameterize the initial curve C with the parameter τ: x=x(τ), t=t(τ) and u=u(τ). • Next, the family of characteristic curves, determined by the points on C , may be parameterized by x=x(s, τ), t=t(x, τ) and u=u(s, τ), with the initial conditions prescribed for s=0.

First Order Linear Partial Differential Equations • As a first step, parameterize the initial curve C with the parameter τ: x=x(τ), t=t(τ) and u=u(τ). • Next, the family of characteristic curves, determined by the points on C , may be parameterized by x=x(s, τ), t=t(x, τ) and u=u(s, τ), with the initial conditions prescribed for s=0. This gives the solution surface

First Order Linear Partial Differential Equations EXAMPLE 1

First Order Linear Partial Differential Equations • Consider with The corresponding PDE reads:

First Order Linear Partial Differential Equations • Consider with • Parameterize this initial curve with parameter l:

First Order Linear Partial Differential Equations • Consider with • Parameterize this initial curve with parameter l: • Solve the characteristic equations with these initial conditions.

First Order Linear Partial Differential Equations • Consider with • The (parameterized) solution reads:

First Order Linear Partial Differential Equations Visualize the solution for various values of l:

First Order Linear Partial Differential Equations When all values of l and s are considered, we get the solution surface:

First Order Linear Partial Differential Equations EXAMPLE 2

First Order Linear Partial Differential Equations PDE: Initial condition:

First Order Linear Partial Differential Equations PDE: Initial condition: Char eqns:

First Order Linear Partial Differential Equations PDE: Initial condition: Char eqns: Parameterised initial condition:

First Order Linear Partial Differential Equations Char eqns: Parameterized initial condition: Parameterized solution:

First Order Linear Partial Differential Equations Char eqns: Initial condition:

• More by User

First Order Linear Differential Equations

First Order Linear Differential Equations. Any equation containing a derivative is called a differential equation. A function which satisfies the equation is called a solution to the differential equation. The order of the differential equation is the order of the highest derivative involved.

3.49k views • 38 slides

PARTIAL DIFFERENTIAL EQUATIONS

PARTIAL DIFFERENTIAL EQUATIONS. Introduction. Given a function u that depends on both x and y , the partial derivatives of u w.r.t. x and y are:.

839 views • 17 slides

Partial Differential Equations

Partial Differential Equations. Introduction, Adam Zornes Discretizations and Iterative Solvers, Chenfang Chen Parallelization, Dr. Danny Thorne. What do You Stand For?. A PDE is a P artial D ifferential E quation This is an equation with derivatives of at least two variables in it.

1.76k views • 78 slides

Chapter 1: First-Order Differential Equations

Chapter 1: First-Order Differential Equations. 1. Sec 1.1: Differential Equations and Mathematical Models. Definition: Differential Equation.

1.11k views • 23 slides

First-Order Differential Equations

CHAPTER 2. First-Order Differential Equations. Contents. 2.1 Solution By Direct Integration 2.2 Separable Variables 2.3 Linear Equations 2.4 Exact Equations 2.5 Solutions by Substitutions. 2.1 Solution By Direct Integration.

997 views • 48 slides

Partial Differential Equations. Chapter 12 Burden and Faires. General 2 nd Order Form. Discriminant. Classification. Conic Sections. Classification. Elliptical PDE. Elliptical PDE. FDM Molecule. ODE Second Order Formula. x i+1. x i. x i-1.

1.05k views • 66 slides

PARTIAL DIFFERENTIAL EQUATIONS. A partial differential equation is a differential equation which involves partial derivatives of one or more dependent variables with respect to one or more independent variables. Consider the first-order partial differential equation (1) In which

1.92k views • 37 slides

Chapter 1: First-Order Differential Equations. Sec 1.4: Separable Equations and Applications. Definition 2.1. A 1 st order De of the form. is said to be separable. 1. 2. 3. 3. Sec 1.2. How to Solve ?. Sec 1.4: Separable Equations and Applications. 1. 2. 3. 4.

432 views • 13 slides

Partial Differential Equations. Paul Heckbert Computer Science Department Carnegie Mellon University. Differential Equation Classes 1. dimension of unknown: ordinary differential equation (ODE) – unknown is a function of one variable, e.g. y(t)

559 views • 12 slides

First Order Linear Differential Equations. Chapter 4 FP2 EdExcel. This is a method used in C4 of the Core Mathematics A level and then in Chapter 4 FP2. When: We can split the variable in order to find a final equation in the form : y=f(x)

395 views • 5 slides

Chapter 1 First-Order Differential Equations

Chapter 1 First-Order Differential Equations. A differential equation defines a relationship between an unknown function and one or more of its derivatives. Applicable to: Chemistry Physics Engineering Medicine Biology. Chapter 1 First-Order Differential Equations.

1.3k views • 37 slides

CHAPTER 2. First-Order Differential Equations. Contents. 2.1 Solution Curves Without a Solution 2.2 Separable Variables 2.3 Linear Equations 2.4 Exact Equations 2.5 Solutions by Substitutions 2.6 A Numerical Methods 2.7 Linear Models 2.8 Nonlinear Model

2.35k views • 155 slides

Partial Differential Equations. Introduction Deng Li Discretization Methods Chunfang Chen, Danny Thorne, Adam Zornes CS521 Feb.,7, 2006. What do You Stand For?. A PDE is a P artial D ifferential E quation This is an equation with derivatives of at least two variables in it.

403 views • 14 slides

Solving First-Order Differential Equations

Solving First-Order Differential Equations. A first-order Diff. Eq. In x and y is separable if it can be written so that all the y-terms are on one side and all the x-terms are on the other. First-Order Differential Equations.

424 views • 9 slides

CHAPTER 2. First-Order Differential Equations. Chapter Contents. 2.1 Solution Curves Without a Solution 2.2 Separable Variables 2.3 Linear Equations 2.4 Exact Equations 2.5 Solutions by Substitutions 2.6 A Numerical Methods 2.7 Linear Models 2.8 Nonlinear Model

3.45k views • 152 slides

Partial Differential Equations. 第三次作業 報告者 : 曾胤棋 指導教授 : 陳正宗老師. 題目 : yu x -xu y =3x u cauchy data:{u(x,0)=x 2 } u(x,o)=x 2

570 views • 6 slides

Chap 1 First-Order Differential Equations

Chap 1 First-Order Differential Equations. 王 俊 鑫 ( Chun-Hsin Wang) 中華大學 資訊工程系 Fall 2002. Outline. Basic Concepts Separable Differential Equations substitution Methods Exact Differential Equations Integrating Factors Linear Differential Equations Bernoulli Equations. Basic Concepts.

657 views • 57 slides

344 views • 13 slides

1.16k views • 80 slides

PARTIAL DIFFERENTIAL EQUATIONS APPLICATION

PARTIAL DIFFERENTIAL EQUATIONS APPLICATION. Name of the faculty: Abdul Rawoof Subject: Mathematics-4 Topic: Application of partial differential equation Branch: B.Tech -2 nd year -1 st semester(CIVIL-2B) Year:2018. Partial Differential Equations. Definition

623 views • 24 slides